Evanescent waves and "phase-matching" argument

Question

I have looked through several Classical ED approaches to introduce evanescent waves, but still can't build the connections.

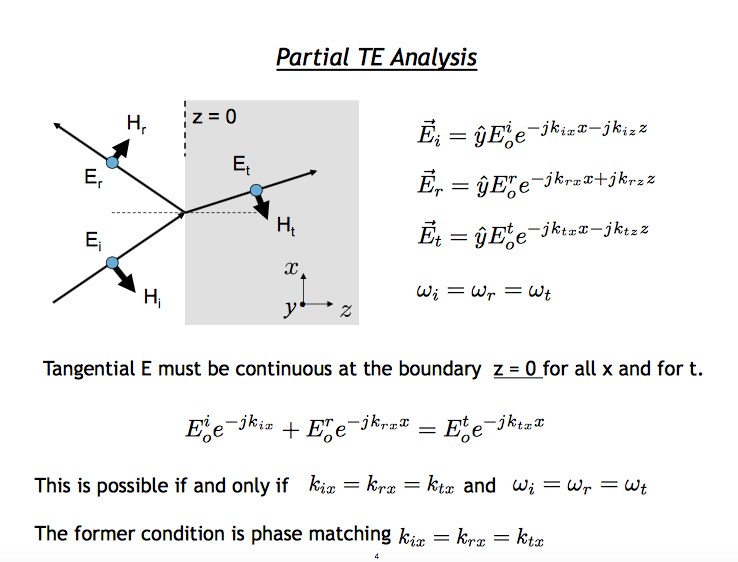
1. I was told that evanescent waves are needed to satisfy boundary conditions on the interface metal-dielectric, see the slide

The argument "if and only if" is not obvious to me. Why is this the only option?

2. Trying to find an alternative way to theoretically "generate" evanescent fields, I came across the statement that phase-matching ensures momentum conservation, which made even less sense relying on (1); however, intuitively it must be true, I just can't see it. I think there might be a cross-matching of terminology in Classical ED and Non-linear Optics here though.

How can I strictly prove the necessary presence of evanescent waves, without making this logical jumps?

Additional comment: I found the argument here, p.113, that since continuity should be met in space and time across the boundary, $k_i^{||}=k_r^{||}=k_t^{||}$ should take place. Otherwise, the boundary conditions can be satisfied instantaneously, but not in time. I like the semantics, but I'd rather see the mathematical prof, if it exists.

Answer 1

This is easy to understand. The condition of continuity of the tangential electric field at $z=0$ has to hold for all $x$ along the $z=0$ interface. This implies that all three x-components of $k$ have to be equal. If this were not the case, you could, e.g., satisfy the condition at $x=0$. Then with different $k_x$ and at an $x \gt 0$ you would get tangential field changed by different factors, which no longer satisfy the boundary condition. Thus you need identical $k_x$ to satisfy the boundary condition for all $x$.