Owen Biesel's paper on 'The Precession of Mercury’s Perihelion'

Question

I have seen this paper was referred to this question How do you calculate the anomalous precession of Mercury? . I am also writing a project paper on Mercury's Perihelion Precession. I will use the calculation for the precession used in this paper. But there are some steps in the paper that i did not understand. I do not have enough reputation to comment on that question.So i am writing down the parts that i did not get. Any help will be appreciated.

(1)(page 9 in the paper)

As you can see there are two terms in the final equation. But when they plugged in the observational values. they only took $2.515*10^7$ but did not use $4.88*10^{-15}$ . WHY?

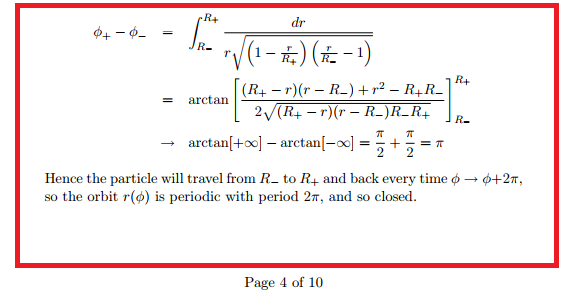
(2)(Page 4) I tried to calculate this integration on Mathematica.But gives different result.

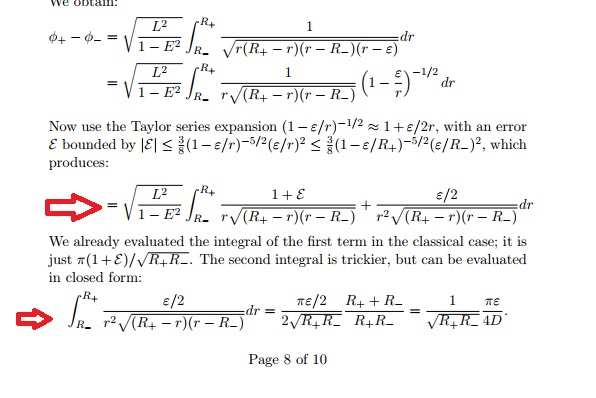
(3)(page 8)I did not get the how the Taylor series expansion led to the equation which is marked in red arrow (1st arrow). also the integration which is marked in red arrow.(2nd arrow).

Answer 1

As far as I can tell from the text, $4.88 \times 10^{-15}$ is the upper bound on the second term in the equation for $\phi_+ - \phi_-$. This is a very small number, meaning the first term alone, $2.515 \times 10^{-7}$, is a good approximation for the equation, and hence the value of $\phi_+ - \phi_-$ as a whole.

In the case of the first arrow, simply replace $1- \left( \frac{\epsilon}{r} \right)^{-1/2}$ with $1+\epsilon/2r$ in the integration.

In the case of the second arrow, integrate the second term between $R_+$ and $R_-$ with respect to $r$.