0
$\begingroup$

I happened to look upon a section of Yakov Perelman's Physics for Entertainment, part I, the author was discussing the relation with distance from centre of earth, and the attraction.

When $d>r_{earth}$, his arguement matches with my intuition, that attraction decreases. The case is baffling when $d < r_{earth}$ - he argues that the weight would be still less than one on the surface, as there would be attraction from all sides.

He writes:

This is because now he earth's attracting forces no longer act just on one side of body, but all around it. (The picture) shows you the weight in the well; it's pulled down by the forces below it and simultaneously up by the forces above it.

And provides this picture:

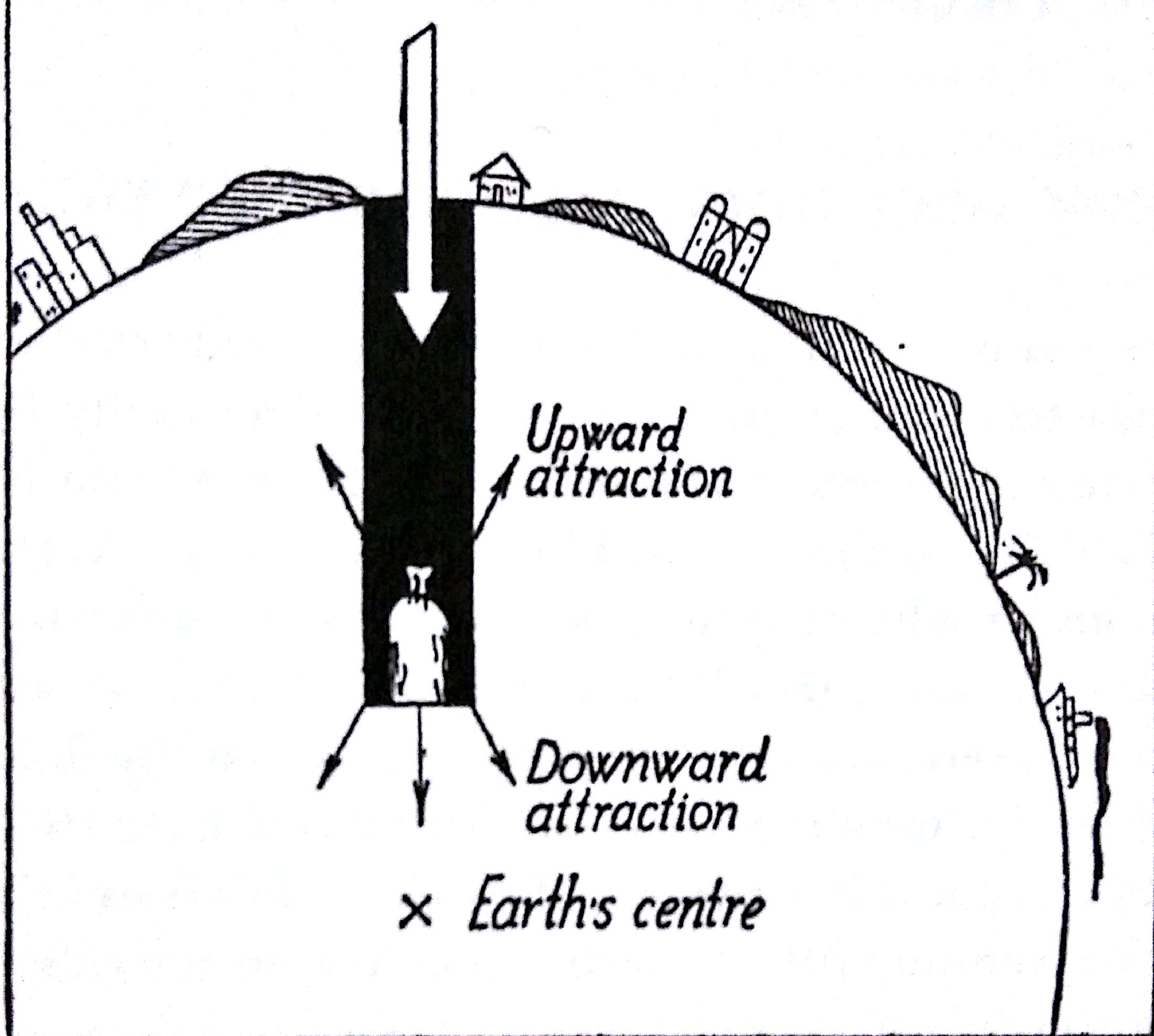
enter image description here

I am not sure about this, (I made some crappy (possibly wrong) thought experiments; and the results were contrary to this); Is Perelman right here ? What is the "Upward attraction"s ?

Improve this question
$\endgroup$
6
  • $\begingroup$ See also 'shell theorem', or Gauss' law. $\endgroup$
    – Jon Custer
    Commented Apr 21, 2017 at 14:44
  • $\begingroup$ Possible duplicates: physics.stackexchange.com/q/18446/2451 , physics.stackexchange.com/q/2481/2451 and links therein. $\endgroup$
    – Qmechanic ♦
    Commented Apr 21, 2017 at 15:01
  • $\begingroup$ See also Earth Science. $\endgroup$
    – gerrit
    Commented Apr 21, 2017 at 15:20
  • 3
    $\begingroup$ Possible duplicate of How does gravity work underground? $\endgroup$
    – David Hammen
    Commented Apr 21, 2017 at 17:18
  • $\begingroup$ As the first answer to the proposed duplicate says, gravitational acceleration inside the Earth at first increases with increasing depth. The key to understanding this is the gradient of gravitational acceleration inside the Earth is given by $\frac {dg}{dz} = 4\pi G \left( \frac 2 3 \bar{\rho}(z) - \rho(z)\right)$, where $z$ is depth, $\bar{\rho}(z)$ is the average density of everything at depths greater than $z$, and $\rho(z)$ is the density of the material at depth $z$. (For a derivation, see physics.stackexchange.com/a/132956/52112.) $\endgroup$
    – David Hammen
    Commented Apr 21, 2017 at 17:25

3 Answers 3

Reset to default
1
$\begingroup$

The 'upward attraction' is gravitation as well. Just imagine the situation in the center of the earth.

Improve this answer
$\endgroup$
2
  • $\begingroup$ There are two contradicting models I am imagining what happens when your centre of mass and the centre of the earth (with zero velocity or acceleration) coincide: One model tells me that no matter how hard some external forces try, as the distance between centre of masses is $0$,you are glued together, you can't be seperated; The other model is telling me arbitrarily small $\epsilon$ force is needed to make you move. I am not sure which model is the correct description of nature. (And this probably have some connection with this problem) $\endgroup$
    – tpk
    Commented Apr 21, 2017 at 14:39
  • $\begingroup$ @tpk in the first, what mass are you 'glued to?' The Earth is an extended object, not a single point mass. $\endgroup$
    – Asher
    Commented Apr 21, 2017 at 15:47
1
$\begingroup$

From your comment on lalala's answer, I think you are trying to apply the formula $$F=G{\frac {Mm}{r^{2}}} $$ to this situation. However, once you are inside the Earth, you cannot use the Earth's mass for $M$. You only include the mass that is closer to the center of the Earth than you are, or all of the mass inside your spherical shell. The way to derive this is to divide the Earth into spherical shells. All shells outside your radius do not contribute, by the shell theorem. All shells inside your radius contribute as you would expect.

The result of this is that as you approach the center of the Earth, $r^2$ decreases, but $M$ decreases faster, and $F$ approaches 0. Since the density is not constant, the force does not decrease monotonically, but varies with decreasing radius. Since the density does not vary within the core, the force monotonically decreases to zero there. (Thanks @davidhammen for correction).

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ Downvoted because F does not smoothly approach zero. It instead increases with increasing depth, then decreases a bit, then increases again, reaching a global maximum at the core-mantle boundary. It's only inside the Earth's core where gravitational acceleration monotonically decreases with increasing depth. $\endgroup$
    – David Hammen
    Commented Apr 21, 2017 at 17:17
  • $\begingroup$ Does that correct the answer? $\endgroup$
    – NoethersOneRing
    Commented Apr 21, 2017 at 19:17
0
$\begingroup$

Well, I am not a physicist, but I believe the general idea could be correct that you would be lighter near to the core of the Earth than you are on the surface of Earth. Weight is a function of mass and gravitational force. On the surface of the Earth, you have the mass of the core and the mass of all other layers of the Earth's surface providing gravitational attraction to pull you down. If you were capable of putting yourself closer to the Earth's core, then you would only have the gravitational force of the core pulling you "down", and you would have the gravitational force of the other layers pulling you "up" (away from the force of the core). Of course, though, gravitational attraction forces are a measure of distance from the center of an object and the masses of the two objects, so the question here must become this: would that change in distance from the core be balanced enough to reduce "Weight."

For that, let us turn to some rudimentary calculations:

First, let us assume the mass of the Earth is roughly 5.9721986×10^24 kg

Now, let us also assume that the inner core accounts for roughly 1.7% of the Earth's mass and everything between the crust (surface) to the edge of the inner core accounts for the other 98.3% of the Earth's mass.

Then, let us assert that the average distance from the center of the Earth (center of inner core) to the surface is 6,371 km = 6,371,000 m.

Let us further declare that there is a person with a mass of 68.04 kg (~150 lb). Based on F = G*(m1 * m2)/r^2, this becomes (6.674×10^(−11) N · (m/kg)^2) * (68.04 kg) * (5.9721986×10^24 kg) / (6371000 m)^2 = 668.1 N (newtons) = 668.1kg*m/s^2

Furthermore, we can state that the radius of the inner core is 1,220 km = 1,220,000 m

Knowing that the inner-core accounts for roughly 1.7% of the Earth's mass, so we'll calculate this to be 1.015273762×10^23 kg.

To determine the change in force, we must calculate the gravitational force acting on the person at the edge of the core: F = G(m1 * m2)/r^2 = (6.674×10^(−11) N · (m/kg)^2) * (68.04 kg) * (1.015273762×10^23 kg) / (1220000 m)^2 = 309.8 N (newtons) i.e., 309.8kg * m/s^2

Thus, the forces acting on the person (before considering "upward" forces) have changed from 668.1 N to 309.8 N, which means there is a decrease in downward force of 358.3 N.

So, we are already going to see a lower weight due to the decrease in downward force and the upward force will further decrease the weight. However, there are some considerable factors left out in this calculation.

We have failed to consider centripetal acceleration and, unless the person is in a vacuum, the forces of atmospheric pressure (further down = more air above the person = higher atmospheric pressure) and convection (caused by the heat of the Earth's inner-core). We have also neglected to consider the height of the individual relative to the distance from the core; however, that is likely negligible here considering that values of (1220000m)^2 vs. (1220001m)^2 are still relatively close to one another.

As previously stated, though, I am not a physicist, and it has been quite a while since I took a physics course, so my calculations could certainly be incorrect. (Someone please correct me if I am wrong.) However, this is my general view on the proposed hypothetical scenario.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.