1
$\begingroup$

Okay so I have this weird problem in my statics book that wouldn't come to a solution no matter what I tried. It states the following:

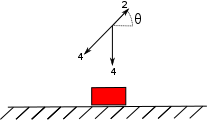
A body of weight $4$ kg.wt is placed on a horizontal rough plane. If a force of magnitude $2$ kg.wt acts on the body with an inclination of $θ$ with the horizontal, or if a force of magnitude $4$ kg.wt acts on the body in the opposite direction of the previous force, the body is about to move. Find $θ$ and the coefficient of static friction.

I have tried to resolve the resultant of the two forces after they partially-cancelled each other, and I've tried to work with the first case alone, since in both cases the body is about to move and $F=μR$. But I could never reach a solution since in all the methods I've tried I always needed the normal reaction ($R$) and I can't get it without the relation $μR$ which equals $2cosθ$, because after resolving the force, the one pointing upwards was ($R+2Sinθ$) and I still had to get $θ$ in order to get mu or anything else. So basically my problem is with getting the theta. All I have so far is that $R=4-2sinθ$.

I have a feeling that I'm doing something wrong. This is my first time studying this topic (friction) in statics, and the problem is a horizontal plane case, which is supposed to be too simple. However, for some reason I'm not finding it simple at all. Is there something wrong with the problem altogether? As in, should they have provided more or other givens? If not, would someone please tell me what I'm supposed to do?

Improve this question
$\endgroup$
3
  • $\begingroup$ Welcome to Physics.SE! I suggest the following: 1) As you receive help, try to give it too, by answering questions in your area of expertise. 2) Take the tour! 3) When you see good questions and answers, vote them up by clicking the gray triangles, because the credibility of the system is based on the reputation gained by users sharing their knowledge. 4) If you get a satisfactory answer, remember to accept it by clicking on the green checkmark. $\endgroup$
    – auden
    Commented Jul 30, 2016 at 21:40
  • $\begingroup$ Keep the two cases separated. I read the question as two different situations that will make the box slide. So that will in the end give you at least two equations - and you have two unknowns, so that should be enough. But now, most importantly, for each case draw a free body diagram and add all forces that act vertically and all that act horizontally. Then add that diagram to the question. This should help a lot $\endgroup$
    – Steeven
    Commented Jul 30, 2016 at 21:41
  • 1
    $\begingroup$ @Steeven Thank you! I showed it to my math teacher and it actually did work. I guess I just misunderstood the problem. :D $\endgroup$
    – Toka
    Commented Aug 10, 2016 at 21:04

1 Answer 1

Reset to default
0
$\begingroup$

Here's how I interpret this question:

enter image description here

We have two equations: The horizontal projection of the 2 kg force equals $\mu$ times the sum of gravity and the vertical projection, i.e. $$2 \cos(\theta) = \mu(4-2\sin(\theta));$$ and similarly for the 4 kg force, except it's projection on the vertical direction is $+\sin(\theta)$, so $$4\cos(\theta) = \mu(4+4\sin(\theta)).$$

Taking a ratio of these two equations (latter over former) gives $2 = \frac{2+2\sin(\theta)}{2-sin(\theta)}$, which can be solved to get $\sin(\theta) = \frac{1}{2}$, i.e. $\theta=30^{\circ}$. Thus $\cos(\theta) = \frac{\sqrt{3}}{2}$, so the first of the original two equations becomes $$\sqrt(3) = 3 \mu \implies \mu = \frac{\sqrt{3}}{3}$$.

Improve this answer
$\endgroup$
0

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.