however, if kinetic friction > applied external force (i.e. without
friction, ofc), then the object is static w.r.t. the observer, and
static friction = applied external force
Kinetic friction can never be greater than the applied force. It can less than the applied force, in which case there will be a net force on the object and the object will accelerate. Once the object is in motion the applied force can be reduced to exactly equal the friction force for a net force of zero and the object will continue moving at constant speed. In general, kinetic friction does not occur until the applied force exceeds the maximum possible static friction force.
However, after 5 seconds the external force applied is ceased, and so
is the friction (since it is a self-adjusting force). Therefore, after
5 seconds, the net acceleration is zero, but at 5 seconds, it still
does retain some velocity.
The kinetic friction force does not immediately cease when the applied force is removed. It is not a self adjusting force. It is generally assumed to remain constant as long as the object is in motion. The self adjusting force is the static friction force which matches the applied force up until the maximum possible static friction force is reached and kinetic friction takes over.
When the applied force is removed, the kinetic friction force now becomes the only external force acting on the object causing it to decelerate until it comes to a stop.
Therefore, after 5 seconds, the net acceleration is zero, but at 5
seconds, it still does retain some velocity.
After the applied force is removed the acceleration becomes negative due to the kinetic friction force that opposes its motion, not zero. It will have an initial velocity equal to that it at at the instant the external force is removed, then decelerate to zero.
Now, Newton's first law of motion states that a body in uniform motion
will retain its state and magnitude of motion until and unless and
unless external force is applied.
That's what the law says, but when the applied force is removed the external force becomes the kinetic friction force alone causing the body to decelerate.
EXAMPLE;
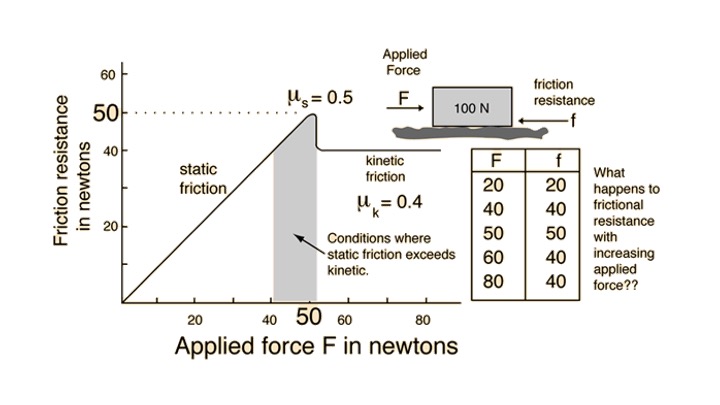
The following example will sum up the above. Refer to the friction plot shown in the figure below taken from the following website, which admits to it being simplistic : http://hyperphysics.phy-astr.gsu.edu/hbase/frict2.html#kin
Let's assume I have a large box of mass $m$ resting on a horizontal surface (floor) with friction. Let the coefficients of static and kinetic friction be $\mu_s$ and $\mu_k$, respectively, where $\mu_{s}\gt \mu_k$ as is generally the case.
I begin to apply a pushing force, but the box does not move because there is now a static friction force equal and opposite to my pushing force. As I gradually increase my pushing force the static friction increases to oppose my force, continuing to prevent the box from moving. We say this tells us that the static friction force is "self-adjusting". In terms of the friction plot, we are on the line where the static friction force exactly equals the applied force.
I continue to increase my applied force until I eventually reach the point where my applied force equals the maximum possible static friction force of $\mu_{s}mg$. If you have ever tried to push something on a floor, at this point you might experience it suddenly "breaking free" with a drop in the resistance to your pushing. In terms of the friction plot this is idealized as a step function (in reality there may be some transition).
Now the friction becomes kinetic equal to $\mu_{k}mg$, which is lower than the maximum static friction force, and which acts opposite to the motion of the box. The friction plot shows the force to be constant implying it is independent of the applied force as well as the velocity of the object. In reality this is only approximately true and only for limited ranges of velocities. For our "textbook" example, we will assume it is.
The box is now in motion. If I continue to apply the force that caused it to move (the maximum static friction force), and the kinetic friction force remains constant, there will now be a net force $F_{net}$ of
$$F_{net}=\mu_{s}mg-\mu_{k}mg\tag{1}$$
From Newtons second law the acceleration of the box will be
$$a=\frac{F_{net}}{m}=\frac{mg(\mu_{s}-u_{k})}{m}=g(\mu_{s}-\mu_{k})\tag{2}$$
Assuming the acceleration remains constant, the velocity of the box $v_{0}$ at some time $t_{0}$ (which could be 5 s as in your example) will be $at_{0}$, or
$$v_{0}=g(\mu_{s}-\mu_{k})t_{0}\tag{3}$$
At time $t_{0}$ I stop pushing the box. The box will continue to slide due to its inertia but its motion is opposed by the kinetic friction force, which is now the only horizontal force acting on the box, giving it a negative acceleration of
$$a=-\mu_{k}g\tag{4}$$
From kinematics the velocity of the box as a function of time is now
$$v(t)=v_{0}-\mu_{k}gt\tag{5}$$
The time it takes the box to stop, i.e., when $v(t)=0$ and kinetic friction ceases is then theoretically
$$t=\frac{v_{0}}{\mu_{k}g}\tag{6}$$
Note that if instead of removing my force at time $t_{0}$ I reduced it to exactly equal the kinetic friction force, the net force would be zero and the box would continue to move at constant velocity of $v_{0}$ per Newton's first law.
Bottom Line; Static friction only exists in opposition to an applied force to prevent the relative motion between contacting surfaces. It matches the applied force with an upper limit. Kinetic friction only exists in opposition to relative motion already underway between contacting surfaces. That motion may or may not be due to an applied force parallel to the surface.
Hope this helps.