Application of Carnot's theorem to calculate efficiency of thermal engine

Question

I understood Carnot's theorem in theory, but I still have some doubt on the practical use of it.

In particular I would like to know: if I have any reversible cycle followed by a gas, then the thermal efficiency of that cycle will be $$\eta=1-\frac{T_{\mathrm{min}}}{T_{\mathrm{max}}}$$

Where $T_{\mathrm{min}}$ is the lowest and $T_{\mathrm{max}}$ the highest among the temperatures of the states involved in the cycle. Is this use of the theorem correct or is there something wrong?

---

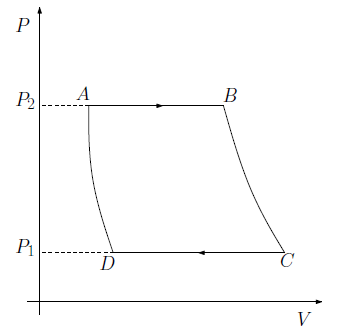
The following example made me doubt about this: consider the reversible cycle in the picture, made of two isobaric processes and two adiabatics.

In this case $T_{\mathrm{min}}=T_D$ and $T_{\mathrm{max}}=T_B$ but the efficiency turns out to be

$$\eta=1-\frac{T_D}{T_A}$$

While, using Carnot's theorem I would have said $$\eta= 1-\frac{T_D}{T_B}$$

---

So I think that my interpretation of the way to use the theorem is wrong. Any suggestion on where my mistake is is highly appreciated.

Answer 1

In particular I would like to know: if I have any reversible cycle followed by a gas, then the thermal efficiency of that cycle will be $$\eta=1-\frac{T_{\mathrm{min}}}{T_{\mathrm{max}}}.$$

As noticed by Wolphram jonny, the sentence above is not true. The Carnot theorem states that any reversible engine operating between two reservoirs has the maximum efficiency given by the equation above. So the only possible reversible cycle between two sources is the Carnot cycle.

It does not mean that you cannot have other reversible cycles. However these cycles would not represent a thermal engine operating between two reservoirs.

For the cycle you drew, there are two possibilities. Either it has two sources and then its efficiency would be less than the Carnot efficiency, which means it is not an irreversible. Or it is reversible which means it has infinite sources. Each source is in thermal equilibrium with a small portion of the cycle.