What prevents this third-order QED scattering from having a non-zero amplitude?

Question

I have learned that in the Dyson-Wick expansion of the QED scattering operator

$$ S=e^{-i\int_{t_i}^{t_f}H\mathrm{d}t} $$

with the QED interaction Lagrangian

$$ H=e\bar\psi\gamma^\mu A_\mu\psi $$

in the limits $t_i\to-\infty$, $t_f\to\infty$, the first-order and third-order terms all vanish. For the first-order terms (single-vertex Feynman diagrams) this seems clear to me, since they correspond to an $e^-e^+$ pair that annihilates into a real photon, which is prevented by energy-momentum conservation at the single vertex.

The same argument holds for many third-order diagrams.

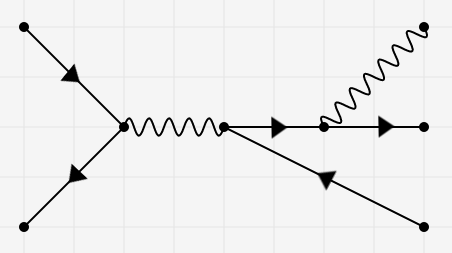
However, there are also three-order diagrams where I cannot see why their amplitudes should vanish, for example this one:

Here, time flows from left to right.

If I understand it correctly, this diagram corresponds to the term (where $\mathcal N$ means the normal ordering "operator"):

$$ \int\int\int dx_1dx_2dx_3 \,\mathcal N\left\{ (\overline\psi^-\gamma^\mu {A_\mu}^-\psi^+)_{x_3}, (\overline\psi^-\gamma^\nu {A_\nu}^+\psi^-)_{x_2}, (\overline\psi^+\gamma^\rho {A_\rho}^-\psi^+)_{x_1}\right\} $$

The process is

• destruction of real $e^+e^-$ pair and creation of virtual photon at the (left-most) point $x_1$.
• destruction of the virtual photon and creation of a virtual electron and a real positron at the (middle) point $x_2$.
• destruction of the virtual electron and creation of an electron-photon pair at the (right-most) point $x_3$.

I don't see why this process should have a zero amplitude. I tried to evaluate it and did not see a delta function that prevents it (like in energy-momentum conservation).

What prevents this process from being physical, i.e. from having a non-zero amplitude?

Answer 1

Not sure where and how you got your magnificent misimpression that your diagram vanishes.

Of course it does not: it is one of the 8 tree diagrams of hard bremsstrahlung, e.g. from S M Swanson, Phys Rev 154 (1967) 1601, all related to each other by suitable crossings and permutations of external momenta. They yield the standard O(α³) cross section for the process.

The amp and its line-perm brethren are physical indeed. An awful amount of actual experimental physics relies on it, and it has an elegant form, cf. section 3 of Berends & Kleiss. There is no good reason it should vanish.