I was hoping that someone who has the book would pipe in here. I don't, but I think I can address some parts of this question.
In perturbative quantum field theory, specifically in particle physics, you may know that we often analyze particle interactions using Feynman diagrams. Each diagram represents some way in which particles can interact, and the sum of all possible diagrams with appropriate coefficients represents the actual behavior of the fields. A generic Feynman diagram might look like this:
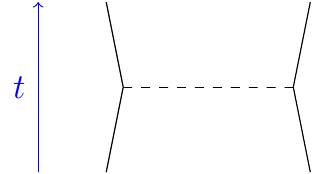
This could represent, say, an electron (left solid line) and a positron (right solid line) exchanging a photon (dotted line).* There are two vertices in this diagram where the lines meet, and each of those could be called an electron-positron-photon vertex because those are the three lines coming into the vertex. (For the one on the left, for example, the electron would come in on the bottom, the photon would come in from the right, and you'd have an electron going out at the top, but if you flip that around it becomes a positron line coming into the vertex.) In the mathematical expression that corresponds to this Feynman diagram, each vertex would be associated with a multiplicative factor which involves the coupling constant $g$, or equivalently, $\sqrt{\alpha}$. There are two vertices, so the diagram as a whole would contain a factor of $g^2 = \alpha$. We would say this diagram is of order $\alpha$.
Now, in particle physics, a particle is really just a (usually localized) disturbance in a field. My guess is that the "kink" Rajaraman is talking about is just some other sort of disturbance in a field. So the same interpretation applies. In short, kinks are effectively particles. Instead of exchanging photons, kinks would exchange something else, presumably mesons. And each vertex which involves two kink lines and a meson line would be associated with a factor
that could include $\frac{1}{\sqrt{\lambda}}$.
The next thing to know is that in quantum field theory, the Born approximation is a way of calculating scattering amplitudes that more or less takes into account only these simple one-exchange diagrams, not any of the more complicated things that can occur in a scattering process. Basically, you find the mathematical expression corresponding to each single-exchange Feynman diagram, add them up, and you get the scattering amplitude. Square the amplitude and multiply by some kinematic factors, and you will get the scattering cross section. It is possible to compute the potential that would give you the same cross section according to classical scattering theory, which is $V(\mathbf{R})$. The calculation is not obvious by any means, but hopefully it should make sense that in some limit, this process of quantum scattering is equivalent to some classical potential, because the quantum theory has to reduce to the classical behavior in the large-distance limit.
*These are not the actual line styles one would use for this process, but that's beside the point

