0
$\begingroup$

I was doing a theoretical research regarding life on Titan. The temperature of Titan is so low, and it needed more sunlight, as a result, the Sun would require to get hotter. My question is, when will the Sun emit 100 times more power than as that of today?

I referred to

this image
(source)

and Stefan-Boltzmann law, $$P= A\varepsilon \sigma T^4,$$ but could not figure out. It may be even impossible that the sun never reaches this stage. I would gladly appreciate any logics, mathematics, or even opinions too.

Improve this question
$\endgroup$
6
  • $\begingroup$ Assume that $R={\rm const}$ and figure out how much hotter the temperature has to be such that $P_\star=100P_{\odot,now}$, that'd give you a first-order approximation. $\endgroup$
    – Kyle Kanos
    Commented Oct 12, 2015 at 13:53
  • $\begingroup$ @KyleKanos Since your comment is after the edit, I have a question: How is this going to help to know when the sun will get 100x more power? $\endgroup$
    – Physicist137
    Commented Oct 12, 2015 at 14:12
  • $\begingroup$ @Physicist137: Yeah, it's not particularly useful on its own. However, it'd give OP a temperature needed for the output which can be used to see what burning cycle is needed. Age estimates for each cycle can be found in abundance elsewhere (online & textbooks). $\endgroup$
    – Kyle Kanos
    Commented Oct 12, 2015 at 14:22
  • $\begingroup$ @KyleKanos: The problem with that idea is that the Sun's temperature never actually rises significantly. The Sun will actually get up to the desired luminosity as a red giant, but by that time its surface temperature has actually gone down; the extra power output is actually more closely related to its larger radius (and surface area) instead. $\endgroup$
    – Michael Seifert
    Commented Oct 12, 2015 at 14:45
  • $\begingroup$ @MichaelSeifert: I know, that's why I posted it as a comment (with the mention of first order approximation, which may even be an over-shoot) and not an answer. OP's best bet would be to do full evolutionary calculations. $\endgroup$
    – Kyle Kanos
    Commented Oct 12, 2015 at 14:58

2 Answers 2

Reset to default
5
$\begingroup$

This is the plot you are looking for. This a solar model taken from the Padova evolutionary tracks by Bertelli et al. (2008). It shows the (log) solar luminosity in terms of the current solar luminosity, as a function of age in years.

From this plot we see that the Sun reaches 100 times its present luminosity at an age of 12.50 Gyr (that is 12.50-4.57=7.43 billion years from now). The Sun is on the first ascent giant branch, burning hydrogen in a shell around its inert helium core.

In practice the figure is not precise to 3 sig figs because it depends to a certain extent on the details of the mass-loss during the giant branch phase and also perhaps more importantly on mixing near the Sun's core, which is still not fully understood.

The luminosity of the Sun versus time

Improve this answer
$\endgroup$
3
  • $\begingroup$ Can you give an estimation of $\frac{d L}{dt}$ at that time? I.e., for instance, how long will it take the Sun to go from $100L_0$ to $101L_0$? It looks pretty steep, but maybe it is only steep on astronomical timescales and Titan will actually have many million years to bask in the warm sunshine before it gets sizzled. $\endgroup$
    – Emilio Pisanty
    Commented Oct 12, 2015 at 16:23
  • 1
    $\begingroup$ @EmilioPisanty According to this model $d \log L/dt \simeq 18.5$ dex/Gyr. To specifically answer your question then, the Sun goes from 100 to 101 solar luminosities in about 230,000 years. $\endgroup$
    – ProfRob
    Commented Oct 12, 2015 at 16:45
  • $\begingroup$ As much as I hate to lose the reputation, this is indeed a better answer. $\endgroup$
    – Michael Seifert
    Commented Oct 13, 2015 at 18:23
3
$\begingroup$

If this site is to be believed, it will only happen for a relatively brief period during the Sun's red giant phase, 7.5 billion years from now (give or take):

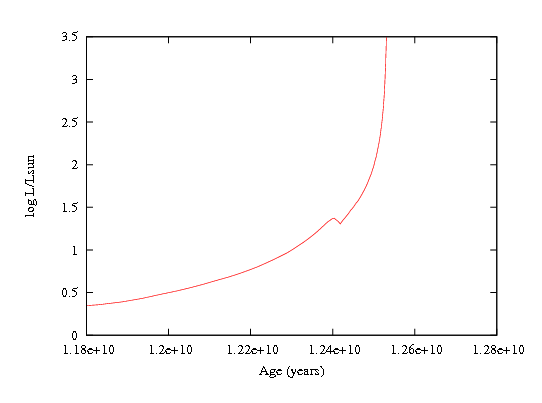
Around the year 7.1 billion AD, the Sun will begin evolving so rapidly that it will cease to be a main-sequence star. Its position on the H-R diagram will begin to shift from where it is now, near the center, towards the upper right where the red giants live. This is because the Sun's helium core will eventually reach a critical point where the pressure from normal gasses cannot hold up the crushing weight being piled on it (not even gasses heated to tens of millions of degrees). A tiny seed of electron-degenerate matter will begin to grow at the center of the Sun. The details of this transition are subject to debate, but theoretical calculations indicate that it will begin when the Sun's inert helium core reaches about 13% of a solar mass, or about 140 Jupiters.

At this point in its life, the Sun will become unruly. ... 500 million years after it hits the critical point, the Sun's luminosity will balloon to 34 $L_0$, fiery enough to create glowing lakes of molten aluminum and copper on the Earth's surface. In only 45 million years more it will reach 105 $L_0$, and 40 million years after that it will leap to an incredible 2,300 $L_0$.

$L_0$ here is the sun's current luminosity (i.e., its total luminous power output). It sounds like the period of time when the Sun's luminosity is between 10 & 1000 times what it is now will only last 100 million years at most. Unfortunately, this would probably not be long enough for Titanean life to evolve into anything interesting (more's the pity.)

Oh, and the dip in the "temperature" graph you posted in the OP is the beginning of the red giant phase. Note that the surface temperature of the Sun is actually cooler during the period in question; but the huge radius of the Sun at this time gives it a much larger surface area $A$ which more than makes up for it.

Improve this answer
$\endgroup$
0

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.