Intuitive explanation of the waves superposition

Question

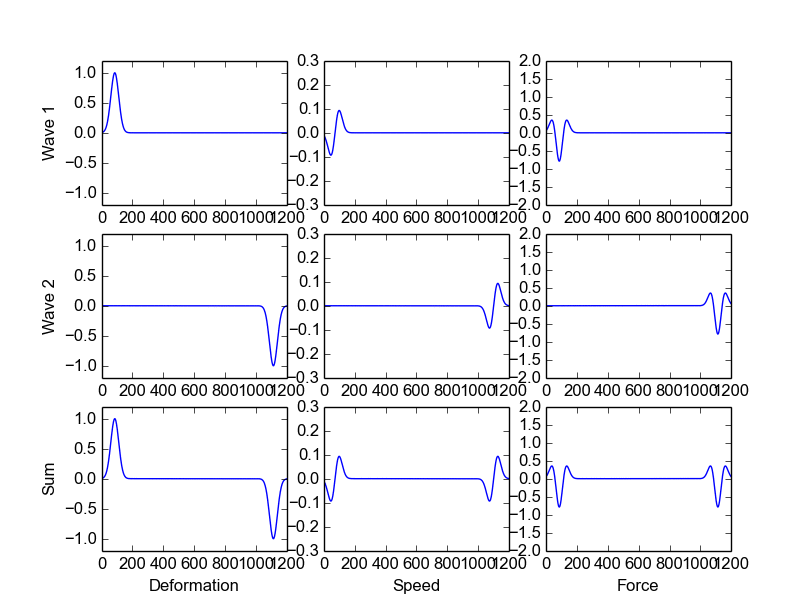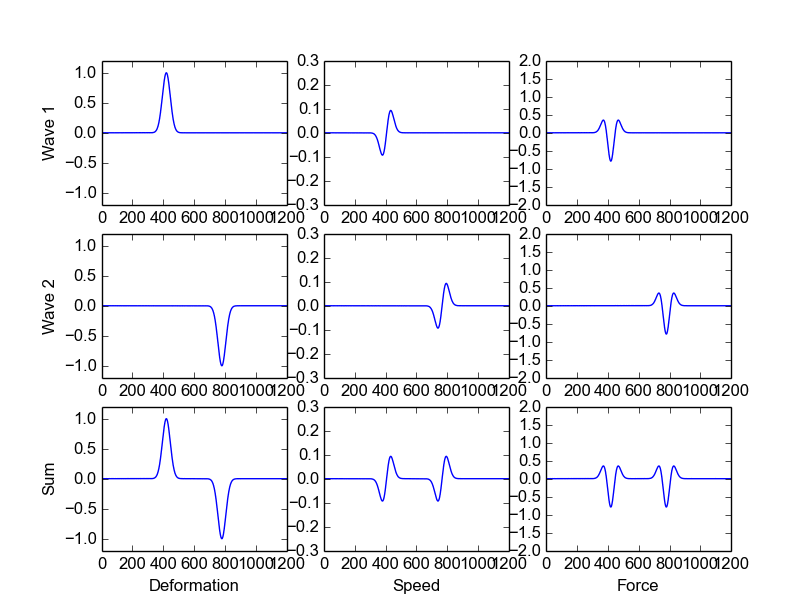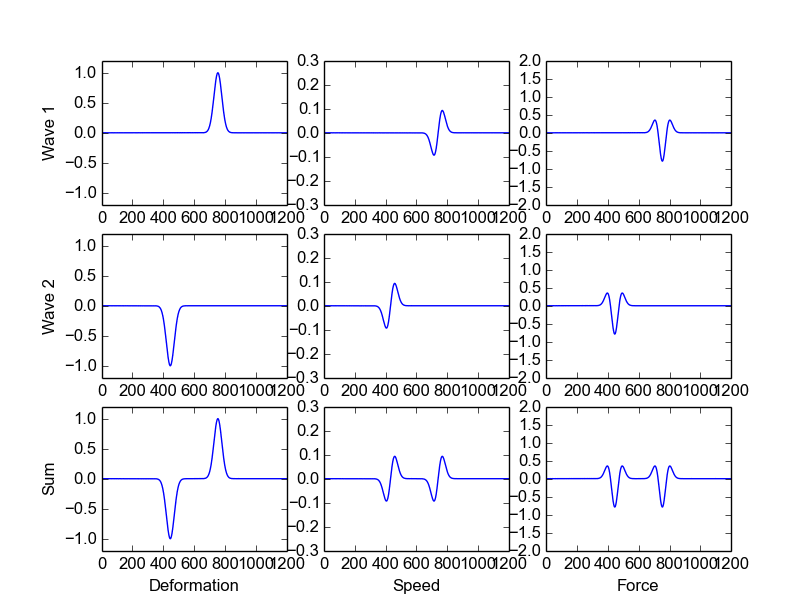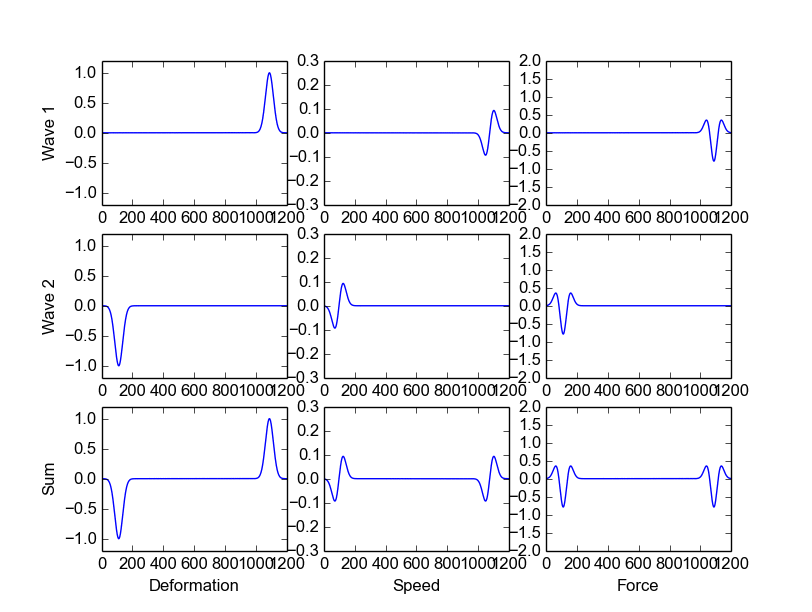
When the two waves collide, why do they pass right through each other?

Mathematically it's due to the principle of superposition: the sum of the two solutions of a wave equation is also a solution. But intuitively it's not clear why the waves would not, say, just cancel each other during the collision.

What would be a convincing 'local' explanation - in terms of the individual particles in the medium (or segments of the medium), that move only due to the interactions with their neighbors?

---

---

Here's a simple example - two colliding wave pulses in the opposite phase with equal amplitudes and wavelengths (animation). In this case explanation is more straightforward (below). How would one make a similar argument for a general case?

In this special case the middle point acts as a fixed point, so that each wave pulse is 'reflected' from a hard boundary. In terms of individual segments: the one closest to the center is drawn to the equilibrium position by the force from the fixed point; it drags the next closest segment with it; etc. When they reach the equilibrium position they continue moving due to inertia and restart the wave in the opposite direction with the opposite phase. The reflected wave has the same amplitude and wavelength due to the symmetry around the equilibrium: the force on each segment at the opposite displacement is a mirror image of the original.

---

EDIT: elaborating on the question

Consider one of the particles on the spring-connected string, like in the bead-spring model (picture). It only interacts with its two neighboring particles - left and right. Now we send two wave packets moving towards each other along the string. For concreteness, let's say we are looking at the particle one to the right from the middle particle (the position where the two wave packets arrive simultaneously and first meet each other). First our particle is moved by its right neighbor due to the wave from the right reaching it. It moves like any other particle in the wave path. But then the particle on its left exerts the force on it, and that one has already been influenced by the wave from the left. Again, all the particle 'knows' is the position of its left and right neighbor. The question is: by just looking at its neighbors, how does our particle 'figure out' to have the displacement that is always equal to the sum of the two constituent wave functions at that point?

Answer 1

When the two waves collide, why do they pass right through each other?

The problem in understanding waves, in my opinion, lies in the fact that one often applies the same concepts he uses in describing particles, to waves. Waves are not particles, and particles are not waves.

While this seems a stupid tautology, it's not that easy to stop mixing the concepts and start thinking in the right framework.

Mathematically it's due to the principle of superposition: the sum of the two solutions of a wave equation is also a solution.

Superposition principle is way more fundamental than you could think. It doesn't just tell you that the sum of two solutions is a solution; it tells you that you can think about each wave independently from the other waves, as if the weren't there. You can picture each wave travelling down the wire, and then sum all the waves that compose your whole waveform.

But intuitively it's not clear why the waves would not, say, just cancel each other during the collision.

Start reasoning in terms of waves. How could a single wave be stopped? Only by dissipation in the medium, or by external forces. Not by means of other waves. That's where the superposition principle plays its role. Different waves (in a linear medium) no not interact. That's it. In your example you see a sort of interaction, but it's actually just a coincidence. It's just visual. You are interpreting the waves as interacting, but actually they are ignoring each other and keeping their behavior unchanged. You could test my statement analyzing the two waves in terms of their momentum/wave-vector instead of their "position".

What would be a convincing 'local' explanation - in terms of the individual particles in the medium (or segments of the medium), that move only due to the interactions with their neighbors?

In waves framework a local explanation would be that the effect of each force acting on a particle is independent of other forces acting on the same particle (or other particles in general). In your example this explains precisely why the center particle doesn't move: it is experiencing equal opposite forces on itself, one coming from the right wave and one coming from the other.

One final remark: the requisite that the effect of each force acting on a particle is independent of other forces acting on the same particle is precisely the superposition principle. It's not just a global property, it's a local one. It must hold in each point of the medium in order to hold globally.

I hope this animation helps you to visualize the importance of superposition.

The top plot shows a wave travelling to the right, the middle one shows a wave travelling to the left, identical but of opposed sign, while the lower figure shows the sum of the two waves.

Link to gif animation

The same but with different amplitudes

Link to second gif animation

---

Spring-mass model

Directly from wikipedia:

The wave equation in the one-dimensional case can be derived from Hooke's Law in the following way: Imagine an array of little weights of mass m interconnected with massless springs of length h . The springs have a spring constant of k:

Here the dependent variable u(x) measures the distance from the equilibrium of the mass situated at x, so that u(x) essentially measures the magnitude of a disturbance (i.e. stress) that is traveling in an elastic material. The forces exerted on the mass m at the location x+h are:

This could be the key point: if you look at $F_{Newton}$ as the effect on the central particle caused by waves passing by, you see need to attribute the cause to $F_{Hooke}$, the elastic force. This effect is precisely linear: how do you tell if the resulting force is caused by a single wave of a certain amplitude, or two waves with different amplitudes that sum to the same amount, or infinite waves that again sum to that total force. You simply can't. There's an infinite number of ways to cause that exact amount of force on the central particle.

Final edit: from the animation it's actually not that clear why the wave shouldn't disappear. It is because you are just looking at the deformation. But it doesn't hold all the information: it's a system evolving in time. You have to also look at speed and force at any instant. This final animation should evaporate all your dilemmas:

You see that when the waves encounter, speeds and forces add, not elide. The elision you see in the deformation domain is just a "coincidence".

Answer 2

When the two waves collide, why do they pass right through each other?

To be sure, if they pass right through each other, they're not colliding, i.e., interacting.

But intuitively it's not clear why the waves would not, say, just cancel each other during the collision.

Keep in mind that each travelling pulse in the animation is essentially a sum of sinusoidal travelling waves that extend over the entire line thus, the components of the travelling waves are passing right through each other at all times and at all places.

What would be a convincing 'local' explanation - in terms of the individual particles in the medium (or segments of the medium), that move only due to the interactions with their neighbors?

If the interactions are not dissipative, the energy of the system is constant. For two waves to destroy each other, rather than pass through each other, the energy associated with the each wave would be converted to... ?