1
$\begingroup$

I was studying mechanical waves today and have questions regarding how a pulse is propagated on a string and its reflection and transmission at a boundary and I hope I can find help here.

First, regarding the propagation of a pulse. Let us assume we have a taut rope and I introduce a pulse in it by whipping one end. When I whip the rope at an end, I apply some force and displace the particles at that end which generates some kinetic energy which must propagate along the rope. I am to understand that each energized particle provides a force for its neighboring particle to displace along the $y$-axis (which is how the energy propagates). What is the force that restores these energized particles back to their initial position? Is it the reaction force according to Newton's third law) from the neighboring particles they just displaced or is it a component of the tension of the string? If it is due to the tension of the string (as I believe it is), what about the reaction force which the neighboring particle should exert? What effect does this reaction force have on the rope? Please give me a clear idea of how this full propagation takes place.

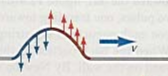
Forces on the particles of a pulse

The blue arrows depicts the restoring force. Now if I have clamped the other end of the rope, the pulse is reflected back inverted. Can you provide an intuitive explanation on how this inversion takes place? I read that the reaction force from the rigid particle causes this inversion, but since the last clamped particle of the rope can not even move, how is the energy due to this force even propagated back and transferred to the adjacent particle which can move? The usual explanation for how a wave is propagated is that when one particle is disturbed (say, moved up), it exerts a pull on another, which in turn exerts a pull on the next one, and so on. In other words, to exert a pull or push on the next particle there must be some movement/disturbance of the previous one. But the particle is clamped.

Improve this question
$\endgroup$
7
  • $\begingroup$ No offence, but two points: 1. I gave up after your 10th question, you could think of splitting this up into a set of questions to improve your chances of getting an answer and 2. Any first year physics book will tell you more than can be detailed here, e.g. Halliday and Resnick or a myriad others, the answers to some of questions are already on the web, e.g. inversion of string on a rope:physicsclassroom.com/class/waves/Lesson-3/Boundary-Behavior $\endgroup$
    – user81619
    Commented Nov 17, 2015 at 13:46
  • $\begingroup$ I visited the link you linked and it doesn't provide a great explanation imo. It just says that the inversion is due to the third law of newton without giving any kind of explanation of how the energy is propagated from a fixed point. To me, article states facts rather than explaining them. I thought of including all my questions in one because I feel they are closely inter related and it would be easier to answer them in a flow. I do not have access to the book you mentioned and the book I am referring to has not given an adequate explanation. $\endgroup$
    – jugalgaladotxyz
    Commented Nov 17, 2015 at 13:52
  • $\begingroup$ @c0smicVorTeX: You are right that physics classroom is quite childish & doesn't explain to the deep. But as count_to_10 said, please split up your questions; don't ask several questions at a time, no matter how closely related they are. $\endgroup$
    – user36790
    Commented Nov 17, 2015 at 13:58
  • $\begingroup$ Okay I shall split the question into 2. $\endgroup$
    – jugalgaladotxyz
    Commented Nov 17, 2015 at 14:02
  • 1
    $\begingroup$ Remove the second part & only keep the first part of how the force or tension interacts. $\endgroup$
    – user36790
    Commented Nov 17, 2015 at 14:02

1 Answer 1

Reset to default
2
$\begingroup$

Try to think the rope made up of small solid balls connected with springs. When you make the bump as shown in your picture the springs are expanded. Now you let go of it. The rising ball applies force upward to the ball on its right. The already expanded springs soon tend to decompress again. In doing so the the falling ball applies a downward force to ball at left. This is how the bump shape moves in the forward direction. The balls at the right keep providing upward force for the forth coming right balls.

When the wave reaches the end which is clamped the right most ball has to remain fixed. All the energy reaches the spring connected with the right most ball. The second last ball will remain high until half of the bump doesn't disappear. Now if there were no clamp the rest half bum had to fall usually. But now it will fall usually + the last spring will decompress very hard and will shake the half bump in downward direction. This will make a reflected bump in the downward direction.

You can similarly conceive the interference of waves on a rope with this model.

Improve this answer
$\endgroup$
5
  • $\begingroup$ So basically it is the tension force that is driving the wave forward and there is no other force acting on the wave ? $\endgroup$
    – jugalgaladotxyz
    Commented Nov 17, 2015 at 15:33
  • $\begingroup$ Well, nothing is really going forward. The bits of string are just going up and down, but in such a way so that the location where these oscillations are happening most moves to the right over time. It can be misleading to think of a wave as an object that you push and pull on, even though waves do carry energy and momentum. $\endgroup$
    – knzhou
    Commented Nov 18, 2015 at 6:44
  • $\begingroup$ @KevinZhou I edited there. I meant the bumped shape moves forward not the particles of string. $\endgroup$
    – user31782
    Commented Nov 18, 2015 at 8:24
  • $\begingroup$ @c0smicVorTeX Yes. In books they usually use the tension force to explain all this stuff. Tension is actually similar to spring forces in this model. You can consider the chemical bonds between consecutive molecules as springs for the sake of simplicity. $\endgroup$
    – user31782
    Commented Nov 18, 2015 at 8:26
  • $\begingroup$ You are welcome. I am not an expert in physics. This is just how I conceptualized waves on strings in my school days. I mean this is just my personal idea -- I don't know if it is 100% right. $\endgroup$
    – user31782
    Commented Nov 18, 2015 at 9:05

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.