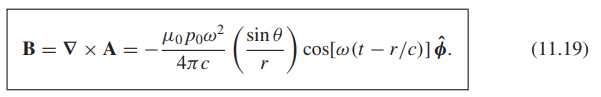I know that there exist plane wave solutions to the Maxwell equations in free space, and I tried solving them for a spherical wave emanating from a point but could find no solution consistent with the 4 equations. This seems to indicate that spherical EM waves are generally prohibited. Why is it then that from small (almost point) sources like light bulbs or from stars we see spherically symmetric EM radiation being released? And why can we apply Huygens principle to EM waves which says that each point on the wave acts as a source of spherical wavelets? Thanks for any help, it's much appreciated.
-
3$\begingroup$ I'm pretty sure spherical waves are possible. You probably miscalculated something $\endgroup$– Níckolas AlvesCommented Jun 1 at 23:26
-
$\begingroup$ How many photons come out of a 2000 lm light bulb? $\endgroup$– JEBCommented Jun 1 at 23:57
-
$\begingroup$ Why does a group of charges with spherical symmetry, where each oscillates radially while retaining spherical symmetry, not radiate? $\endgroup$– mmesser314Commented Jun 2 at 1:36
-
$\begingroup$ The simplest counterexample is a rotating dipole, which radiates in all directions. $\endgroup$– John DotyCommented Jun 3 at 0:43
-
$\begingroup$ I seem to recall an ideal time varying dipole puts out spherical waves. $\endgroup$– R. RomeroCommented Jun 3 at 21:56
5 Answers
You are correct: there are no spherical EM waves. An alternative proof is to appeal to the hairy ball theorem. If we put a spherical surface around a point source, then the electric field at each point on the sphere is tangent to the sphere (since it must be perpendicular to the propagation direction from the source to the point). The hairy ball theorem ensures the electric field is zero somewhere on the sphere. Thus, for the field to have the same amplitude and phase everywhere on the sphere, it must be everywhere zero, always.
The reason we see spherically symmetric light sources in real life is because those sources are incoherent. Your calculation and the above proof only rules out coherent spherical symmetric light sources. That is, a source with a single fixed frequency of emission cannot emit in a spherically symmetric way. But visible light coming off real sources is never of a fixed frequency. On some timescale (the coherence time, roughly $\tau=1/\Delta\nu$ where $\Delta\nu$ is the bandwidth), the phase of the light wave coming off a fixed point on an emitting body will randomly drift. Similarly, on some length scale (the coherence length $c\tau$), the phase of the light emitted by different points on the object varies randomly. Even if the actual EM field at any given instant around an object is never spherically symmetric, the randomness (incoherence) in the light means that when we make measurements with devices that average the light intensity over some large (compared to the coherence length and time) area of the object and time, we get something spherically symmetric. Note that average intensity is a scalar, and thus not subject to the hairy ball theorem.
At radio frequencies, the picture is a bit different. It is easy to directly measure electric fields at this frequency. It is also much easier to make highly coherent radio sources. In the RF world, it is a basic and accepted fact that there is no such thing as an isotropic antenna. There is always at least one dead spot in the emission pattern (e.g. for an ordinary dipole antenna, the dead spot is along the antenna axis). That is what motivates technologies like beamforming, which tries to dynamically change the pattern of emission depending on where the target is to improve reception.
It is common in optics to use the phrase "spherical wave" to refer to pieces of a spherical wave. E.g. if you pass laser light (which can have a long coherence length) through a lens, you can produce spherical wavefronts. These wavefronts are not complete spheres, as forbidden by the hairy ball theorem. Rather, they are only sectors of spheres. Or, in other words, the "coherent point source" that is the image of the laser in the lens does not emit in all directions, which makes its existence possible. The description of the wave as spherical is still useful when all we care about is the field in the region of space that is illuminated by the laser. That is, wavefronts can be locally spherical, just not globally.
-
7$\begingroup$ You wrote "there is no such thing as an omnidirectional antenna", that depends on how you define "omnidirectional". If you do insist that it be vectorial besides omni then the hairy ball theorem applies but if it is about the scalar power density radiation pattern being omnidirectional, a definition that all antenna engineers would use, then "omnidirectional" is possible, see arxiv.org/abs/physics/0312023 and other publications by Matzner. $\endgroup$ Commented Jun 2 at 7:46
-
1$\begingroup$ The hairy ball theorem only applies to polarized sources, and only when you insist that the polarization must be the same in every direction. $\endgroup$ Commented Jun 2 at 21:51
-
3$\begingroup$ @JohnDoty All coherent sources are polarised, and the hairy ball theorem very much doesn't require the polarization to be the same in every direction. That said, the theorem does require the source to be linearly polarised in every direction. $\endgroup$ Commented Jun 2 at 22:15
-
3$\begingroup$ Once you introduce the possibility of elliptical polarization which varies with direction, the hairy ball theorem is no longer applicable, and isotropic coherent radiators (as discussed previously here) become possible. $\endgroup$ Commented Jun 2 at 22:19
-
3$\begingroup$ In the RF world, it is a basic and accepted fact that there is no such thing as an omnidirectional antenna Incorrect. In the RF world, the accepted definition of an omnidirectional antenna is not an ideal source, but a practical one - it only needs to be omnidirectional with respect to a plane (IEEE Std 145-2013 - IEEE Standard for Definitions of Terms for Antennas). True point sources are known as isotropic antenna or isotropic radiators instead. $\endgroup$– 比尔盖子Commented Jun 3 at 11:54
Birkhoff's theorem says that the only spherically symmetric solution of Maxwell's equations is the field of a static point charge, given by the Coulomb potential. However, anything that approximates spherically symmetric radiation to an almost arbitrary degree is permitted (I am writing "almost" because there are physical constraints on the situation, but not mathematical ones). You write it yourself: "almost pointlike sources". Their radiation is not perfectly spherically symmetric.
We must be precise with our definitions.
A spherical wave with spherical symmetry (the E and B fields depends only on r), is impossible, according to Birkhoff's theorem.
However, spherical waves are more naturally defined as waves that emanate spherically outwards. In general, spherical waves are everywhere - you'd expect an arbitrary configuration of charges to radiate waves that travel outward at speed c. However, the cited theorem implies some sort of $\phi$ and $\theta$ dependance in the fields.
The textbook Griffiths provides a few concrete examples. The author starts with a point charge that oscillates up and down, and has a dipole moment $p = p_0 \cos(\omega t)$. He then approximates and solves for the E and B fields far away, and ignores the static fields:
If we then solve for the Poynting vector (a vector representing energy flow), and average over time
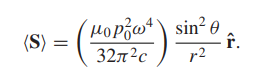
This wave travels outward at speed c, with energy flow decreasing by $1/r^2$, and energy flow pointing radially outwards, exactly as you'd expect. Note that the waves are not spherically symmetric - the fields are smaller near the poles, and the energy flow depends on $\sin^2\theta$.
Now, technically this is not an exact solution to Maxwell's equations due to the approximations, but is very close far away. Luckily, Griffiths actually provides another example of spherical wave that is an exact solution (problem 9.35). It's quite similar - it has the same $\sin^2\theta$ term in Poynting vector.
Griffiths provides a general approximation for an arbitrary configuration (rotating dipoles, other arbitrary motion, etc.). He also shows that in general, any accelerating point charge produces spherical waves that travel outward at speed c.
Regarding the How.
The point charge can only emit spherical EM radiation or monopole radiations if the charge of that point particle changes without any incoming or outgoing current,
Suppose a spherical charge distribution and the charge in it is changing as a function of time, the electric field on an arbitrary shell will change with time and any charge will feel that, a perfect spherical EM radiation.
But this can't happen as it will violate the conservation of charge principle.
Because vast numbers of photons are produced at once from a source such as a xandle in all directions in 3D space, spherical wave forms are made.