Do pear-shaped nuclei really have anything to do with time travel?

Question

Recently (in the last week or two), various articles about pear shaped nuclei have appeared, such as this one from Science Alert and this from the BBC

The Science Alert article includes the quote

We've found these nuclei literally point towards a direction in space. This relates to a direction in time, proving there's a well-defined direction in time and we will always travel from past to present.

and further on,

So what does all of this have to do with time travel? It's a pretty out-there hypothesis, but Scheck says that this uneven distribition of mass and charge causes Barium-144's nucleus to 'point' in a certain direction in spacetime, and this bias could explain why time seems to only want to go from past to present, and not backwards, even if the laws of physics don't care which way it goes.

They've also linked to a copy of the paper on the Arxiv.

The paper's way over my head so I couldn't do much more than scan, but no mention of time travel seems to be mentioned there.

Do these assertions about time travel make sense? And if so, why do they make sense? What does the direction which the nucleus points in have to do with the "direction of time"?

Answer 1

To be honest, much of this feels like very irresponsible journalism, partly on the part of the BBC and very much so on the part of Science alert.

If you're looking for an accessible resource to what the paper does, the cover piece on APS Physics and the phys.org piece are much more sedate and, I think, much more commensurate with what's actually reported in the paper.

The paper itself is very moderate in its claims and it restricts itself very well, from what I can tell, to what they found: that certain radium and barium nuclei appear to be pear-shaped. Finding pear-shaped nuclei is not new (a similar paper (eprint) made the news in 2013, and was discussed on this site here), though Bucher et al. seem to have found hints of a discrepancy with theory with regard to just how pear-like these nuclei look like. This is, however, not at the level of statistical significance that would require any rethinking of the theory at this time.

It is important to note that pear-shaped nuclei are indeed consistent with the Standard Model of particle physics. Pear-shaped nuclei are a bit of a problem because their shape has a direction, that is, you can draw a vector that starts at the flat end and points toward the pointy end (call this the pear vector $\vec P$). (The alternative, a rugby-ball-shaped nucleus, has an axis, but no preferred direction on this axis.) Because of symmetry considerations, this pear vector $\vec P$ needs to be on the same axis as the nucleus' spin $\vec S$, but these symmetries don't tell you which way they have to point, so you get two different versions of the same nucleus:

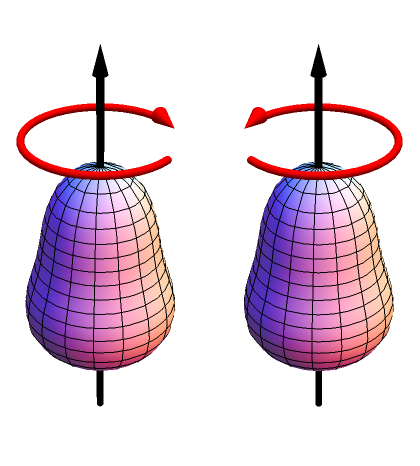
^{Mathematica code for this image through Import["http://goo.gl/NaH6rM"]["https://i.sstatic.net/HLcYp.png"], CC BY-SA with attribution to this page.}

In a theory of nuclear physics that is mirror-symmetric, then both of these nuclei need to be completely equivalent (and, particularly, have the same energy), because they are mirror images of each other. What this paper finds (and what Gaffney et al. found in 2013) is that there are nuclei where this is not true, and these two nuclear states have different energies: the ground state is the "pear" state, and not the "anti-pear" one.

Fortunately, this is not a problem: in fact, we've known since 1956 that nuclear physics is not mirror symmetric, i.e. it is not invariant under the parity operator $P$. Fortunately, though, there is a related symmetry that takes up the slack, and it is charge conjugation symmetry $C$, which takes matter to antimatter and vice versa. Much of the Standard Model, including a lot of nuclear physics experiments, is $CP$ symmetric: if you take a mirror version of the experiment, and on top of that you swap out all particles for their antiparticles, then the physics is the same.

However, $CP$ violations are still compatible with the Standard Model and have already been observed experimentally. On the other hand, the known $CP$ violations are not really enough to explain the matter-antimatter balance in the universe (a.k.a. the baryogenesis puzzle), which is not explained by the Standard Model, so any beyond-the-SM $CP$ violation is a good place to look for solutions to the baryogenesis problem.

There is also a bigger, stronger symmetry, which happens when you combine $CP$ inversion with time inversion $T$ to get what's called the $CPT$ transformation. Because of very basic facts about spacetime, all reasonable physical theories must be $CPT$ symmetric. This is one of the reasons $CP$ violations are so interesting: they point to microscopic violations of time-inversion symmetry.

---

So how does the paper at hand relate to all these generalities? The authors have confirmed the existence of $P$ violations, already observed, and they have found hints that these violations - the peariness of the pear-shaped nucleus are stronger than the existing theory. However, they are not comparing against ab-initio theory (i.e. they compare against approximate theoretical models, so the fault could be in the approximations they made) and, to quote from the paper's discussion,

the large uncertainty on the present result does not allow one to elaborate further.

---

So how did we get from there to time travel? That's where you need a large amount of journalistic 'creativity' for the joins to work. The APS Physics piece is clear and to the point, and it makes a good show of understanding the limitations of the paper.

On the other hand, the press release, from the University of West Scotland, is already pretty breathless. They quote at length from (presumably a direct interview with) Dr. Scheck, but I think his claim that

Further, the protons enrich in the bump of the pear and create a specific charge distribution in the nucleus, which shouldn’t be there according to our currently accepted model of physics.

is stretching a fair bit the pretty modest claims of the paper (cf. supra). Dr. Scheck goes on to claim that

We’ve found these nuclei literally ‘point’ towards a direction in space. This relates to a direction in time, proving there’s a well-defined direction in time and we will always travel from past to present.

but, same as John Rennie, I'm struggling to see how he connects the (known, possibly stronger than accepted) $P$ violation they found to a confirmation of a $T$ violation, let alone relating that to time travel.

Onwards from this, the BBC piece offers very little above the UWS press release, and this is a pretty bad sign - while the BBC does often have great science content, this piece is essentially a redigested press release with the hype turned up one notch, and I can't resist pointing out that things like this have been pointed out as one cause of the bigger problems science has at the moment.

The Science Alert piece (also syndicated at RedOrbit and Business Insider), on the other hand, goes a bit further along on the dodgy journalism side. In particular, it misidentifies the Scheck interview as with the BBC instead of a press release, and it quotes from a couple of stories from last year, but makes it sound like they are reaction quotes from experts interviewed about the recent paper. Other than that, it feels like a re-re-digest piece with the hype turned up three notches.

If you want the hype, then, go to the press release - that's its job. If you want a sober assessment of the implications of the work, go to the APS Physics piece or the phys.org one, which make it clear that there are few implications yet beyond nuclear physics - if indeed the result stands the test of an accurate measurement.

Answer 2

The articles are a little on the hysterical side, but I think they are just saying that violation of CP-symmetry means there must be violation of T-symmetry.

T-symmetry means that physical laws are unchanged if we reverse the direction time flows. Classical theories obey T-symmetry, and it seems intuitively obvious that quantum mechanics would as well. But it doesn't. We've known for a while now that CP-symmetry is violated, and for reasons that are a bit involved that means T-symmetry must also be violated. See for example What sort of experiment would directly test time reversal invariance?.

The claim is that the observation of a non-zero octupole moment for nuclei (i.e. pear shaped) implies that CP-symmetry must be broken and therefore that T-symmetry must be broken, but then we already knew that from other observations so it's not new.

It also isn't clear to me why breaking of T-symmetry rules out time travel. We normally discuss time travel in terms of closed time-like curves (CTCs) i.e. by using general relativity. We have no theory unifying general relativity and quantum mechanics, but there have been many suggestions of ways in which quantum mechanics may prevent CTCs from forming. Some of these may involve violation of T-symmetry, but offhand I don't know of any such arguments.

Answer 3

The popular press's description of this experiment is wildly wrong. It's hard to tell whether they just got it completely wrong on their own, or Scheck got it wrong and they're accurately describing what he said, or if it's some combination of the two. Scheck is a co-author but not the first author, and none of the ridiculous things they represent him as saying are actually in the article.

The observation of a static octupole deformation in a nucleus is unusual and interesting, but has absolutely no implications for CP or T violation per se. For these articles to suggest that it does have such implications is pure nonsense.

The easiest way to see that it's nonsense is to realize that molecules commonly have asymmetric shapes, and this has been known for a century or more. For example, the ammonia molecule has a pyramidal shape, so that you can have a version of the molecule oriented in the +z direction and another version oriented in the -z direction. These two shapes represent two minima in a potential with a tall barrier between them. The probability of tunneling between the two minima is extremely small. The ground state is doubly degenerate, and the degeneracy is split by some very small amount. The reason the splitting is small is not because CP violation is small -- you get the splitting even without CP violation. The reason the splitting is small is because the tunneling probability is small. Because the interaction conserves parity, the two eigenstates of energy are eigenstates of parity.

The physics in the nuclear case is exactly analogous if you really have a permanent octupole deformation, i.e., if the two minima are really separated by a tall enough barrier so that the tunneling probability is small. In most cases that have been observed previously, there was no tall barrier, the tunneling probability was high, and therefore you got a system that behaved somewhat like an asymmetric rotor, but not really. This is not really a permanent octupole deformation. People will refer to these as cases of strong octupole correlations or strong octupole collectivity. In these systems, rather than getting close parity doublets, you get separate negative and positive parity rotational bands, with the positive and negative parity bands being offset in energy relative to one another. One of the signatures of strong octupole correlations is unusually strong electric dipole transitions between the two bands.

Emilio Pisanty gave a nice analysis of a lot of this physics in a previous answer: https://physics.stackexchange.com/a/76340/4552

The only way that this connects to CP or T violation is that it is possible to have an atomic system in which the nucleus has a permanent octupole deformation, and in that situation you can get interactions that effectively amplify the CP violation of the electroweak force. That's cool stuff, but it isn't what was done in this experiment. This powerpoint has a nice explanation.