39
$\begingroup$

In a recent question, Ben Crowell raised an observation which really puzzled me. I obtained a partial answer by looking in the literature, but I would like to know if it's on the right track, and a fuller explanation for it.

It is a well-known fact in atomic and molecular physics that electronic eigenstates of inversion-symmetric molecules never have electronic dipole moments. This is because the electromagnetic hamiltonian that governs molecular physics is parity invariant: under a reflection the eigenstates must map to themselves, but nonzero vector quantities - like dipole moments - must switch signs.

However, it was a fairly big news item earlier this year (see e.g. the University of York press release or the piece in Nature News, 8 May 2013) that atomic nuclei can be 'pear-shaped'. This was predicted in the fifties, such as e.g.

Stability of Pear-Shaped Nuclear Deformations. K. Lee and D. R. Inglis. Phys. Rev. 108 no. 3, pp. 774-778 (1957)

and was experimentally confirmed this year in

Studies of pear-shaped nuclei using accelerated radioactive beams. L. P. Gaffney, P. A. Butler et al. Nature 497, 199–204 (09 May 2013). E-print on L.P. Gaffney's L.U. page.

A pear-shaped nucleus is one that has a nonzero electric octupolar moment. The pear shape arises from the added contributions of quadrupole and octupole perturbations on a spherical shape, winding up with something like this:

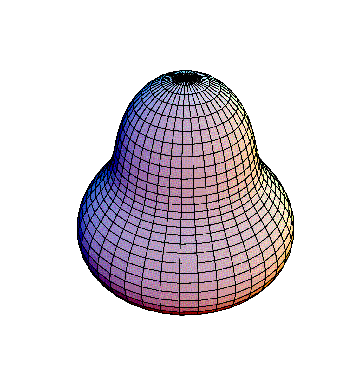
enter image description here

However, this poses an immense problem, because octupole moments have odd parity. If you reflect a pear-shaped nucleus (as opposed to a rugby-ball-shaped quadrupolar one), you get a pear pointing the other way. Having such a nucleus requires a mixing of parity-even and -odd contributions to an energy eigenstate, and this is not allowed for eigenstates of the parity-conserving electromagnetic and strong interactions that (presumably) shape atomic nuclei.

To put this another way, having a pear-shaped nucleus requires a way to tell which way the pear will point. The nuclear angular momentum can break isotropy and provide a special axis, but the 'pear' is a vector (pointing from the base to the stem) and one needs parity-violating machinery to turn a pseudovector angular momentum into a vector quantity.

Another way to phrase this is by saying that if such an eigenstate were possible for a parity-conserving hamiltonian, then the reflected version should also be a degenerate, inseparable eigenstate. Having a unique such ground state means having a way to lift that degeneracy.

I can then pose my question: why are pear-shaped nuclei possible? Is my reasoning incorrect? That is, can parity-conserving hamiltonians lead to such parity-mixed eigenstates? Or are there in fact parity-violating interactions that decisively lift the degeneracies and shape these nuclei? If so, what are they?

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ A nice physics.SE member emailed me the Gaffney paper. The energy levels of 224Ra had been found in 1989 by Poynter et al. In nuclear physics we never see the kind of classic pattern of energy levels seen in an asymmetric rotor in molecular physics: interleaved bands with 0+, 2+, ... and 1-, 3-, ..., with an exact J(J+1) level spacing. In 224Ra the negative-parity band is raised in energy. What's new about Gaffney's work is that they measured absolute electromagnetic transition strengths, which are better probes than energies. The shape is probably unstable rather than statically pear-shaped. $\endgroup$
    – user4552
    Commented Sep 7, 2013 at 20:08
  • 2
    $\begingroup$ I registered to "up vote" the answers given here which cover the fundamentals of this extremely well. However, I don't have enough reputation to contribute yet. I can add a link here to the content of the Nature paper under discussion: ns.ph.liv.ac.uk/~lg/papers/… Unfortunately, there is a 6-month embargo on Nature articles, which restricts the publication of the content elsewhere until this passes. $\endgroup$
    – user39997
    Commented Feb 5, 2014 at 14:28

1 Answer 1

Reset to default
13
$\begingroup$

I have a partial answer to my question, which I'm posting down here because the question was getting too long.

After a good look online at a bunch of confusing (to me) papers from the nuclear physics literature, I came upon this review:

Intrinsic reflection asymmetry in atomic nuclei. P. A. Butler and W. Nazarewicz. Rev. Mod. Phys. 68 no. 2, pp. 349-421 (1996).

Here they draw an analogy to the Jahn-Teller effect, which is originally a molecular physics principle that states that spatially degenerate ground states are in general not possible: there will always be some deformation of the molecule - or some other interaction, however small - that breaks the symmetry of the system and therefore necessarily lowers the energy of at least one of the degenerate states. Thus they explicitly state that

Stable reflection-asymmetric deformations in the body-fixed frame can be attributed to a parity-breaking odd-multipolarity interaction which couples intrinsic states of opposite parity.

Later on, they pinpoint the cause of this parity breaking to the weak interaction:

Parity violation (in the laboratory frame) is caused by the parity-nonconserving component, $V^\text{PNC}$, of the weak interaction. The magnitude of this effect is of the order of $\alpha_p=G_Fm_\pi^2 /G_S\sim10^{-7}$, where $G_F$ is the Fermi constant and $G_S$ is the strong coupling constant.

If this is the case, my natural assumption is that a nucleus whose ground state is pear-shaped must be part of a nearly-degenerate doublet (which comes from the original degenerate ground state of the strong and electromagnetic interactions) separated by about $10^{-7}$ of the gap to the next excited state. The first, 'weakly' excited state would then also be pear-shaped, and would have the projection $$\text{(pear shape vector)}\cdot\text{(angular momentum)}$$ in the opposite direction to the ground state. Is this intuition correct?

Improve this answer
$\endgroup$
4
  • 1
    $\begingroup$ Right. The tunneling from the pear to the parity-inverted pear is very weak - a tunneling effect of a sort - and due to this exponential weakness, it sometimes competes with the small but "power law" violations of parity from the weak interactions. So the latter are able to rotate the lowest-energy eigenstate to a superposition that is highly imbalanced in the pear and inverted-pear. $\endgroup$
    – Luboš Motl
    Commented Sep 5, 2013 at 16:01
  • $\begingroup$ @LubošMotl Cool. I think I do understand most of that. (I'm not quite sure what you mean by 'power law', though.) Do you have a good and relatively simple reference where us atomic physicists can see how this happens? $\endgroup$
    – Emilio Pisanty
    Commented Sep 5, 2013 at 16:11
  • 2
    $\begingroup$ Dear Emilio, unfortunately, I am not able to enumerate good references now (although I may have encountered that sometime in the past), your articles were very helpful for that. By a "power law", I just meant that the matrix elements of the Hamiltonian between pear and anti-pear contributed by the weak force are proportional to a power of the radius of the nucleus - while the tunneling sort of goes like exp(-T) where T is some "thickness of a potential barrier" that some of the nucleons have to go through, and T grows with the nuclear radius. $\endgroup$
    – Luboš Motl
    Commented Sep 5, 2013 at 16:21
  • 2
    $\begingroup$ Although the tunneling is fundamentally due to the strong force which is stronger than the weak force, the exponential suppression may make it weaker than the weak force. A thing to check is that the correction from the weak force is large enough for the pearness to be observable... A similar competition appears for alpha and beta decays. Beta is slow because it's the weak force. Alpha is due to tunneling of alpha-particles which is strong force but it depends on some T exponentially so alpha-decay lifetimes span a huge interval differing by dozens of orders of magnitude. $\endgroup$
    – Luboš Motl
    Commented Sep 5, 2013 at 16:23

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.