Does a AAA battery have a dipole moment?

Question

Does a AAA or D battery have an electric dipole moment? Why don't the opposite poles of two batteries attract each other like that of magnet's?

Answer 1

Not only is the capacitance and charge involved in a AA battery very small (I estimate 25 pF, assuming half-millimeter anode radius and half-centimeter cathode radius), but the inner structure is cylindrical:

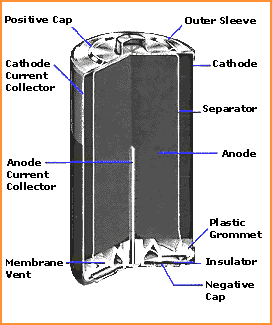
This means that the stored energy in the battery is in an electric field which mostly points radially. If the field were purely radial, the dipole moment would be zero (though there would be quadrupole and higher moments).

Answer 2

Yes, in principle a battery does have an electric dipole moment.

The chemical reaction in a battery moves electrons from the cathode to the anode, leaving the cathode with a net positive charge and the anode with a net negative change. The charge will be given by the capacitance of the battery, $C$:

$$ Q = CV $$

where $V$ is the battery voltage i.e. about 1.5V. The trouble is that I don't know what the capacitance of an AAA or D battery is, but I would guess that it is vanishingly small. That means the charge separation and therefore the electric dipole will also be vanishingly small.

I would be very interested to know of experimental measurements of the dipole field of an open battery. Some Googling suggests the idea has occurred to other people but i wasn't able to find any trace of the experiment having been done.

Answer 3

The other two answers explain conceptually why a cyclindrical battery has an electric dipole moment, but neither of them try to estimate its value quantitatively. Here's my attempt to make a very rough estimate. Warning: if you don't like approximations, then best to stop reading now.

As Rob said, the interior of a battery can be fairly well modeled by concentric cylinders, with a capacitance given (in SI units) by $$C = \frac{2 \pi \epsilon_0 k L}{\ln(b/a)},$$ where $k$ is the dielectric constant of the separator between the cathode and the anode, $L$ is the length of the battery, and $a$ and $b$ are the inner and outer radii of the separator, respectively.

According to https://www.mdpi.com/2313-0105/7/4/64, $k = 3$ is a reasonable dielectric constant for the separator in some battery or other, so let's go with that.

I think that Rob may have misunderstood the definitions of $b/a$, because I don't think it makes any sense to have the anode radius be half a millimeter and the cathode radius be half a centimeter; these should be the radii of their interfaces with the separator, which are extremely close together. According to https://eprints.qut.edu.au/16412/1/Jonathan_Johansen_Thesis.pdf, we can take $a = 4.3$ mm and $b = 4.5$ mm, so $\ln(b/a) = 0.0455$, not $2.30$ as in Rob's estimate. But Wikipedia says that a typical battery separator is only 12-25 microns thick, not the 200 microns suggested by the source linked above. Wikipedia's estimate would decrease the denominator $\ln(b/a)$ by about an order of magnitude. (That source also considers a AA battery rather than the AAA that the OP asked about, but the loss of an "A" will be the very least of our sources of error in this calculation.)

A AA battery is $L = 50$ mm long.

Putting these numbers together, we get a capacitance of 184 picofarads. Using Wikipedia's value for the separator thickness could get that up above a nanofarad. These are actually quite "normal" values for capacitance; you can buy capacitors with these capacitances for literally two cents each. A new 1.5-volt battery will therefore have a net charge of 276 picocolombs on each conductor - about 2 billion excess or missing electrons each.

We can very roughly estimate the battery's electric dipole moment by assuming that each conductor's net charge is concentrated at the center of its terminal. This would get us an electric dipole moment of 13.8 picocoulomb-meters or $4 \times 10^{18}$ Debyes.

As Rob mentioned, this is not a good approximation - in reality, the charge is actually distributed almost perfectly uniformly across the cylinders that form the bulk of the conductors. (Indeed, we implicitly used that more accurate - but incompatible - approximation in calculating the battery's capacitance.) So this figure should be interpreted as a very loose upper bound on the battery's electric dipole moment - I suspect that the true value is at least one order of magnitude smaller. (On the other hand, if we overestimated the separator thickness, then correcting that would make the separated charge, and therefore the dipole moment, larger than our estimate, potentially lessening the overestimate from our charge distribution assumption.) Unfortunately, a more accurate estimate of the electric dipole moment would probably require a quite detailed model of the battery's inner structure.

Since batteries have an electric dipole moment, they should attract each other electrostatically when in the side-by-side, antiparallel arrangement that is most common inside battery-powered devices. The potential energy of two ideal dipoles separated by a distance $r$, both oriented perpendicular to the axis joining them, is (Jackson eq. 4.26) $$W = \frac{{\bf p}_1 \cdot {\bf p}_2}{4 \pi \epsilon_0 r^3},$$ so if they are antiparallel then the force between them is $$F = \frac{3 p_1 p_2}{4 \pi \epsilon_0 r^4}$$ and directed inward. (Note that any error in our estimate of $p$ will get squared, i.e. amplified, in the expression for force.)

For two adjacent AA batteries, $r = 14$ mm, the AA battery diameter.

There are at least three reasons why this expression is not a good approximation for the true force between two real batteries:

1. The multipole expansion is a long-distance expansion, and at separations comparable to the characteristic length scale of the systems (as in this case), all multipole orders will contribute significantly, so the expansion is not particularly useful;
2. Fact #1 is especially true for the batteries considered here, where the cylindrical near-symmetry cancels out most of the dipole moment, so the dipole-dipole interaction probably makes an unusually minor contribution anyway;
3. The charge distribution within each battery is not fixed, but will rearrange itself in response to the presence of the other battery's dipole moment.

But if we just foolishly use the equation above anyway, despite all these caveats, we get that two adjacent batteries electrostatically attract each other with a force of very roughly $1.34 \times 10^{-4}$ Newtons, or the weight of 13.6 milligrams in standard gravity. Depending on the battery's chemistry, this is about $4-9 \times 10^{-4}$ times its weight! That's a whole lot more than I expected. Given all of the approximations, my intuition is that the actual electrostatic attraction force is much smaller than this very rough estimate, but I'm not positive about that. But in any event, the electric dipole-dipole interaction force between batteries is clearly much smaller than the magnetic dipole-dipole interaction force between bar magnets.