How do we know that space expanded faster than a speed of light in inflation?
I have read this Phys.SE question, and it says that limit for faster than a speed of light is for matter and waves only.
I mean isn't edge of universe is determined by something being there like waves or matter?
Cause if there are no waves and no matter - then how does one know that space is there? How can one tell that space is there or not if there is no matter nor waves there with which one could interact, how could one differentiate from space being there and not being there? But then if waves or matter are required to be there for us to know that this is where the edge is, how then universe could expand faster than a speed of light? I must be missing something obvious here, please forgive me I am not a physicist.
-
$\begingroup$ The space between the 'matter and waves' expanded faster than light. This rate of inflation comes from theoretical models - it is not directly observed. $\endgroup$– lemonCommented Sep 20, 2014 at 10:26
-
$\begingroup$ There is no edge to the universe - this is actually addressed by several of the answers to the question you link. The Big Bang wasn't like an explosion that happened at a point, with the universe expanding outwards from that point. The theory used to describe the expanding universe (the FLRW metric) assumes that the universe is the same everywhere. This means it can't have an edge otherwise the points in spacetime near the edge would be different to the rest of the universe. The requirement for homogeneity means the universe is either infinite or it's closed - either way it has no edge. $\endgroup$– John RennieCommented Sep 20, 2014 at 10:47
-
3$\begingroup$ @JohnRennie: So we only allow questions which prove that the OP has all the right concepts already? In that case none of us could post any question, because it's pretty clear that all of our current physical theories are incorrect and probably amusingly nonsensical from the viewpoint of our extraterrestrial physicist friends who have found the correct TOE some six billion year ago. In other words: where do we set the cutoff for non-experts? $\endgroup$– CuriousOneCommented Sep 20, 2014 at 10:54
-
$\begingroup$ @JohnRennie so if universe was same all along during big bang what was the inflation? Not trying to be smart-ass but something has expanded, what was it? Or there was no singularity and universe popped in at big size and that popping in with lots of energy is what we call inflation? $\endgroup$– Matas VaitkeviciusCommented Sep 20, 2014 at 11:04
-
3$\begingroup$ I think this wasn't stated explicitly enough in any of the answers - the universe did not expand at the speed of light during inflation. This is a recurring misconception. You can always find two points which formally recede at $c$ but closer points do not. But this is true for an expanding universe at any time, even now. The only time where you cannot find closer points not to recede at $>c$ is the single one instant of the Big Bang, because there the distance grows infinitely for every two points. But we do not trust the theory at that point. $\endgroup$– VoidCommented Sep 20, 2014 at 12:18
2 Answers
How do we know that space expanded faster than a speed of light in inflation?
Let us start from the beginning, on the reason that the Big Bang theory was proposed as a model for the universe. The reason was the observations that all clusters of galaxies were receding from each other. This is what happens from an explosion at the center, in three dimensions. Within the framework of General Relativity it was proposed that the universe started from one singularity, and a particular solution was proposed and modeled the data up to a point, called the Big Bang.
Inflation came in to try and explain other observations, in fact the cosmic microwave background (CMB) measurements . They show a very homogeneous universe, because even though the maps show hotter and colder regions, the differences are smaller than 0.0002 Kelvin . In the old Big Bang model homogeneity could not be attributed to the universe reaching a thermodynamic equilibrium during the creation of nuclei, because by then the universe was too large for all of it to be able to communicate thermodynamically with velocity of light interactions and homogenize the temperatures.
Thus inflation was imposed for the very beginning after the hypothesized singularity, a time where quantum dynamical interactions homogenized the universe to create the levels of homogeneity obsered in the CMB.
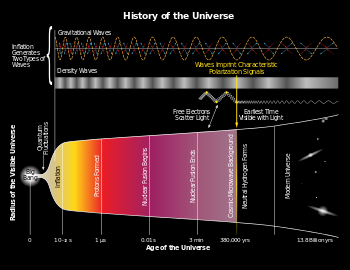
Current BB model
Cause if there are no waves and no matter - then how does one know that space is there? How can one tell that space is there or not if there is no matter nor waves there with which one could interact, how could one differentiate from space being there and not being there?
You are correct in this, that is why one talks of the "observable universe" The models fit the observable universe.
But then if waves or matter are required to be there for us to know that this is where the edge is, how then universe could expand faster than a speed of light?
Models can be extrapolated to currently unobservable parts . We observe their imprint in the CMB, (370000 years after the BB) and maybe, if BICEP2 results hold, in the imprint of the gravitational waves on the CMB (10^-32 seconds after the BB), and using the equations of the current BB model we know that now a part of the then universe is inaccessible to us because the equations tell us that it has/is receded/ing with velocities larger than the velocity of light. See the answer by CuriousOne on this.
-
$\begingroup$ Hello Anna V I don't think it's correct in meaningful way that our equations tell us there's an infinite expansion out there. Equations might say that, but the assumption is built in somewhere. They're not robust, but $\endgroup$ Commented May 7, 2015 at 13:05
-
$\begingroup$ @LucyMeadow define infinite. Our formulae are not infinite. They fit the observable and we extrapolate to the unobservable. The human brain does this every time we catch a ball thrown at us, fitting the observable. We have mathematics as a tool.The gravitational formulae are fitted in the earth but hold also for the back side of the moon, which is unobservable. $\endgroup$– anna vCommented May 7, 2015 at 13:11
Inflation does not violate any local speed of light physics and there is no global prohibition in general relativity against spacetime points that are moving away from each other faster than the speed of light. Such spacetime points are simply not causally connected, i.e. there is no physical way to communicate between them (since light signals from one can not reach the other point). What this means is that we can not look "beyond" the event horizon of the local universe. We don't know what is "outside" of this event horizon. If inflation is correct, the universe may be much, much larger than the part that we can see.
Or maybe the inflation hypothesis is not correct, and something else happened that has similar consequences for the homogeneity as inflation. What could that be?
For instance, the universe could have existed before T_cosmological=0, and it may have been in a well mixed state (i.e. homogeneous) well before T_cosmological even began. This happens in "big bounce" models.
Or the speed of light could be vastly faster at ultrahigh energies, which would allow the initial universe to homogenize much quicker than what we calculate using general relativity and quantum mechanics.
Or general relativity needs a modification, like by adding torsion leading to what's called Einstein-Cartan theory. If I understand correctly, in that model the universe was never arbitrarily small, the big bang starts with a finite size, which also seems to help to make it more homogeneous.
I am sure the theorists here will have some arguments why all of my pet models have already been ruled out (in which case I apologize for talking nonsense!).
The important takeaway for everybody (physicists and non-physicists alike) is that it's important to keep an open mind about several possibilities.
-
$\begingroup$ I figured it might be helpful to add that the torsion-based cosmological model is, at arxiv.org/abs/2006.07748, described as eternal to the past as well as to the future, with each "big bang" being spatially "local", and, consequently, causally separated from others in a multiverse, with their causal separations definitively allowing unverifiable variations in the speed of light. Nikodem Poplawski has described it in 2010-2020 papers whose preprints can be found by his name at Cornell University's "Arxiv" site. It's falsifiable if there's no preferred direction of motion. $\endgroup$– EdouardCommented Feb 21, 2021 at 22:58
