Isn't the Coulomb interaction a photon interaction between two charges?

Question

Isn't the Coulomb interaction a photon interaction between two charges? if yes then what does the following text mean? (Many-particle Physics by Gerald D. Mahan.)

Answer 1

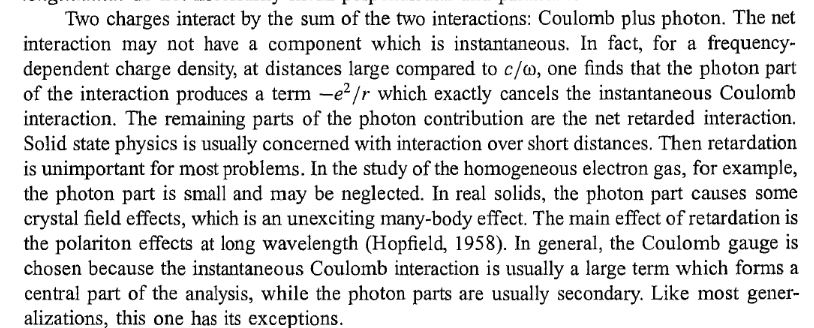
The Coulomb "interaction" appears as the answer to a very specific question, even in QED, that is "what is the correction to the ground state energy of the electromagnetic field if two charges $q_1$ and $q_2$ are pinned at specific locations separated by a distance $r_{12}$?" .

The answer to that question is exactly $\Delta E_0 = \frac{q_1q_2}{4 \pi \epsilon_0 r_{12}}$ and is the Coulomb energy.

Note that there is no time dependence and no $\hbar$ here because A) the problem as it is posed is time invariant and B) quantum effects cancel exactly when the particles are pinned (although they may appear via other mechanism such as vacuum polarisation which is here overlooked or already accounted for via charge renormalization).

If we try to see where does this interaction come from, the explanation tends to vary depending on the gauge that we choose.

Essentially, if you remember the Maxwell-Gauss equation, you know that $\nabla \cdot \vec{E} = \frac{\rho}{\epsilon_0}$. In Fourier space, this gives that $\vec{k}\cdot \vec{E} \propto \rho(\vec{k})$.

If there is no charge in the system, this means that the electric field will only have transverse modes (akin to what happens when we do normal EM field quantization in vacuum). If there are charges however, you will have also longitudinal modes for the electric field. Depending on the gauge, they may appear in the formalism as spin-0 photons.

These spin-0 (or longitudinal) photons cannot be observed in vacuum but obviously do contribute to the build up of the electric field between charges and so do they contribute to the interaction energy between charges and the EM field.

Now, in the general case, particles are moving around and are not pinned at specific locations, in this case, one can still split the contribution of the EM field to the total energy of the system as arising from either longitudinal or transverse polarisations of the electric field. The latter contribution gives rise to photon propagation and retarded effects while the former gives rise to the instantaneous Coulomb interaction in an exact manner.

When the time and length scales involved are of that of atomic systems (what is usually referred to as the low energy scale), the instantaneous Coulomb interaction dominates and we can forget the other terms in the hamiltonian.

Answer 2

Briefly, no.

Charged particle interaction is fundamentally a non-instantaneous photon exchange. The interaction can be written or expanded as the ''naive'' instantaneous interaction ($e^2/r$) plus a photon exchange portion which contains exactly a $-e^2/r$ term and a non-instantaneous (retarded in time) term. The author calls the first term the Coulomb interaction, and he calls the second the photon interaction. (We note that the sum of these two results in the cancelling of the instantaneous $e^2/r$ term, giving us a time-dependent expression for charged particle interaction, as expected.)

Then I think the author says that since distances are small, times are also small, and so the instantaneous Coulomb term dominates. Thus it is acceptable in most cases to use the naive 19th century Coulomb definition.

---

Edit2:

I agree that the author is being very semantically confusing, and even contradictory.

The author is using a loose definition of the word "interaction" (as was I, before this edit). Strictly speaking, the expression "$e^2/r$" does not describe an interaction, but rather just tells us the strength of the force. That is, it doesn't say at all how the force arises, it just describes the end result. (Note the analogy to Newtonian vs Einsteinian gravity.)

Thus you would call it the Coulomb Law; "Coulomb Interaction" is something that does not exist, or has not yet been proposed (since it would be an instantaneous action-at-a-distance mechanism). As far as we know, all charged particle interaction takes place by exchanging photons. (You could call the exchange of photons the "Coulomb Interaction", but then there wouldn't be the distinction that the author makes.)

The author is essentially trying to say that we can ignore the mechanism by which the charged particles interact, and study only the resultant forces and effects arising from their interaction. It would be acceptable, in my opinion, to ignore that somewhat misleading paragraph of the text.