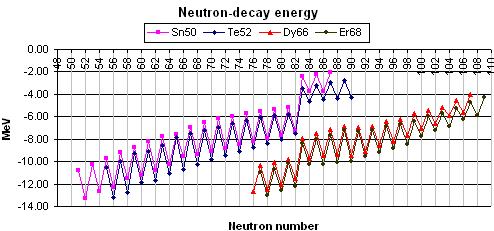
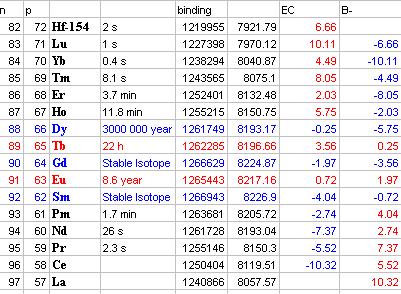
Two nuclides (a type of a nucleus with some values of $Z$ and $N$) that have the same number of neutrons $N$ have the same number of neutrons. The previous sentence is a tautology but I wrote it to show that the equality doesn't mean anything special, aside from the things that are obviously implied by it.
Two nuclides that have the same total number of nucleons - neutrons plus protons - have the same total number of nucleons - neutrons and protons. In the latter case, it also means that the two nuclides are approximately equally heavy and that there may be a beta-decay transformation from one to the other (beta decay doesn't emit any protons or neutrons, so it approximately conserves the mass of the nuclide).
In both cases - equal $N$ or equal $A=Z+N$ - are less important than if two nuclides have the same $Z$. The number of protons $Z$ is by far the most important quantity that determines the character of the resulting material. It's because the electrons only feel the electrostatic force of the nucleus and it only depends on $Z$; it doesn't depend on $N$ or $A=Z+N$. A large chunk of matter has to neutralize the electric charge, so it has to assign the right number of electrons - namely $Z$ electrons for one nucleus.
Consequently, these $Z$ electrons get inevitably arranged into the shells of atomic physics and the properties of the material are therefore a quasi-periodic function of $Z$, the number of protons, as the periodic table makes very clear. That's why the chemical properties of e.g. iodium-127 and iodium-131 are almost identical while iodine-131 and xenon-131 are extremely different chemically, despite their having the same value of $A=N+Z$.
Of course, the mass of the materials depends on $A=Z+N$ and not just $Z$. Also, some isotopes are radioactive while others are not. But radioactivity is not really "chemistry" or "material science"; radioactivity is a rare process that only influences the nuclei while chemistry and material science depends on the electron shells.