One problem is thinking of a photon (or perhaps even waves in general), as if it were a particle:
for a particle we may reason about the time it takes from its emission to reach another point, whereas for a wave we solve wave equation with the boundary conditions (i.e., assuming that the wave is present everywhere). Unfortunately, if your undergraduate studies devoted much more time to classical mechanics than to classical E&M (as is often the case), it is bound to play some evil jokes when trying to understand particle-wave duality.
One could object that the speed of light is finite, and that the photon mode cannot have a finite amplitude immediately everywhere after the device is turned on. Indeed, here we have to describe the propagation of a wave front - but there is nothing particularly quantum about it, that is the same problem would emerge, if we reasoned about a classical Michelson-Morley interferometer. But this latter was never intended to work in such non-stationary conditions - we are observing stationary picture here, averaged over many photons.
This brings us to another important point - observations in "classical" quantum mechanical experiments, at least as far as Copengahenn interpretation is illustrated, are all done for a very large number of particles - i.e., averaged over a quantum ensemble. Same is true, e.g, for a two slit experiment, the image on the screen is not created by a single electron (each of which is detected at a point), but results from detecting many electrons successively passing through slits, one-by-one.
Update
Restating the problem
Rather than considering Michelson interferometer, one could consider a simpler Fabri-Perot one, or even a wave reflected by a mirror. Moreover, the problem in the Q. arises not only for electromagnetic waves, but any other type - elastic waves in beams, waves in a cord, sound waves in an organ tube, etc.: How can we observe interference, if the forward wave arrives to the detector before the reflected wave? The only special factor for EM waves is that we can consider them at very low intensities, when they have to be quantized (although such experiments can be also done for phonons, i.e., elastic waves in clean micro-crystals.)
One-mirror interferometer
Let us for now stick to a single wave reflected by a mirror:
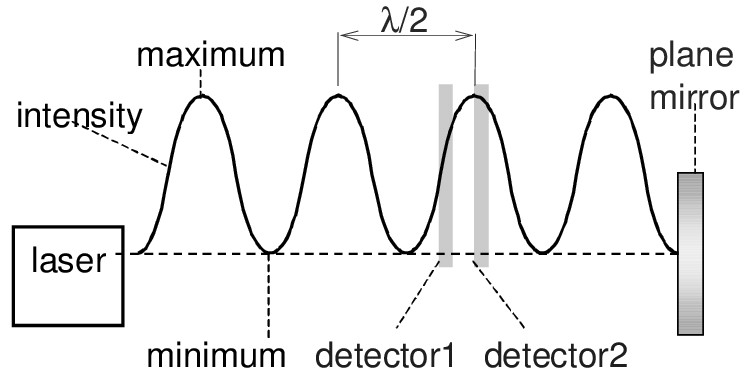
(image source)
The wave is described by a generic wave equation
$$
\frac{\partial^2 u(x,t)}{\partial x^2}-\frac{1}{c^2}\frac{\partial^2 u(x,t)}{\partial t^2}=0,$$
with the boundary condition on the mirror, which I take for simplicity to be $u(0,t)=0$.
The eigenmodes of the interferometer are thus
$$
u_k(x,t)=a_k\sin(kx)e^{-i\omega_k t} + a_k^*\sin(kx)e^{i\omega_k t}
$$
If we wish to work with a quantum field, we perform canonical quantization, replacing $a_k,a_k^*$ by operators and imposing a commutation relation (up to the coefficients that I disregard here):
$$
a_k,a_k^*\longrightarrow \hat{a}_k,\hat{a}_k^\dagger,\\
[\hat{a}_k, \hat{a}_{k'}^\dagger]=\delta_{k,k'}.
$$
(See Canonical quantization, Quantization of EM field.)
Creation of a photon then means adding an excitation to a specific mode of the EM field:
$$
\hat{a}_k^\dagger |\phi\rangle,
$$
where $|\phi\rangle$ is a state in a Fock space. (note that it is not necessarily a vacuum state - some photons might be already present.) But note that the underlying mode structure already exists - the only difference between the quantum and the classical case is how we describe detection (at point $x_0$:
$$
\text{Classical measurement: }I(x_0,t)=|u(x_0,t)|^2,\\
\text{Quantum measurement: }I(x_0,t)=\langle\phi|u^\dagger(x_0,t)u(x_0,t)|\phi\rangle.
$$
In case of a high photon occupation numbers, $\langle \phi|\hat{a}_k^\dagger \hat{a}_k|\phi\rangle\gg 1$ the quantum results approaches the classical one - this is also the regime in which functions classical Michelson-Morley interferometer.
Forward and reflected wave
One thing that was swept under the carpet in the preceeding discussion is that Michelson-Morley interferometer works in a stationary regime - the light was turned on long time ago, and enough time has passed for the wave to propagate from the light source to the mirror and back, and a stable interference patternn has been established. In other words, MM interferometer proves that phase velocity of light is constant - without specifically addressing the group velocity, which could be studied only by observing the transient behavior - i.e., how the interference pattern is established after the light turned off.
One caveat here is the time of propagation vs. the time of emission - no light source emits photons instantly. In fact, the most high-notch quantum experiments are performed on a tabletop, where the emission time of a laser is longer than the propagation time of a photon, and the whole mode structure is "sampled" before the photon is actually "emitted". One needs to sue special techniques to obtain ultrashort pulses, which would allow to model transient behavior of MM interferometer.
If we do assume for the sake of discussion that the emission time is much shorter than the time of light propagation between the mirrors (e.g., because the interferometer is extremely big), then we have to consider a behavior of a wave front, i.e., a superposition of many waves, so that the amplitude increases from zero to a finite value in a narrow space region:
$$
u(x,t)=\sum_k f(k)u_k(x,t).
$$
In the quantum case it means that our initial photon state was created by an operator
$$
\hat{A}^\dagger|vac\rangle=\sum_k f(k)a_k|vac\rangle.
$$
The forward wave then indeed reaches the detector before the reflected waves, as we see in the gig below.
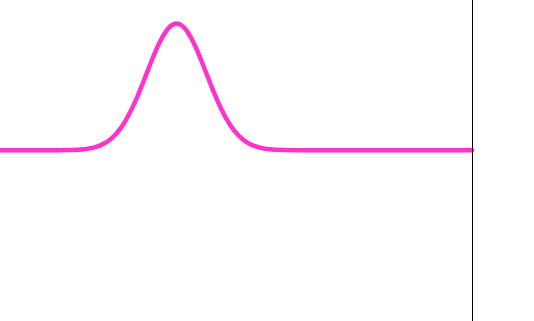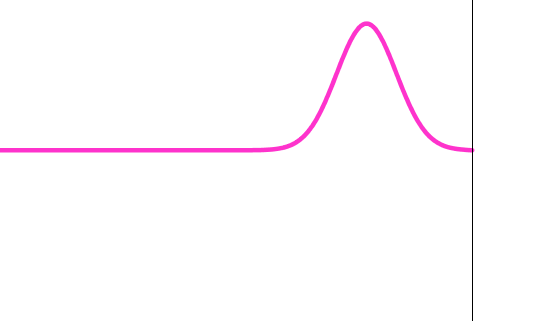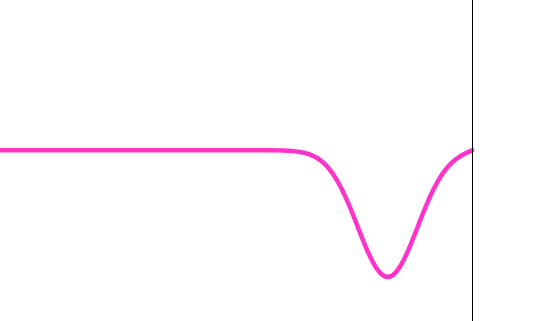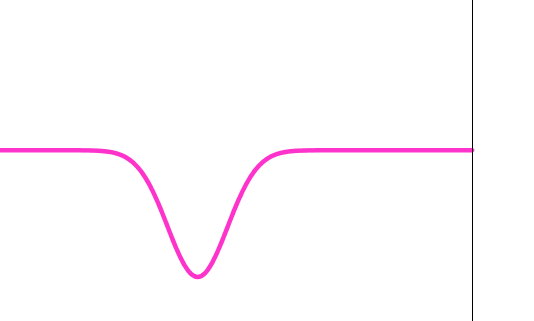
(image source)
The problem with this gif, is that it shows propagation of a wave packet, rather than a wave front, which would look like a step function, rather than a Gaussian - in case of a wave front we could speak of interference between the forward and the backward wave:

As a simple model we can take a hyperbolic tangent:
$$
u(x,t)=A\tanh\left[\omega t - k(L+x)\right] -A\tanh\left[\omega t - k(L-x)\right]
$$
This wave (guessed by the method of images) would not violate the boundary conditions, as $u(0,t)=0$. At time $t=0$ the wave front starts from the point $x=L$. Note that the solution applies only to the region $[-L,0]$, between the light source and the mirror (see the very first image) - we don't care about the values fro $x>0$, which are beyond the mirror.
The position of the front of the forward wave (the first term) is
$$x_{forward}(t) =\frac{\omega}{k}t-L=ct-L,$$
whereas the position of the backward front is
$$x_{back}(t) =L-\frac{\omega}{k}t=L-ct.$$
- The forward front reaches the detector at $x_0$ at time $t_0=(x_0+L)/c$.
- The backward front emerges from after the reflection from the mirror at $t_1=L/c>t_0$
- Finally, the backward front reaches the detector at $t_2=(L-x_0)/c$ (note that $x_0<0$ with the zero of the axis at the mirror). At this point the interference begins, which in the case of this simple wave simply amounts to the cancellation of the forward wave by the reflected one.
Thus, if we measure the intensity at $t < t_1$, we observe only the forward way, whereas at times $t>t_2$ we observe interference.
Quantum case and Copenhagen interpretation
In quantum case the above picture is augmented by treating $A$ as an operator, whose amplitude is described according to the initial state with $n$ photons:
$$
|n\rangle=\left(A^\dagger\right)^n|vac\rangle,
$$
whereas the field operator is now
$$
u^\dagger(x,t)=A^\dagger\left\{\tanh\left[\omega t - k(L+x)\right] -\tanh\left[\omega t - k(L-x)\right]\right\}.
$$
The quantum nature of light then means that during detection we remove photons - at least one photon. Thus, if we detect a photon at times $t<t_1$, we detect only the forward wave, and no interference is observed. If we detect at times $t>t-2$, the interference pattern is measured (cancellation.)
As stated above, in MM experiments the photon occupation numbers are very high, and removing a single photon amounts to negligeable quantum fluctuations. One can however think of an experimental setting similar to the one used in the discussion of the two-slit experiment, where electrons/photons are incident one-by-one. In this case we have a probability of detecting the photon at times $t_1$ and $t_2$. Since these two detection possibilities are mutually exclusive, we have $p_1+p_2=1$. These probabilities, predicted by the matrix element of the field calculated at times $t_1,t_2$ can be deduce by performing many successive measurements, i.e., by averaging over a quantum ensemble - out of $N$ events, only in $p_1N$ of them the photon will be detected at time $t_1$. The ensemble is required, since measurement destroys the photon (as per Copenhagen interpretation.)
Note that measurement at$t_1$ also destroys interference - just like it does in a two-slit experiment, if we try to measure through which experiment an electron passed.
Conclusion
The Copenhagen interpretation survives this test. This is not surprizing - various interpretations of QM are called interpretations, precisely because all of them accommodate experimental observations. If any experiments contradicted this interpretation, it would long ago be relegated to the status of a disproved hypothesis, while the "correct" interpretation would have become a physical theory.



