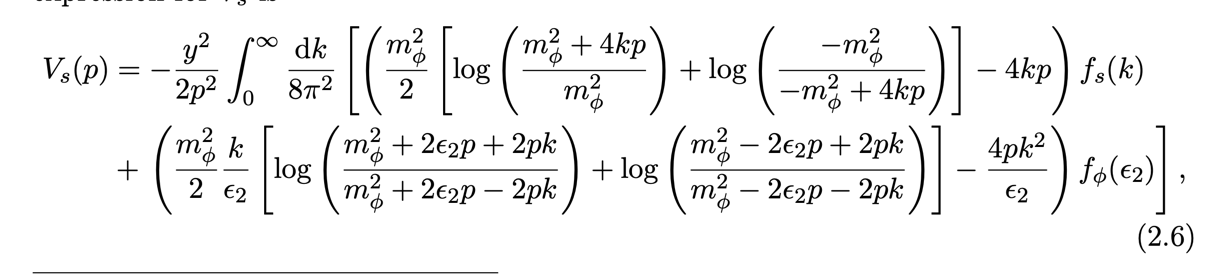I have recently been looking at papers talking about neutrino self-interactions and have seen quite different results among papers. One considers the addition of ${\mathcal{L}} \supset \frac{\lambda_{\phi}}{2} \nu_a \nu_a \phi$ for a scalar mediator $\phi$. They give the cross section $$\sigma(\nu_{a}\nu_{a} \leftrightarrow \nu_{a}\nu_{a})=\frac{\lambda_{\phi}^4s}{128\pi((s-m_{\phi}^2)^2+\frac{m_{\phi}^4\lambda_{\phi}^4}{(32\pi)^2})}$$ with $s=2E_{1}E(1-\cos(\theta))$ and $1$ denoting the target, which gives me $$\Gamma^{\phi}=\int \frac{d^3 p_1}{(2\pi)^3}\sigma(\nu_{a}\nu_{a} \leftrightarrow \nu_{a}\nu_{a}) f_{v_a}=\frac{\lambda_{\phi}^4 T^2}{(8\pi)^3E}\int du \frac{u(u\text{ln}(1+e^{-u})-Li_2(-e^{-u}))}{(u-w)^2+(\frac{w\lambda_{\phi}^2}{32\pi})^2}.$$ Where $\omega=\frac{m_{\phi}^2}{4ET}$ However, in an updated version of the paper, they strangely give a new formula: $$\sigma(\nu_{a}\nu_{a} \leftrightarrow \nu_{a}\nu_{a})=\frac{\lambda_{\phi}^4s}{32\pi((s-m_{\phi}^2)^2+\frac{m_{\phi}^4\lambda_{\phi}^4}{(32\pi)^2})}$$ (factor of 4 difference). $$\Gamma^{\phi}=\int \frac{d^3 p_1}{(2\pi)^3}\sigma(\nu_{a}\nu_{a} \leftrightarrow \nu_{a}\nu_{a}) f_{v_a}v_{Moller}=2\frac{\lambda_{\phi}^4 Tm_{\phi}^2}{(8\pi)^3E^2}\int du \frac{u^2 \text{ln}(1+e^{-\omega u})}{(u-1)^2+(\frac{\lambda_{\phi}^2}{32\pi})^2}$$ with $v_{Moller}=\sqrt{(\vec{v}-\vec{v_1})^2-(\vec{v} \times \vec{v_1})}$ which I compute to be equal to $1-cos(\theta)$. So now I am doubting if either of these are correct.
Similarly, I find another paper which considers a new interaction ${\mathcal{L}} \supset \frac{\lambda_{\phi}}{2} \nu_s \nu_s \phi$ for a fourth neutrino flavor $s$. They give the following expression for the thermal potential:
Which exactly matches the expression given by the previously mentioned paper (with $y$ in place of $\lambda$) despite the fact that $2y=\lambda$. This makes me further question if the expression given above for $\Gamma^{\phi}$ is correct or if the authors are missing a factor related too $\lambda$. I have not yet learned QFT so am unable to derive any of this myself but am hoping someone could lead me in the right direction. None of my results match what is given by these papers and I can't tell if 1) they give different expressions than they used or 2) they are doing the calculations with the expressions they give but doing so incorrectly.