Doubt regarding angular momentum conservation

Question

in the above question I have doubt regarding validity of angular momentum conservation.

The question is solved by conserving angular momentum about axis of larger disc as follows;

$$0 = -\frac{1}{2} M(\frac{R}{2})^2 w + M(Rw')R + \frac{1}{2} M(R^2)w' w'=\frac{w}{12}$$

but as the smaller disc is operated by motor, is angular momentum conserved when motor is turned on

For example: if two similar discs are put one on one, and one of them is operated by motor giving a constant angular velocity $w $, both will move in same direction with $w$. and angular momentum is not conserved

please clear doubt.

Answer 1

The only situations where angular momentum is ''not conserved'' are where your system of interest is coupled to some other system which you aren't explicitly considering so you can take or give angular momentum to this other system (the standard example is some object coupled to the Earth, then when we start to spin we ignore the earth spinning slightly in the opposite direction).

In the situation you're looking at the big disk is ''freely rotating'' so there is no way for the system to transfer angular momentum to anything else.

Answer 2

In classical mechanics, for a fixed pole $H$, the balance equation of angular momentum reads

$$\frac{d}{dt}\boldsymbol{\Gamma}_H = \mathbf{M}_H^{ext} \ ,$$

i.e. time derivative of the angular momentum of a system equals the external (!) moment, i.e. moment exerted on the system by external actions.

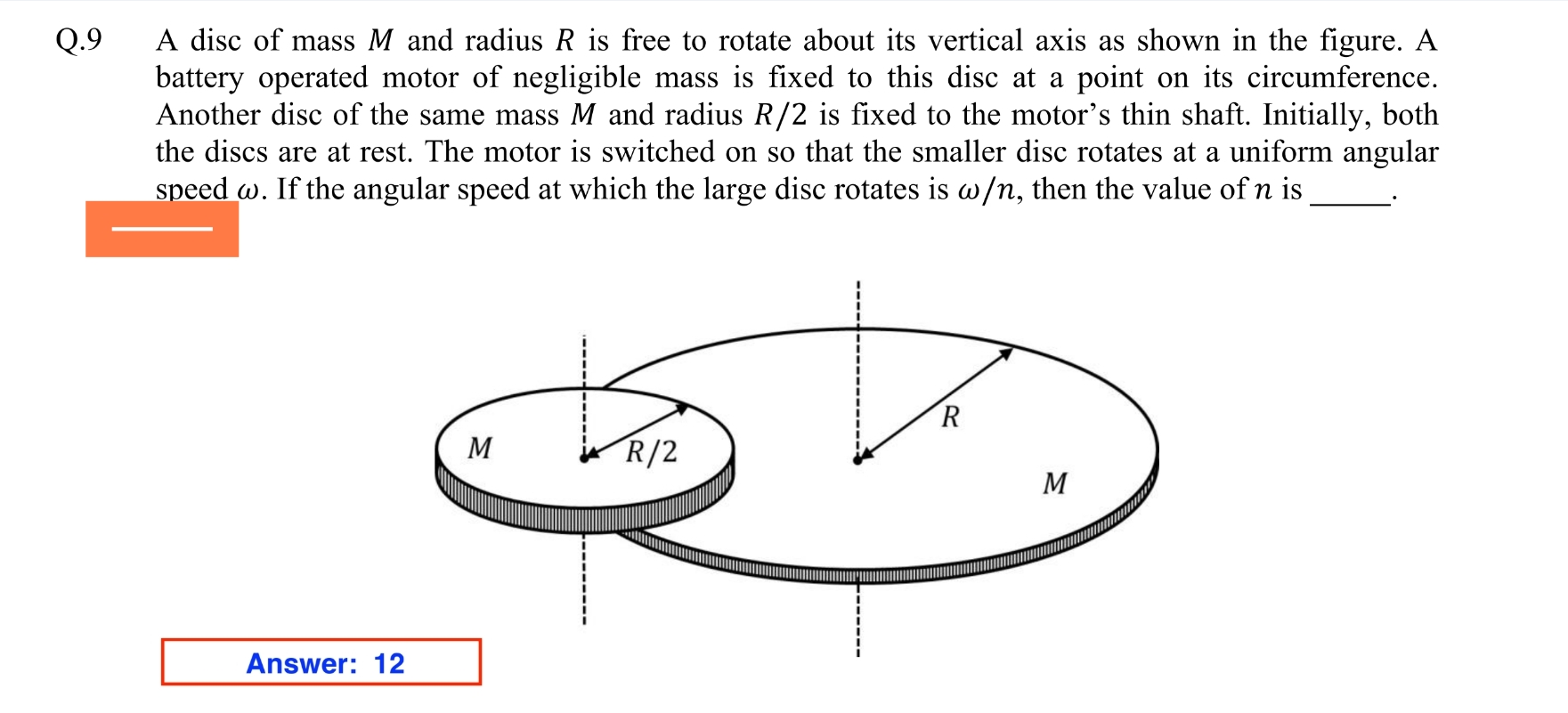
Let's connect the two disks with a motor (not connected anywhere else, so that no constraint exists to provide external actions), and analyze the angular momentum around the axis of rotation of the disks and the motor, called $z$-axis, of different systems and sub-systems:

• whole system disk 1 + disk 2 + motor: the angular momentum of the system is the sum of the angular momentum of its components, $$\Gamma_z = \Gamma_z^1 + \Gamma_z^2 + \Gamma_z^m$$ and no external moment with non-zero $z$-component exist if the cylindrical hinge of disk 1 is a perfect constraint, $M_z^{ext} = 0$ and thus

$$\dot{\Gamma}_z = 0$$

• disk 1: disk 1 has angular momentum $\Gamma_z^1$ and the a torque from motor acts on it $M_{1m}$ and it's equation reads

$$\dot{\Gamma}_z^1 = M_{1m}$$

• disk 2: disk 2 has angular momentum $\Gamma_z^2$ and the a torque from motor acts on it $M_{2m}$ and it's equation reads

$$\dot{\Gamma}_z^2 = M_{2m}$$

• motor: motor has angular momentum $\Gamma^m_z$ and the same and opposite moments it provides to the disks act on it (third principle of dynamics), $M_{m1} = - M_{1m}$, $M_{m2} = - M_{2m}$, i.e.

$$\dot{\Gamma}_z^m = M_{m1} + M_{m2} \ .$$

Summing the equations for the single components, you can easily realize that the equation for the whole system holds, providing the conservation of the $z$-component of the angular momentum of the whole system.

Answer 3

System: small disc, large disc and massless motor.
No external forces and torques act on the system thus angular momentum about an axis is conserved.
The massless motor affixed to the outer rim of the larger disc plays no part in the calculation other than making the small disc rotate from an initial angular speed of zero to a final value of $\omega$.
The change in angular momentum of the small disc about the axis of the small disc is of the small disc is $\frac 12 I_{\rm small,com}\omega- 0$.
For the large disc the change in angular momentum about the same axis as for the small disc is $\frac 12 I_{\rm large,rim}\omega- 0$ and $I_{\rm large,rim}$ can be found by using the parallel axis theorem.
As the net change in angular momentum is zero, $(\frac 12 I_{\rm small,com}\omega- 0) + (\frac 12 I_{\rm large,rim}\omega'- 0) =0$.

For example: if two similar discs are put one on one, and one of them is operated by motor giving a constant angular velocity w, both will move in same direction with w.
System: small disc, large disc and massless motor.
No external forces and torques act on the system thus angular momentum about an axis is conserved.
The given example with the motor being massless and not connected to anything outside the system would result in both discs staying at rest.
If the motor was connected to something outside the system, and hence an external torque was acting on the system, would the discs rotate and the angular momentum of the system would change.