Angular momentum of system or individually?

Question

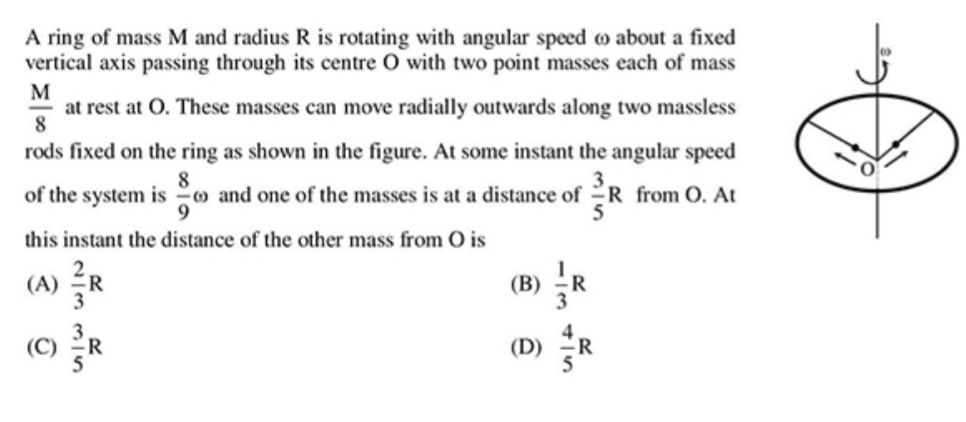
In the solution of the problem below, it was solved using conservation of angular momentum. The equation was $I_1\omega_1=I_2\omega_2$ where $I$ is the moment of inertia of the entire system, which means the deduced equation is $MR^2\omega=\left(MR^2+\frac{M}{8}\left(\frac{3R}{5}\right)^2+\left(\frac{M}{8}\right)x^2\right)\frac{8\omega}{9}$ with which I completely agree.

Here is the second problem: There is a platform of a disc (mass $M$, radius $R$) which is free to rotate about its axis. A person was standing at the circumference of the disc. Initially the system is at rest. Suddenly he started running with velocity $v$. Now we are asked to find $\omega$ of the disc.

Here, when the problem was solved, it was solved by taking the angular momentum of the person separately using $mvr_{\perp}$ formula and taking angular momentum of the disc separately.

My question is: Why can't I use $I_1\omega_1=I_2\omega_2$ in this case? Since $I$ was taken of the entire system in the first problem. So why can't I just take $I$ of the system in this case? Which would obviously give the equation $0=\left(\frac{MR^2}{2}+mR^2\right)\omega$ where $m$ is the mass of the person. Also if we were to calculate angular momentums separately, then why didn't we do the same in the first problem, where we could calculate the angular momentums of both the balls and then the ring?

Answer 1

In your second problem, the person is treated as a particle which is not a material point belonging to the disc rigid body. You can only lump his inertia in with the moment of inertia of the disc if he "rides along with the disc" and acts kinematically like a material point of the disc.

Angular velocity is introduced to relate velocities belonging to the same rigid body. If $A$ and $B$ are two material points belonging to the same rigid body, then the relative velocity is given by ${\bf v}_A - {\bf v}_B = {\bf \omega} \times ({\bf r}_A - {\bf r}_B)$, where ${\bf r}_A - {\bf r}_B$ is the position of material point $A$ relative to material point $B$. This formula does not hold for the person: you cannot relate his velocity to the velocity of some material point on the disc through the disc's angular velocity.

Edit: You may be able to get away with the method you described but only at the initial time instant, when the person's velocity matches that of a material point belonging to the rim of the disc. For that split moment, the person behaves as though he belongs to the disc.

Answer 2

Why can't I use $I_1\omega_1=I_2\omega_2$ in this case? Since $I$ was taken of the entire system in the first problem. So why can't I just take $I$ of the system in this case? Which would obviously give the equation $0=\left(\frac{MR^2}{2}+mR^2\right)\omega$ where $m$ is the mass of the person.

So what are you going to plug in for $\omega$? The man and the disc have different angular velocities. If we consider the man is moving clockwise, the disc is rotating counter-clockwise. Therefore they don't have a common $\omega$.

But in your first question, all bodies have the same angular velocity.

Actually your second equation is not incorrect. It will give you $\omega=0$ which is the angular momentum of the system, as expected. Though you are asked to find the angular velocity of the disc. Therefore you have to consider the components of the system (man and disc) seperatedly.

Answer 3

In both problems, the angular momentum of the entire system is conserved, where the system consists of three and two objects, respectively. The objects within the system can move relative to each other and hence cannot be viewed as a rigid body. Therefore the contribution of each object should be calculated separately.

In the first problem we get $$L = I_{\text{ring}}w_{\text{ring}} + I_1w_2 + I_2w_2$$ where $I_{\text{ring}}$ and $w_{\text{ring}}$ refer to the ring, while $I_1, I_2$ and $w_1,w_2$ refer to the point masses. The catch is that at any instance we have $w_{\text{ring}} = w_1 = w_2$ as point masses are fixed on rods attached to the ring. The other data are $$I_{\text{ring}} = MR^2, \quad I_1(r_1) = \frac{Mr_1^2}8, \quad I_2(r_2) = \frac{Mr_2^2}8$$ where $r_1, r_2$ are distances of the point masses from the center.

In the second problem we get $$L = I_{\text{disk}}w_{\text{disk}} + I_{\text{person}}w_{\text{person}}.$$ Now we don't have $w_{\text{disk}} = w_{\text{person}}$ because the person is not fixed to the disk. We do know that $$I_{\text{disk}} = \frac12MR^2, \quad I_{\text{person}} = mR^2$$ At the beginning we had $w_{\text{disk}} = w_{\text{person}} =0$ and later we have $w_{\text{person}} = \frac{v}{R}$ so we can calculate $w_{\text{disk}}$.