"Now the short arm moves 1/4 the distance, but we get 4x the force"
This description in the video is a bit confusing. They are not talking about the distance the tip of the short transfer arm in the middle moves, because that tip moves the same distance as the tip of the long transfer arm in the middle, because they are in contact. They are talking about the distance the tip of the short outer arm on the far left moves, relative the distance the tip of the long outer arm on the far right moves. The diagram below is a schematic of the situation at timestamp 1:46 in the video:
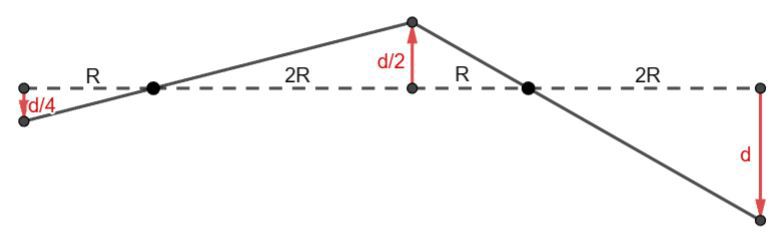
It is now clear the distance they are talking about is the vertical distance the tips of the outer arms move.
An even better replication of a fulcrum lever using gears (or pulleys) is to have both gears on the same shaft as depicted below:
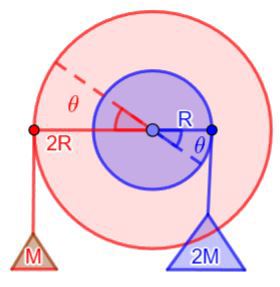
In this set up the mechanical advantage is 2:1 when the larger gear is the input and it is tempting to think that this is due the the larger gear having twice the radius than the smaller gear, but this is not necessarily the case. The mechanical advantage is in fact proportional to the vertical distances the weights move, rather than the ratio of the radii. This concept is known as the "virtual work" theorem of levers. The work is proportional to the force multiplied by the distance it moves through: $$\Delta W = F_{in} \ \Delta d_{in} = F_{out} \Delta d_{out},$$.
where d is the distance the force moves through. It applies universally to gears, levers and pulleys. It is called "virtual work" because it applies even when the forces/gears/levers/pulleys are not actually moving. It is how far the force would move, if the apparatus did move. (You could argue that at a quantum level, nothing is truly stationary.) It also solves the "right angled lever paradox" of relativity and is more fundamental than the traditional law of levers. This is because it is based on conservation of energy and work in equals work out, if losses like friction are ignored.
The distance the force moves through at each end (which is the distance you asked about) is more fundamental and your question raised an important issue.
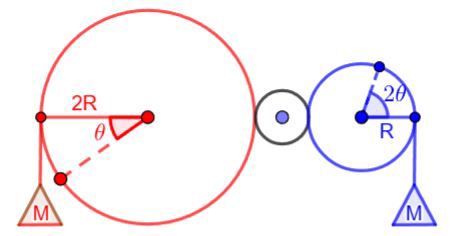
In the above setup the forces are balanced, but the torques are not. It is tempting to think that if the torques are not in balance on either side, then the gears should rotate (as is implied by the traditional law of the lever). This is not the case here and the above system is in fact in equilibrium. This demonstrates the superiority of the 'virtual work' interpretation of levers. The virtual work on the left is $F \times d = Mg \times \theta 2R$ and the virtual work on the right is $F \times d = Mg \times 2\theta R$ and these are equal and so the system is in equilibrium. Force applied to the perimeter of a gear is equal everywhere along that perimeter. Where the teeth mesh, the forces are equal and the force all along the perimeter of the next gear is equal all the way around the perimeter and so on. This shows that the force acting on the perimeter of the right hand gear must be equal to the force acting on the left hand gear, in this case. The force acting on the perimeter is only multiplied, when the gears are on the same axle as shown in the diagram above this one.
Just in case you are curious and to show the usefulness of the virtual work interpretation, here is how it solves the "Right Angled Lever Paradox" of relativity:
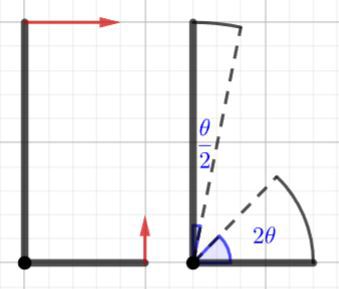
The diagram on the left is a right angled lever, as seen by an observer moving relative to the lever. In its rest frame, the lever has equal length arms with equal forces acting on the ends and is in equilibrium. To an observer moving relative to the lever with a velocity such that the gamma factor is 2, the length of the lever arm parallel to the motion is halved and the force acting on it is also halved according to the laws and transformation of relativity. This means the torque acting on the longer arm is four times the force acting on the shorter arm and according to the traditional lever law the lever should be rotating clockwise in this reference frame, which gives rise to the paradox. In the diagram on the right, the situation is analysed in terms of virtual work. Due to lengths parallel to the motion length contracting, angles transform in an asymmetrical way. The angle the longer arm rotates through is 1/4 of the angle the shorter arm rotates through.
The work done by the longer arm is $W_i = f \times d = F \times \theta/2 \ R = F \theta R/2$.
The work done by the shorter arm is $W_o = f \times d = F/2 \times 2\theta \ R/2 = F \theta R/2$.
Since $W_i = W_o$, the work in is equal to the work out and the lever is not expected to rotate by the virtual work theorem, resolving the paradox.




