0
$\begingroup$

I have enjoyed reading the question "how do levers amplify forces" and related discussion available at How do levers amplify forces?.

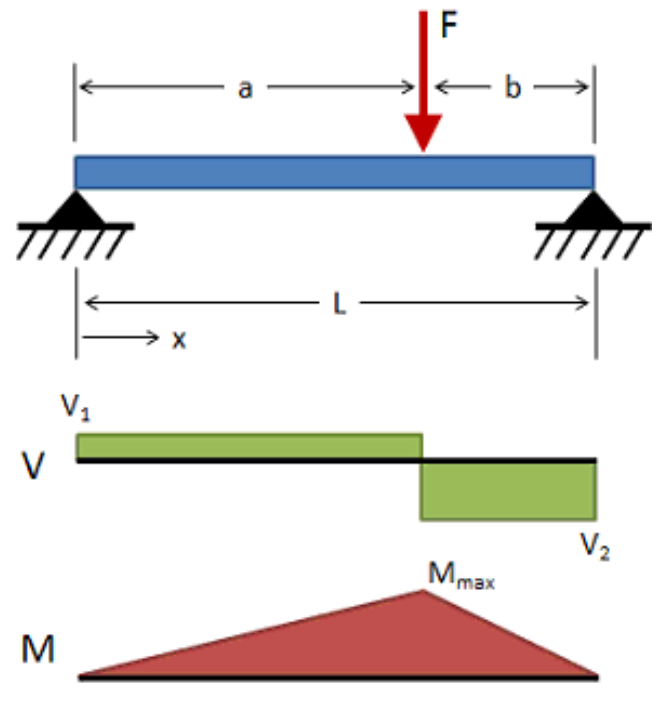
enter image description here

I have been reflecting on how levers work and a larger force can be produced by applying a smaller force at a suitable distance. The answer seems to be in the way internal stress occur inside a bar/beam with larger stresses on the shorter side of the beam. The picture below shows how the bending moment is larger on the side with the smaller lever arm. I see this system as being equivalent to an inverted seesaw with off-center fulcrum with the support on the right experiencing a large force than the left support.

When we open a door from the handle, it is (feels) easier than when we try to open the same door from somewhere closer to the hinge (smaller lever arm). I understand the explanation in terms of torques (our torque matches the resistive torque at the hinge) and the larger the lever arm the smaller our force is. But, more intuitively, it is still had to conceptually understand what happens inside the door to allow us to open the door more easily.

Improve this question
$\endgroup$
1
  • $\begingroup$ what happens inside the door Is the part of the question that will deter people from answering, as we don't know what's 'inside the door'. $\endgroup$
    – Gert
    Commented Dec 24, 2021 at 1:41

1 Answer 1

Reset to default
1
$\begingroup$

At this level of analysis, nothing happens inside the door. At this level of analysis, the door is a rigid body.

The next level of analysis is that the door flexes a bit, but that is imperceptable to the human opening a door in a normal manner. It has nothing to do with the requirement of larger force when pressing the door nearer to the hinge.

As well, the degree and means of flexing the door undergoes is in general fairly complicated. Does it undergo elastic deformation? Does it yield? Does it deform? Are the outer layers significantly different to the inner parts? Are any of these things different under different forces? How do they depend on the size and speed of the deformation of the door? And others one might have fun with in other contexts, such as for example bridge building and calculating safe load on the bridge.

But none of those considerations will be relevant to the ordinary door opening process. You simply won't be able to tell the difference by pushing on the lever. The lever is viewed as a rigid body.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Applying the same force farther from the hinge produces a larger torque and it feels easier to open the door. We apply the force at a certain point external to the door and internal forces are generated that transmit the motion to the entire door. In the thread I referenced, the solid object is modeled as a series of connected springs that stretch to try to conceptually explain how an input force is amplified in to a bigger force beside from using equations .That is why I am thinking that the internal forces are eventually responsible. $\endgroup$
    – Brett Cooper
    Commented Dec 24, 2021 at 13:02

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.