To see an example of where assuming the ratio of the input force to the output force is proportional to the ratio of the arm lengths produces incorrect results, see this other thread. The distance the force moves through at each end (which is the distance you asked about) is more fundamental and your question raised an important issue.
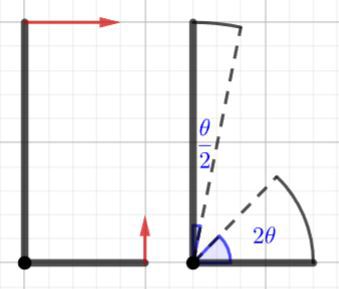
The diagram on the left is a right angled lever, as seen by an observer moving relative to the lever. In its rest frame, the lever has equal length arms with equal forces acting on the ends and is in equilibrium. To an observer moving relative to the lever with a velocity such that the gamma factor is 2, the length of the lever arm parallel to the motion is halved and the force acting on it is also halved according to the laws and transformation of relativity. This means the torque acting on the longer arm is four times the force acting on the shorter arm and according to the traditional lever law the lever should be rotating clockwise in this reference frame, which gives rise to the paradox. In the diagram on the right, the situation is analysed in terms of virtual work. Due to lengthlengths parallel to the motion length contracting, angles transform in an asymmetrical way. The angle the longer arm rotates through is 1/4 of the angle the shorter arm rotates through.
The work done by the longer arm is $W_l = f \times d = F \times \theta/2 \ R = F \theta R /2$$W_i = f \times d = F \times \theta/2 \ R = F \theta R/2$.
The work done by the shorter arm is $W_s = f \times d = F/2 \times 2\theta \ R/2 = F \theta R/2$$W_o = f \times d = F/2 \times 2\theta \ R/2 = F \theta R/2$.
Since $W_l = W_s$$W_i = W_o$, the work in, is equal to the work out and the lever is not expected to rotate by the virtual work theorem, resolving the paradox.