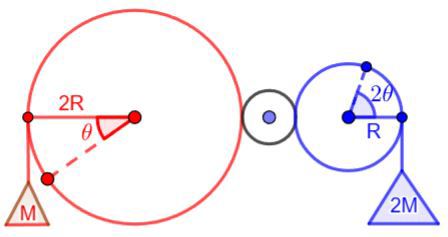
For gears we use the radius of the gears instead of the arm lengths of lever. We can set up some gears to replicate a lever by having two gears that have a third idler gear in the middle, as in the diagram below:
The size of the middle gear is not important, as it is only there to reverse the direction of the third gear. If we attach weights to the perimeters as shown in the diagram, Then a mass of M attached to the larger gear with radius 2R will balance 2M attached to the gear with radius R, just like a lever with one arm twice as long as the other.
The torque applied by the weight on the larger gear on the left is $T_1 = M \times 2R$ and the torque on the smaller gear on the right where $T_2 = 2M \times R$ so the torques are equal in magnitude, just as in the case of the lever.
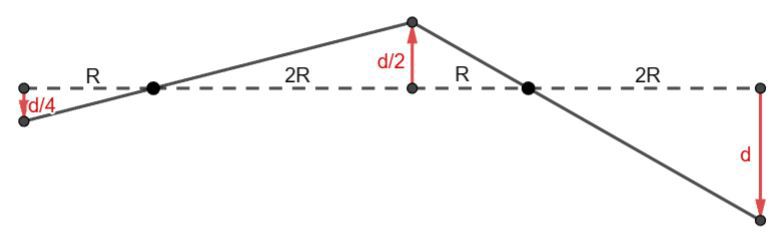
"Now the short arm moves 1/4 the distance, but we get 4x the force"
This description in the video is a bit confusing. They are not talking about the distance the tip of the short transfer arm in the middle moves, because that tip moves the same distance as the tip of the long transfer arm in the middle, because the tips are in contact. They are talking about the distance the tip of the short outer arm on the far left moves, relative the distance the tip of the long outer arm on the far right moves. The diagram below is a schematic of the situation at timestamp 1:46 in the video:
It is now clear the distance they are talking about is the vertical distance the tips of the outer arms move.
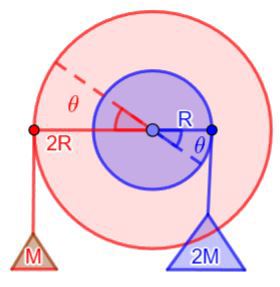
An even better replication of a fulcrum lever using gears (or pulleys) is to have both gears on the same shaft as depicted below:
Now the angle the input lever moves through is the same as the angle the output lever moves through, exactly as in the classic fulcrum lever case. In this set up the mechanical advantage is 2:1 when the larger gear is the input and it is tempting to think that this is due the the larger gear having twice the radius than the smaller gear, but this is not necessarily the case. It might surprise you to learn that the gear set up at the top of this answer has no mechanical advantage! (However, it does still multiply torque and angular velocity at the axles of the gears.) This is because when you pull on the mass labelled M, the mass labelled 2M rises by exactly the same distance as M falls. You need to apply a downward force equal to 2Mg on the left, to raise the larger weight on the right. The mechanical advantage is in fact proportional to the vertical distances the weights move, rather than the ratio of the radii. This concept is known as the "virtual work" theorem of levers. The work is proportional to the force multiplied by the distance it moves through: $$\Delta W = F_{in} \ \Delta d_{in} = F_{out} \Delta d_{out},$$.
where d is the distance the force moves through. It applies universally to gears, levers and pulleys. It is called "virtual work" because it applies even when the forces/gears/levers/pulleys are not actually moving. It is how far the force would move, if the apparatus did move. (You could argue that at a quantum level, nothing is truly stationary.) It also solves the "right angled lever paradox" of relativity and is more fundamental than the traditional law of levers. This is because it is based on conservation of energy and work in equals work out, if losses like friction are ignored.