Why two equipotential surfaces cannot intersect What is the logic and reason behind it?I know that two electric field lines cannot intersect but what about equipotential surfaces?
2 Answers
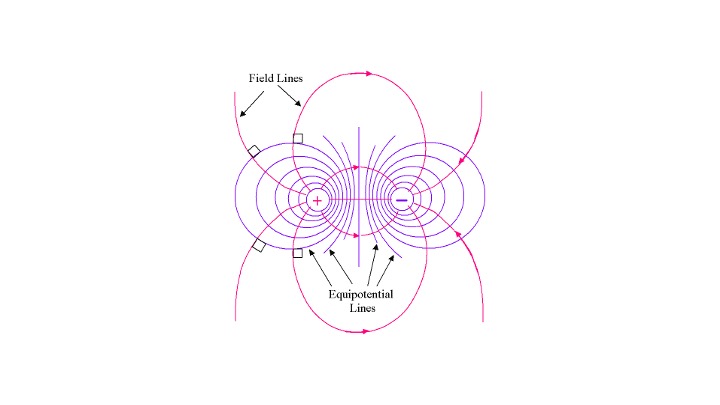
An equipotential surface is a three-dimensional version of equipotential lines. Since no work is required to move charge along equipotential lines, the lines are always perpendicular to the electric field lines along which work is required to move charge. See https://courses.lumenlearning.com/suny-physics/chapter/19-4-equipotential-lines/#:~:text=An%20equipotential%20line%20is%20a,perpendicular%20to%20electric%20field%20lines. It then follows that since electric field lines cannot intersect, equipotential surfaces represented by equipotential lines likewise cannot intersect.
See the figure below.
Hope this helps.
Hope this helps.
An equipotential surface is a surface with a constant value for the potential. So, it takes a certain amount of work to move a charge there from infinity.
If you have two equipotential surfaces with same value that intersect, then you really have one equipotential surface—not two.
But you can’t have two equipotential surfaces with different values that intersect… because that means a point of intersection somehow has two values. That work done to get there from infinity has two values.