Is the electric field strength along an equipotential surface constant?

Question

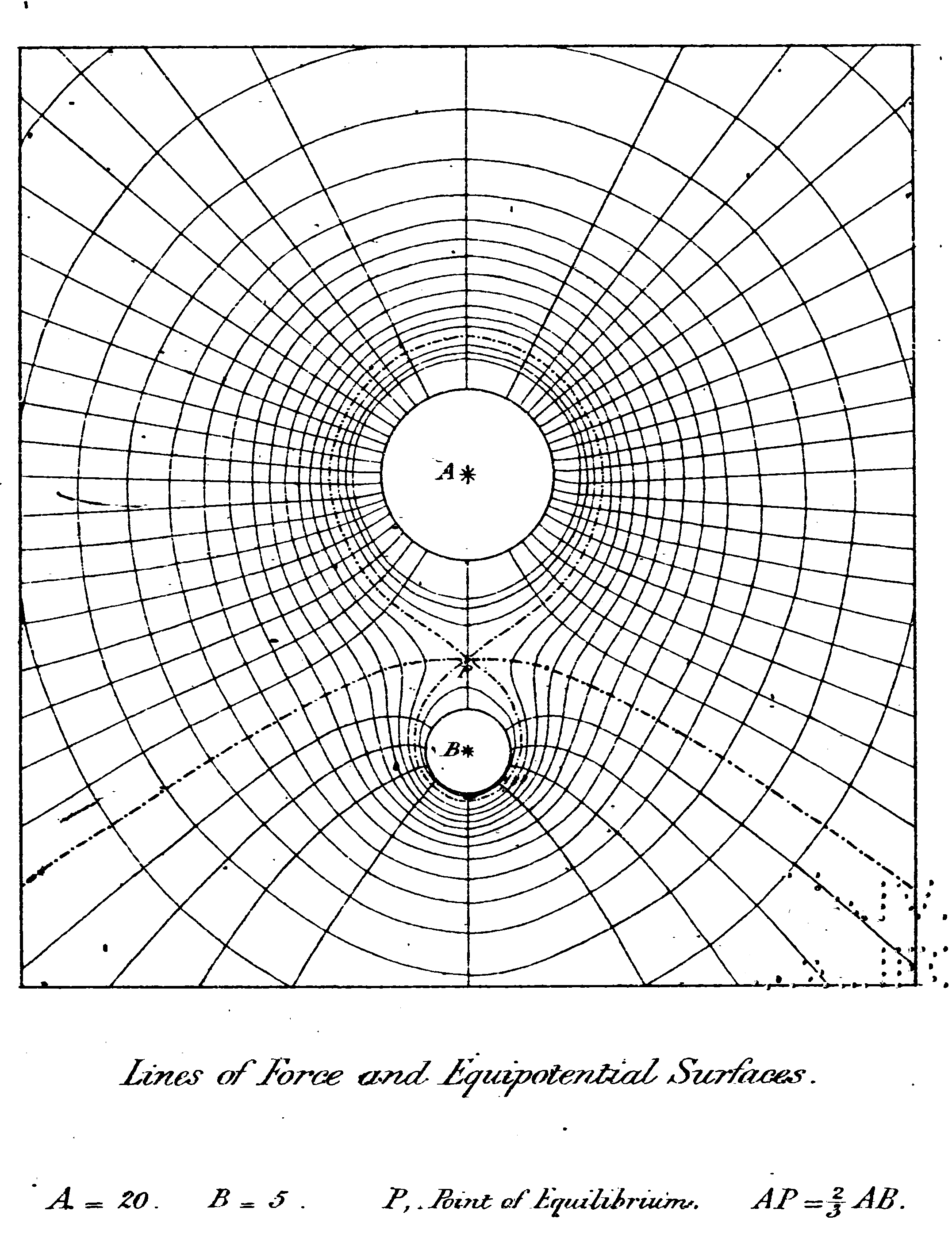
I'm trying to determine whether or not the electric field strength $|\vec{\mathcal{E}}|$ is constant everywhere on an equipotential surface. I know an equipotential surface is defined as $$ S = \{\mathbf{x}\in\Bbb{R}^3\text{ s.t. } V_{\mathbf{x}} = c\} $$ where $V_{\mathbf{x}}$ is the electric potential at $\mathbf{x}$ and $c$ is some constant.

At first it seemed incredibly intuitive, but upon trying to prove it I keep going in circles. It seems true for simple cases such as a single charge (in which the equipotential surfaces are circles surrounding the charge) and parallel plates (in which the equipotential surfaces are parallel lines). I'm not sure about other cases though.

Any help is appreciated! :)

Answer 1

Consider the symmetry plane between two equal magnitude but opposite sign charges. It has the same potential everywhere (zero Volts in the usual gauge). Does it have the same electric field strength everywhere?

For simplicity we'll place a $+1 \,\mathrm{C}$ charge at $+1\,\mathrm{m}$ on the x-axis and a $-1 \,\mathrm{C}$ at $-1\,\mathrm{m}$ on the x-axis.

The symmetry plane is the y-z plane (where x = 0).

Now we compute the field strength at the origin and at $(0,+1)\,\mathrm{m}$ and at $(+1,+1)\,\mathrm{m}$.

• At $(0,+1)\,\mathrm{m}$ this is easy because both field contributions point in the -x direction: \begin{align*} E &= 2 * \left( (9\times 10^9 \,\mathrm{N\,m^2/C^2})\frac{1\,\mathrm{C}} {(1\,\mathrm{m})^2} \right) \\ &= 1.8 \times 10^{10} \,\mathrm{N/C} \end{align*}

• A $(+1,+1)\,\mathrm{m}$ both field contributions point toward negative x, but one points toward positive y and the other toward negative y. The y-directed components cancel out so we only care about the x-directed parts: \begin{align*} E &= 2 * \frac{1}{\sqrt{2}}\left( (9\times 10^9 \,\mathrm{N\,m^2/C^2})\frac{1\,\mathrm{C}} {(\sqrt{2}\,\mathrm{m})^2} \right) \\ &= 6.4 \times 10^{9} \,\mathrm{N/C} \end{align*}

And the field strength continues to drop rapidly as you move away from the origin in the y-z plane.

Answer 2

The claim is false in general. DMcKee has provided a working counter-example, but it helps to look at the reason for it. The electric field is the gradient of the electrostatic potential, which means that its magnitude is essentially inversely proportional to the spacing between two adjacent equipotentials.

That means that if two neighbouring equipotentials curve in different ways then their spacing will increase or decrease, and the electric field will correspondingly change as well. The examples you mention are quite special because their symmetry precludes this type of behaviour, but as soon as you get anything asymmetric like, say, two point charges (of whatever charge)...

^{Image source: J.C. Maxwell, A Treatise on Electricity and Magnetism, public domain.}

you get nontrivial curves with changing spacings. As a good workout you should visualize the equipotentials that are on either side of dmckee's counterexample, and it will clarify where the electric field is stronger - where the potential surface is steeper - and where it is weaker, with a gentler potential slope.

More generally though, and in a nutshell: the equipotential is a contour of equal height, but that says nothing about how steep the surface is at each point.

Answer 3

Mathematically the relationship between electric field $E$ and potential $V$ is $E = -\nabla V$ so that the electric field is normal to an equipotential surface. As you move across an equipotential surface you feel no force in that direction. This is the result that is given for the dipole in the previous answer.