Rope over an un-ideal pulley in an Atwood's machine

Question

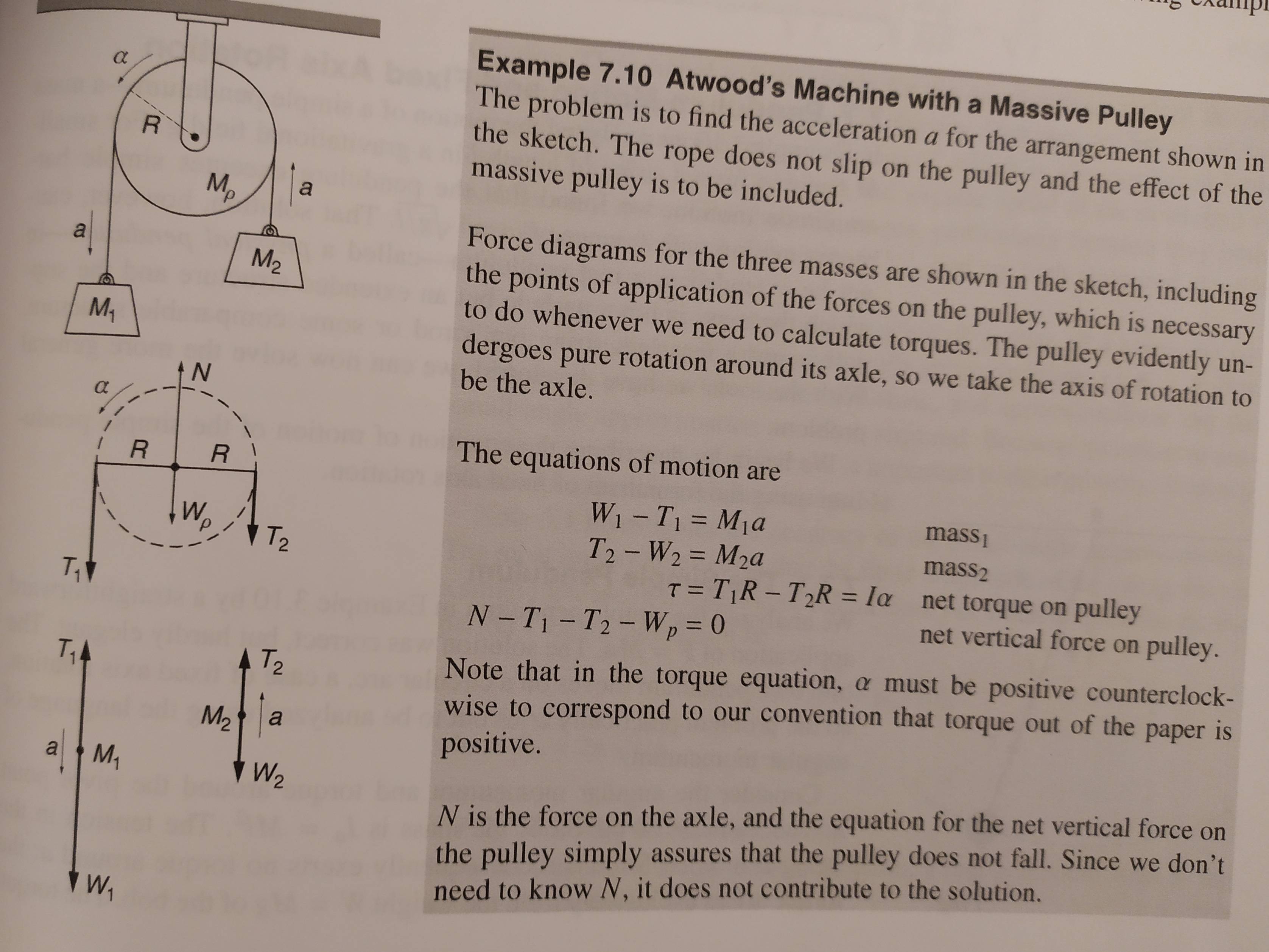
Please consider a pulley with a taut rope over it and two objects (with different masses m1 and m2) hanging on each side. The pulley is not negligible - it has a mass Mp.

In the solution to a problem I'm working on (from An Introduction To Mechanics by Kleppner and Kolenkow), the free body diagram seems to show the following:

1. The tension of the string on each of the two sides of the pulley is different

2. However, on each side, the tension exerted on the pulley is equal (in magnitude) to the tension exerted on the hanging mass

The first point, I think, makes sense to me. I understand that since the pulley is not-idealized, i.e. it does have a mass, we can't just assume that the tension everywhere on the string is the same (which is possible in exercises with idealized pullies). So we assign a different variable - T1 and T2 - to each side of the string.

However, I'm not sure how we can justify the second point. Why should the tension on each end of the string (for each side of the pulley) by the same?

Trying to think it through, I realize that since the string is always taut, all of the little rope pieces move at the same speed. Thus they have equal accelerations. And thus the net force on each little piece of rope should be the same.

However, I was unable to prove algebraically that the tension at the top equals the one at the bottom. This is because, while each little piece of rope along the string is only connected to its other fellow rope-piece neighbors, the piece of rope at the top is touching the pully itself (otherwise it wouldn't exert a force on it). And then I get stuck. Or, I reach equations which claim that the tensions at the two ends (top and bottom) shall be equal only if the rope is not accelerating (which is not what's happening in the problem above).

So, is the 2nd point exhibited in the book's diagram correct? If so, please explain why.

Please note: I'm not looking for help with the exercise. The diagram from the problem made me run into this question, and I'm using it to illustrate my confusion.

Answer 1

Although we are told that the pulley is non-ideal - so the rope exerts a non-zero torque on the pulley - we are told nothing about the rope. So we must assume that the rope is "light" i.e. we can treat it as if it had zero mass.

If the tensions at the top and bottom of, say, the piece of rope to the left of the pulley were not equal and opposite then there would be a non-zero net force on this piece of rope (we don't need to include about the rope's weight because we are treating it as having zero mass). And a non-zero net force on a massless piece of rope would produce an infinite acceleration, which is unrealistic.

Therefore the tensions at the top and bottom of the left-hand piece of rope must be equal and opposite, and the same argument applies to the right-hand piece of rope too.