How do we prove the downwards force of a massless rope on a pulley it wraps around is $2T$?

Question

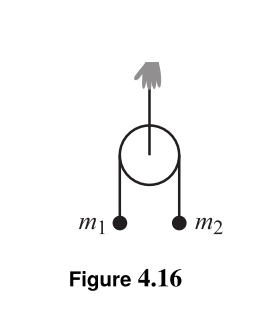
I took this picture out of one of Morin's problem books.

The entire system is under the influence of gravity. The ropes are massless, and the circle is massless.

The original question asks to give the force the hand must exert on the pulley in order to keep the pulley from accelerating downwards. The masses are free to move, as they will if one of the masses is greater than the other. What we want to keep still is the actual circle.

My question has to do with a specific step:

$$F_{hand} = 2T$$

Why is it that the force we must apply from above must be equal to $2T$? I know the obvious answer:

"Well, because if you analyze the system, there's two parts of the rope pulling down on the pulley, each one pulling down with a tension $T$".

But what I want to see is how a rope that has a tension of $T$ running through it and wraps around a circle causes that rope to exert $2T$ on the circle.

The argument that its the net force downwards isn't really enough: if those sections pointing downwards were each hanging from the pulley, then the argument would be enough.

But in this case the rope wraps around the pulley, with each tiny section $dl$ of rope exerting a different amount of force in the vertical direction on the pulley. I'd like to see how the sum of all the forces exerted downwards on the pulley by the rope wrapped over it sum up to $2T$.

My question restated in a simpler manner:

How do we integrate the normal force from tiny pieces of rope over the entire circular pulley in order to get a total vertical force of $2T$ on it?

Here is my work so far:

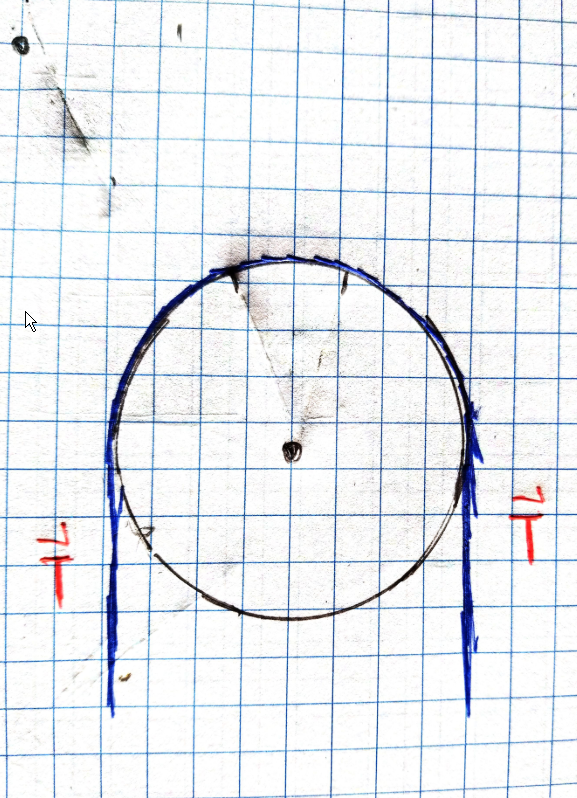
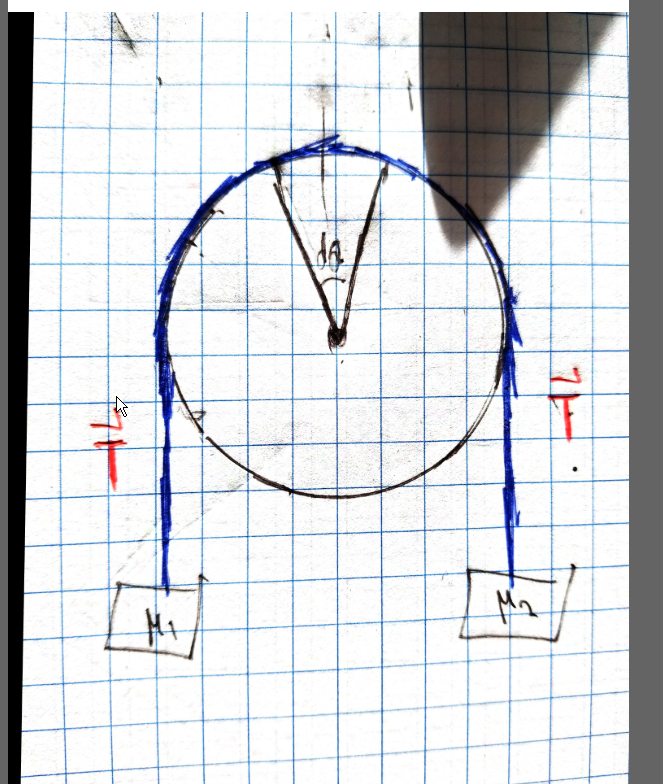
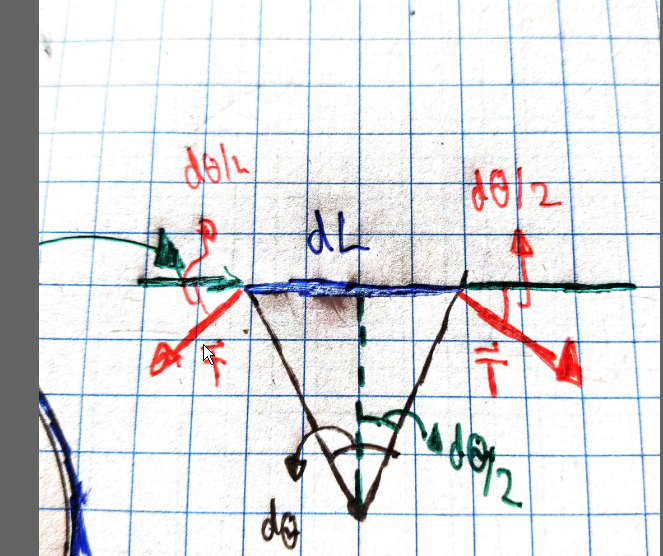
We start with the rope wrapped around the pulley, with a tension of $T$ running through it. I didn't draw the masses at the bottom of the rope, but the net force from the rope is downwards.

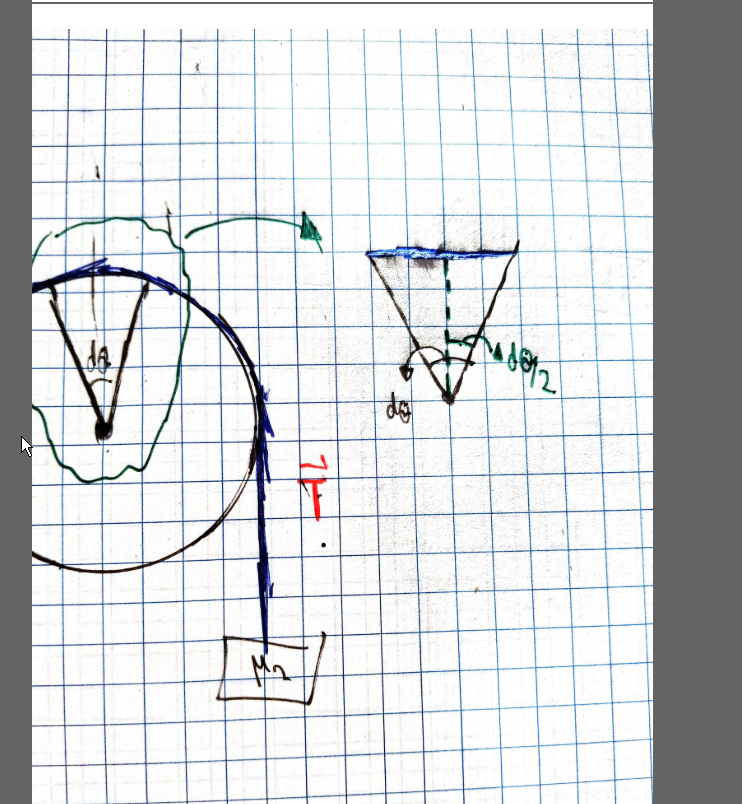
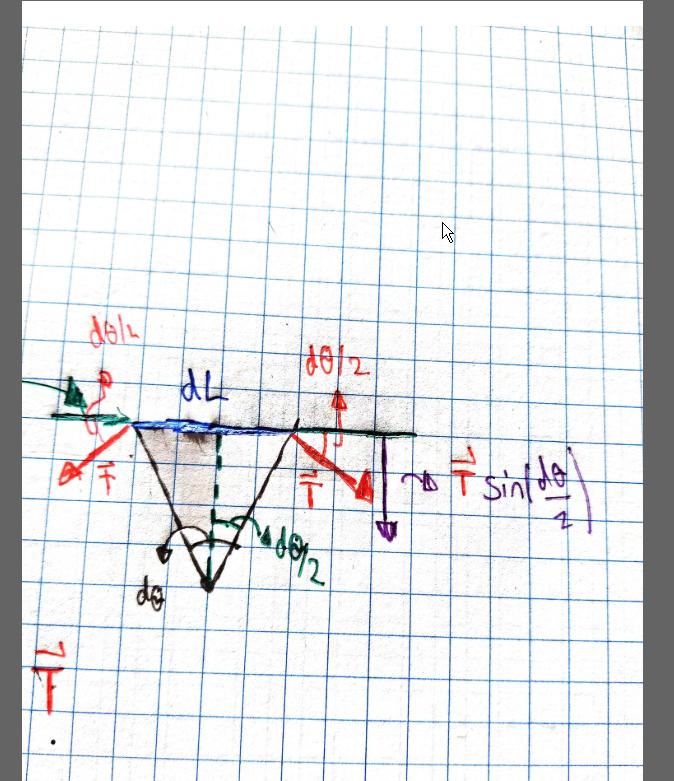
We then consider a little segment of rope $dL$ subtending a little angle $d \theta$

Since we're considering the rope on an infinitely small surface of the circle, it's perpendicular to the radius of the circle.

The ammount of force applied on our $dL$ from the right is $T$, and from the left is $T$.

To get the component of the force towards the circle and therefor the normal force, we need to consider the component pointing to the circle from either side, $Tsin(\frac{d\theta}{2})$, and then add them together to get a total normal force of $2Tsin(\frac{d\theta}{2})$ pointing TOWARDS thae circle (not necessarilly down).

Aaaaand, I'm not sure what to do from here...

Thanks!

Answer 1

Consider the system of the pulley, plus all of the rope directly touching the pulley. The net force on this system must be zero, but it experiences two downward forces totalling $2T$. This must be balanced by an upward force $2T$ exerted by the hand. There is absolutely no need to consider the forces between the rope and pulley here, because they are internal forces.

Now consider the system of the pulley alone. It experiences an upward force $2T$ from the hand, so it must also experience a downward force $2T$ from the rope directly touching it, by normal force.

This is completely rigorous, no integration required. But if you insist on an explicit derivation, simply note that the normal force per angle $d\theta$ is $T \, d\theta$. Furthermore, the vertical component of the normal force is $T \sin \theta \, d\theta$. Hence we have $$F = \int_0^\pi T \sin \theta \, d\theta = 2 T.$$

Answer 2

It does not really matter the details of the interaction between the rope and the circle. Imagine instead a system of particles all moving around, in such a case, if the center of mass does not move, the sum of forces up must be equal to the sum of forces down. You can imagine your system as the circle with the rope but without the masses. In such a case the two forces downards ar T and T, made by each mass over the rope, and the force up is F, because both the mass and the acceleration is zero, then F=2T.