NON-SOLID WATER BELOW 0 degrees Celsius
I assume you intended to say "absolute zero" as it would be explained by thermodynamics--as opposed to simply "freezing point."
From a theoretical perspective, technically, no it can't without defying the laws of physics. But someone did offer a practical explanation that resurrected the worst memories of Calculus I - wherein the limit approaches infinity as your value reaches zero. Of course, calculus makes it clear that it's technically impossible to reach zero; but immediately declares that any value that has reached its limit at nearly 0 is--for all intents & purposes--negligible and therefore zero.
I immediately think of Chemistry's "Heisenberg Uncertainty Pricinple" relating the speed of electrons (in orbit) to their known location within their shell. If we know their location, we don't know their speed; and knowing their speed prevents us from knowing their location. For kinematic measurements to work when [velocity=d1-d2/t], t, or time, must be a positive number. Unfortunately that means we don't know exactly where the electron is--simply that it exists somewhere between d1 & d2 like a blurred olympic sprinter during a photo finish. Alternatively, in order to know both the velocity & exact location of an electron in space & time, then time MUST equal zero. As the denominator...that's not ideal, because if t=0, the equation is 'undefined'; but mildly more upsetting is the fact that 'undefined' equations render time impossible with humans trapped in the void between 2 broken dimensions where objects neither travel, accelerate, or experience gravity--let alone perceive.
So for our world to function without our existence being unrecorded, we believe 2 measureable truths can coexist simultaneously and equally--albeit with some statistical probabilities sprinkled in to inch us closer to that pesky 'zero.'
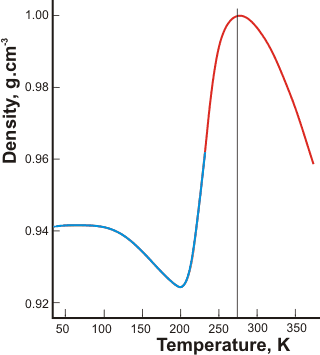
Anyway...potassium & sodium salts are added to water (ice) across most roads in the world because road salt "lowers waters freezing point so that it is no longer capable of freezing at 0C. Thats a magical way of saying salts ionic bonds complex with ice molecules in a manner that disrupts their crystalline structure alignment across every dipole or hydrogen bond. Salt will then increase ice's density to a level thats higher than solid water and liquid fresh water--hence the bouyancy of salt water. If youve cooked, youve likely put salt in a pot of water to change when it boils. Pressure is a whole other essay, but obviously altitude (~atmospheric pressure) and closed pressure systems will have additional effects on water's molecular kinetic energy (prop. to speed & heat).
Also...Mg-based salts are used but they actually melt the salt via the heat emitted from resulting chemical reaction--which is entirely unlike typical road salt that doesn't change temp.