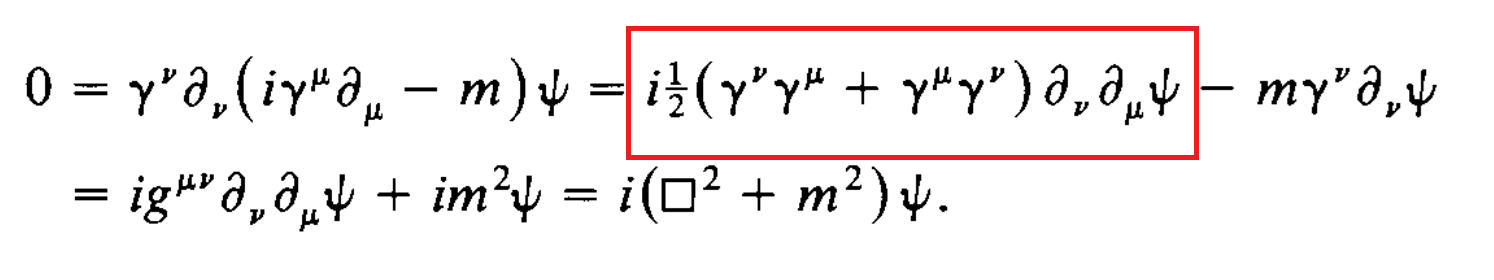Dirac Equation and the Klein-Gordon Equation

Question

I am trying to solve an exercise in Halzen and Martin's Quarks and Leptons book and got stuck on doing some math. The Dirac equation reads $$i \gamma^{\mu} \partial_{\mu} \psi - m\psi = 0.$$ Now, I want to act $\gamma^{\nu}\partial_{\nu}$ on both sides. What I have done was:

$\gamma^{\nu}\partial_{\nu}$ $\left(i \gamma^{\mu} \partial_{\mu} \psi - m\psi \right)$ = 0

Apply linearity and the product rule to get

$\gamma^{\nu} \partial_{\nu} \left(i \gamma^{\mu} \partial_{\mu} \psi\right) - \gamma^{\nu} \partial_{\nu} (m\psi) = 0$

$i\gamma^{\nu} \gamma^{\mu} \partial_{\nu}\left(\partial_{\mu} \psi\right) + i\gamma^{\nu}\partial_{\nu}(\gamma^{\mu})\partial_{\mu}\psi - m\gamma^{\nu} \partial_{\nu} \psi = 0$

I am stuck on simplifying the first two terms. As in the key, they have written it the following fashion:

I am puzzled as to how the quantity in red box was derived. I do understand the process after this though, where they made use of the anti-commutator relation $\left\{ \gamma^{\mu}, \gamma^{\nu}\right\} = 2g^{\mu \nu}$. Can someome walk me through how to get from the last line of my work to the red box?

Answer 1

It's simple if you think about how you change the summation variables.

$\gamma^\nu \gamma^\mu\partial_\mu\partial_\nu=\frac{1}{2}(\gamma^\nu \gamma^\mu\partial_\mu\partial_\nu+\gamma^\nu \gamma^\mu\partial_\mu\partial_\nu)$

This is straightforward I didn't do anything fancy here. Now what you can do is in the second term relabel the variables like this $\mu \rightarrow \nu$ and $\nu\rightarrow \mu$ and then use the fact that partial derivatives commute, so finally one gets:

$\gamma^\nu \gamma^\mu\partial_\mu\partial_\nu=\frac{1}{2}(\gamma^\nu \gamma^\mu+\gamma^\mu \gamma^\nu)\partial_\mu\partial_\nu$