In accelerated pure rolling ,as we know velocity of the bottom most point is zero with respect to ground but what makes it to have zero tangential acceleration. If tangential acceleration is to be zero at that point ,it must have net force zero in horizontal direction.The ground applies friction on bottom most point, which in turn applies some internal force on oher points in the body(due to rigidity of body) causing the whole body to get angular acceleration ,the other points applies the same reaction force on the bottom most point .We don't know the magnitude of internal forces. So on what basis we say that these forces cancel each other to make the tangential acceleration or horizontal acceleration of the point zero?
4 Answers
$\begingroup$
$\endgroup$
The ground applies frictional resistance at that point, yes. But since the wheel (let's say car) isn't slowing down, the transmission is applying torque through the axle, which becomes an equal and opposite force at the wheel: No acceleration, no force (tangentially speaking).
I think a confusing point is bringing in the real-world. It may help to just imagine the acceleration on an abstract wheel rotating at uniform speed, and then transform to a frame that matches the situation. It's clear that velocity at a point goes to zero, and the acceleration at that point is entirely radial.
At least that works for me.
$\begingroup$
$\endgroup$
The ground applies friction on bottom most point
Maybe it does, maybe it doesn't. If the translational and rotational velocities match exactly, no friction will arise.
If there is drag on the object so that it is decelerating, then there will be tangential acceleration on the bottom point.
answered Oct 4, 2023 at 18:29
$\begingroup$
$\endgroup$
One way to deal with the problem is to work in a frame that moves with the wheel.
From that frame, the tangential velocity of the wheel at the contact point matches the velocity of the ground. The relative velocity is zero.
If the car (I suppose the wheel attached to a car) is accelerating, the equation $F = ma$ is no longer valid (in that accelerated frame). There is a force from the ground to the wheel but no acceleration, in the same way that someone inside the car feel a force from the seat on his back without accelerates with respect to the car.
answered Oct 4, 2023 at 22:29
$\begingroup$
$\endgroup$
The wheel is rolling on the $x$-axis in the positive $x$ direction
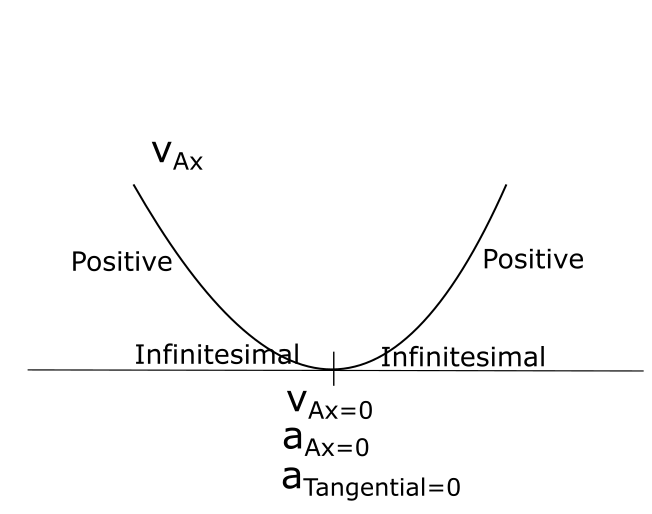
Ahead of the contact point, the points on the lower front edge of the wheel are moving downward, toward becoming a future contact point themselves, but also forward in the $x$ direction. As they approach becoming a contact point, their movement becomes smaller and more vertical, but a infinitesimal positive $v_x$ component remains, trending to zero.
The instant that point $A$ is the contact point, $v_{Ax} = 0$ . Then $A$ starts traveling upwards, again with a positive $v_{Ax}$ component.
When we graph $v_{Ax}$ over time, the big picture looks something like a sine wave with a positive offset. Not knowing the pattern of acceleration, we do not know the exact shape of the curve.
However, when point $A$ is near the instant of contact, the $v_{Ax}$ curve is shaped roughly similar to an upward facing parabola.
The slope of the $v_{Ax}$ curve is the $x$-acceleration $a_{Ax}$. The slope $a_{Ax}=0$ when $v_{Ax}$ is at minimum ( when $v_{Ax}=0$, when $A$ is point of contact ). At that instant, the $x$ axis is tangent to the wheel at point $A$, so $a_{Ax}=0$ is the tangential acceleration.
