Why does the point in contact (having zero net velocity) in pure rolling moves further?

Question

We know that in pure rolling motion the contact point have zero net velocity. So, why does the ball moves further, if the velocity of contact point is zero? And what is the role of centripetal acceleration in pure rolling motion and my teacher said that the work done by static friction on contact point is zero in pure roll, but why?

Answer 1

So, why does the ball moves further, if the velocity of contact point is zero?

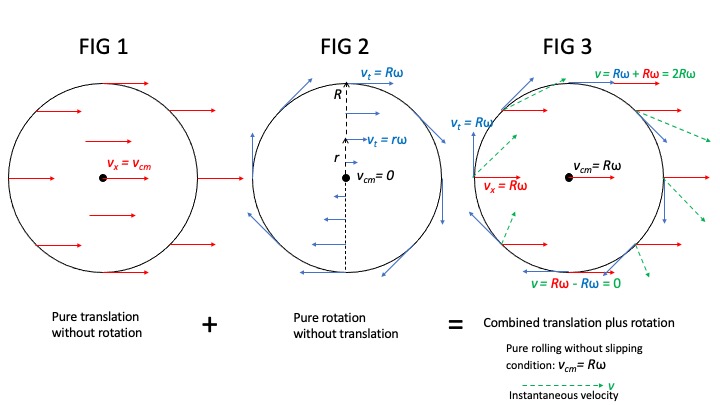
The ball moves further because it is based on the velocity of the center of mass $v_{cm}$, where $v_{cm}=R\omega$ for rolling without slipping where $\omega$ is the angular velocity, and not the instantaneous velocity at the point of contact, which is zero, as shown in FIG 3 below. These values are based on combining pure translational motion of FIG 1 with pure rotational motion of FIG 2.

And what is the role of centripetal acceleration in pure rolling motion

In short, its purpose is to ensure the circular motion of all the particles of the ball. The centripetal acceleration refers to the continually changing direction of the tangential velocity in FIG 2 towards the center of rotation, and is due to the centripetal force pulling in on all particles of the ball to prevent them from flying off due to their inertia. The origin of that force is the intermolecular forces of attraction.

and my teacher said that the work done by static friction on contact point is zero in pure roll, but why?

To answer this question we need to start with the general equation for work,

$$W=\int \vec F \cdot d\vec s$$

or equivalently,

$$W=\int \vec F\cdot\vec v \ dt$$

where $\vec v$ is the velocity of the point of application of $\vec F$

For the example shown in FIG 3 below, where the rolling motion is horizontal and at constant angular velocity, there are no net external forces acting on the ball and therefore static friction is not involved. The surface could just as well be frictionless. The ball will roll continuously due to its translational and rotational inertia per Newton's 1st law.

However if there were a net force or torque force acting on the ball, then static friction would generally be needed at the point of contact for the ball to rotate without slipping. Since the static friction force acts at the point of contact, and the instantaneous velocity as well as the instantaneous acceleration at that point is zero, static friction performs no work. One can think of the static friction force as the mechanism that enables or facilitates rotation without slipping as opposed to being the energy source for the rotation. That source is the applied force or torque.

Hope this helps.

Answer 2

The point of contact does indeed not move (relative to the surface) during the moment of contact.

But the rest of the ball does. It sort of "rolls over" or "tilts over" the contact point. While the contact point remains stationary (translation-wise), the rest of the points of the objects do move forwards.

Think of a star "rolling" down an incline. While a star spike - a leg - touches the surface it doesn't move, but the rest of the start does "roll over" this leg. After the rest of the star has move far enough "over" the contact point, the touching leg lets go and a new leg takes over, whose contact point with the surface now is stationary.

Think of a rolling ball as a star with many, many legs. So many that you can't distinguish them from each other. Each "leg" - which now is so small that we are talking about just a single point on the ball's surface - is stationary while touching the surface. But it also only touches for a brief infinitesimally short moment before it lets go and allows for another point to take over.

Thus the contact point for a rolling ball always only touches the surface during a very, very short moment during which it doesn't slide. When looking at the rolling ball from the outside, since no contact points slide - although they are contstantly being taken over by a new contact point - then the ball in general does not slide.

---

When we have agreed on the above, then your next questions about centripetal acceleration and work done by static friction will be easily answered:

1. Each point in the ball (except for the very centre) experiences a centripetal acceleration towards the ball's centre. This is the effect that keeps all the "particles" that make up the ball together. Had the ball been made out of flour, then the particles would not be kept in place and the ball would immediate disintegrate when spinning. It is the centripetal acceleration of the contact point that causes it to not stay in contact with the surface but rather to be "lifted up" again after the ball has "rolled over it".

2. There is static friction acting at the contact point in whatever point that is touches at any moment. Since nothing moves, then the static friction does no work on this particles (work is defined as a force causing a displacement). Work is being done on the ball as a whole, but the local (net) work done specifically on the particle in contact with the surface is zero.

Answer 3

In pure rolling, the point of contact has centripetal acceleration, which is the primary cause of the particle at point of contact. Since, acceleration is radially inwards, the intial velocity imparted to the particle at point of contact will be perpendicular to direction of motion, and so will be it's displacement. Since friction is acting tangentially and the displacement in radially inward. Work done by static friction is 0.