In Fresnel Diffraction at a circular aperture the central image according to 'Optics' by brij lal and subramanyam will be bright if odd number of full half-period zones can be constructed.But(according to me) an odd number of half-period zones would correspond to 0 amplitude i.e. the central spot will be dark as each half-period zone differs from the next by a phase of pi.
I'd like to know if I'm wrong and why!
If you check your calculation you may differ from any text by how you define odd and even boundaries. See the Wikipedia page on Fresnel Diffraction, for example, to see that the Fresnel integral for the central point in the image plane is proportional to:
$$\int_0^a\rho \, \exp\left(i\,\frac{k\,\rho^2}{2\,L}\right)\mathrm{d}\rho = 2 \frac{L}{k} \exp\left(i\,\frac{k\,a^2}{4\,L}\right) \sin\left(\frac{k\,a^2}{4\,L}\right) = 2 \frac{L}{k} \exp\left(i\,\frac{\pi\,a^2}{2\,\lambda\,L}\right) \sin\left(\frac{\pi\,a^2}{2\,\lambda\,L}\right)$$
for an aperture of radius $a$ with light of wavelength $\lambda$ and with the image plane a distance $L$ from the aperture. Therefore, the apertures for peak intensities at the central point are defined by boundaries between the half period zones are defined by $a = \sqrt{(2\,n\, + 1)\,\lambda\,L}$, where $n = 0,1,2,\cdots$, when the path difference between the centre to centre line and the aperture edge to centre line is $\lambda/2,\,3\lambda/2,\,\cdots$, The apertures for nulls at the centre point are defined by $a = \sqrt{2\,n\,\lambda\,L}$, when the path difference is $0,\, \lambda,\,2\lambda,\cdots$. So this would seem to agree with your text. To help you intuitively, look at the diagram below:
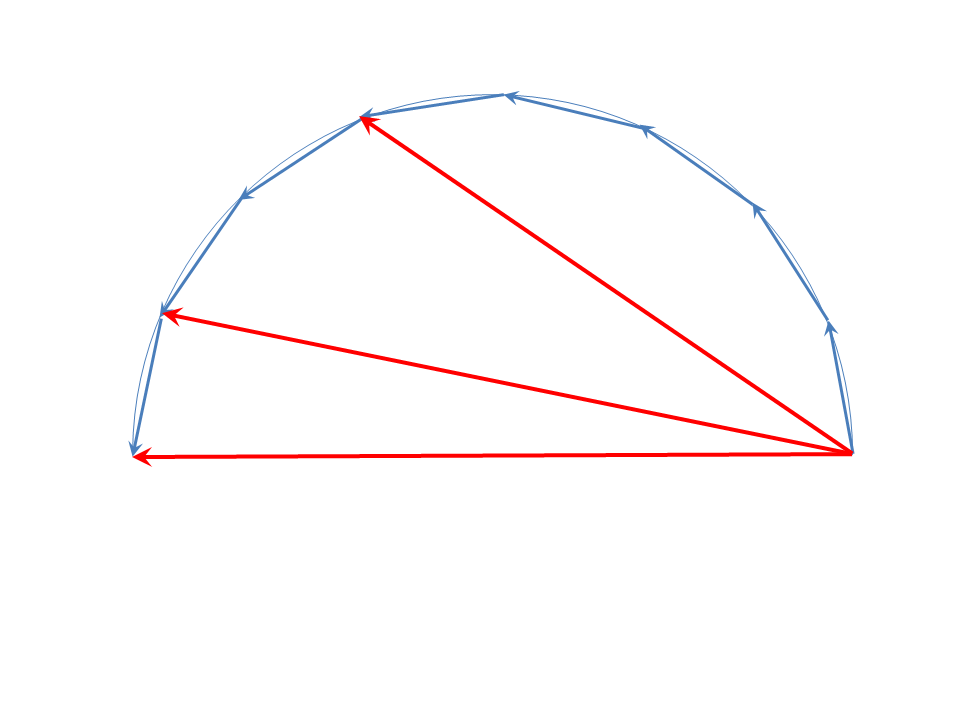
and think of all the little contributions from parts of the aperture to the integral. As your path difference increases from 0 to $\lambda/2$ the little contributions turn around and are actually pointing in the direction opposite to the little contributions from near the aperture centre (blue vectors). But the sum (red vectors) does not begin to decrease in magnitude until one reaches a path difference of $\lambda/2$. So this diagram might help you understand why everything seems to be "out-of-phase" with your expectations.
