0
$\begingroup$

This is a question which was asked

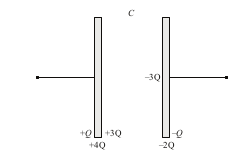
The plates of a parallel plate capacitor of capacitance C are given charges +4Q and –2Q. The capacitor is then connected across another identical uncharged capacitor. Find the final potential difference between the plates of the first capacitor.

Initially, in the isolated capacitor charges will be distributed as below

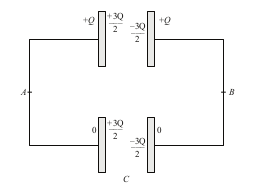
enter image description here

The answer stated that after the connection is made, the inner plate charges will be equally distributed as shown in figure below

enter image description here

Doubts:

  1. Why is charge on outer plate of the original capacitor 0 and of the new capacitor non zero?
  2. Why should the charges on inner plates be equally distributed as emphasized in the answer to it

Im aware that both of them should have the same potential and therefore we can calculate their final charges but it is the charge distribution within their plates which is confusing me.

Improve this question
$\endgroup$
2
  • $\begingroup$ It seems to me that the charge distribution on both the initial configuration and the final one are wrong. $\endgroup$
    – naturallyInconsistent
    Commented Jun 21, 2023 at 9:51
  • $\begingroup$ (a) Yes: on the first diagram the charge on the outer surface of the right hand plate should be +𝑄. (b) The distribution of charges on the inner and outer surfaces of unequally charged capacitor plates can be found using symmetry and Gauss's law; a question about the principles involved might be more appropriate for this site than a question in a homework-like context! (c) On the second diagram, symmetry shows that the 'final' charges on the outer surfaces of the plates should be the same for both capacitors. $\endgroup$
    – Philip Wood
    Commented Jun 21, 2023 at 11:14

0

Reset to default

Browse other questions tagged or ask your own question.