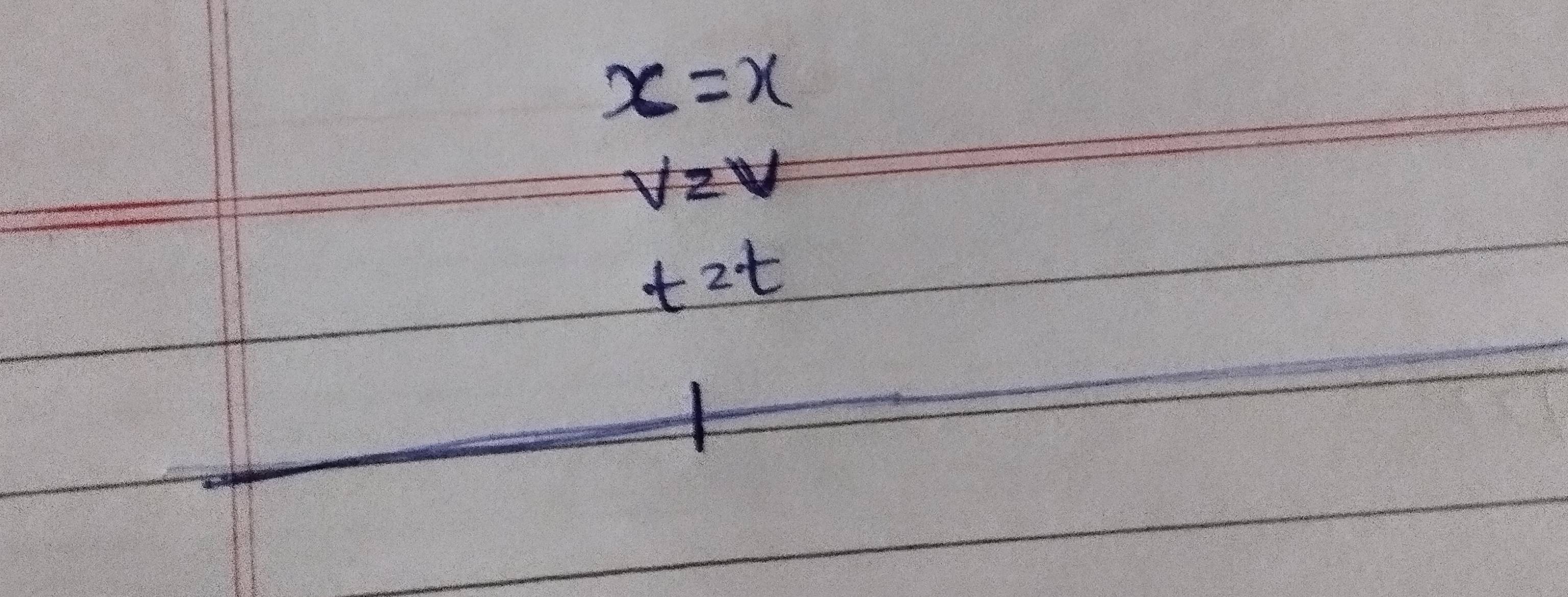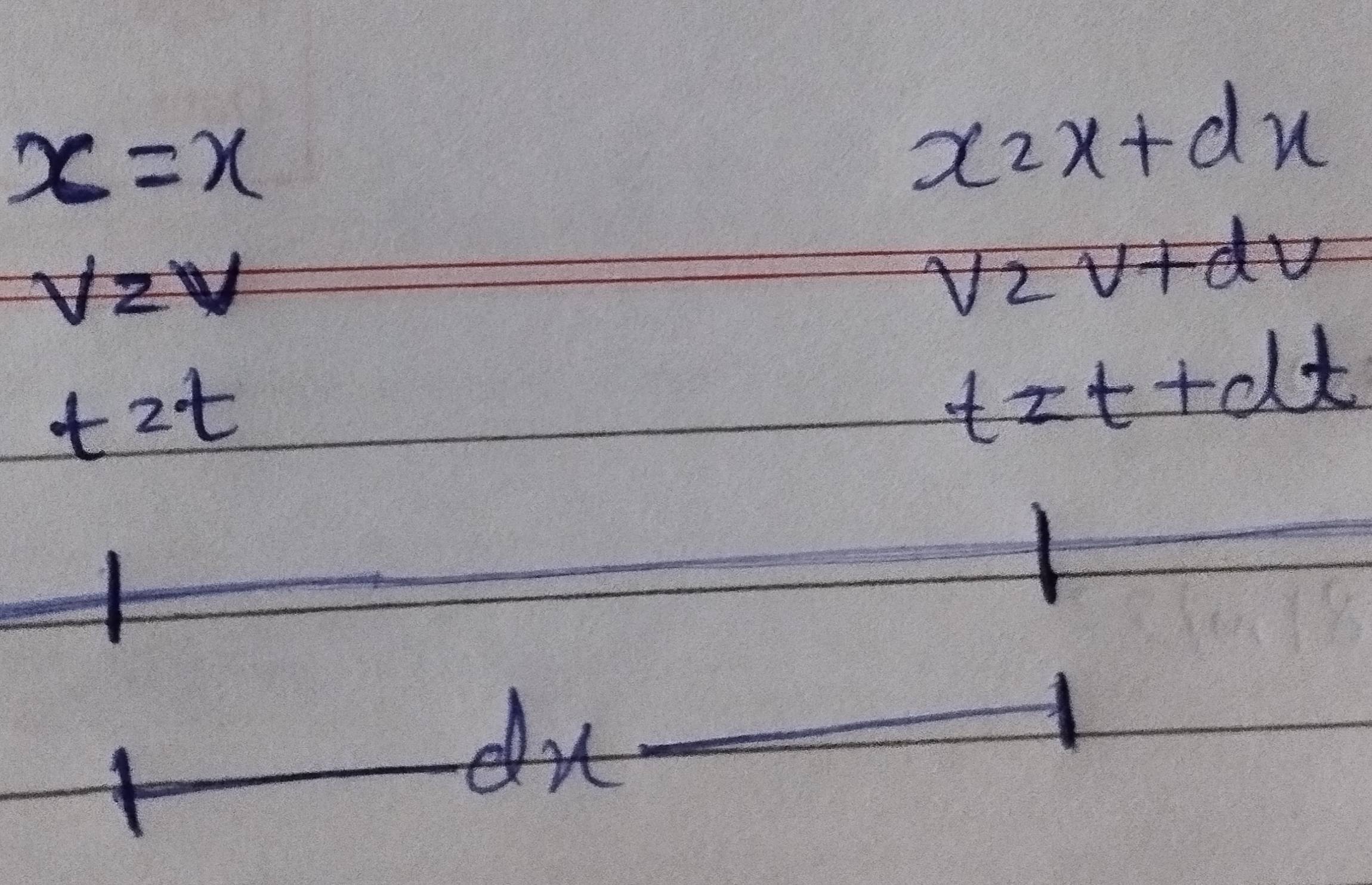0
$\begingroup$

$a$=acceleration

$v$=velocity

$x$=position along x axis

$t$=time instant

My teacher derived the $a$=$v$$dv$/$dx$ formula as follows

Assume a particle at time $t$ is at $x$ position having $v$ velocity

Assume, When time=$t+dt$,it has position as $x+dx$ and velocity as $v+dv$

enter image description here

Therefore, acceleration is

Final velocity-Initial velocity/time interval

Which here, is $v+dv-v$/$t+dt-t$=$dv$/$dt$

Now,we can write $a$=$dv$/$dt$×$dx$/$dx$

And since $dx$/$dt$ is velocity from $x$ to $x+dx$, we can write

$a$=$vdv$/$dx$

enter image description here

Now,I know that when $v$=0, $dx$=0 as well, so the equation $a$=$vdv$/$dx$ becomes indeterminate or undefined

My only question is,can you represent this situation of when $v$=0,$dx$=0 and therefore $a$=undefined/indeterminate according to $a$=$vdv$/$dx$ in proper structured drawings or diagrams only about this formula? (I know there are other ways to find acceleration which don't give indeterminate or undefined value) just talking about this formula, like the diagram my sir just showed?? ,It mathematically and graphically makes sense to me,i can solve the hardest questions of my grade, but i just don't want a calculus proof, I just can't plug in numbers and derivatives,i want to physically understand this formula and also how it becomes undefined "physically" when $v$=0,like a situational based story/diagram would help a lot.

Improve this question
$\endgroup$
2
  • $\begingroup$ To avoid 0/0 take the limit so your values approach, but are not really 0. $\endgroup$
    – Yukterez
    Commented May 12, 2023 at 23:03
  • $\begingroup$ No, for a = const and v = 0 that really means that $\frac{\mathrm dv}{\mathrm dx}\to\infty$ $\endgroup$
    – naturallyInconsistent
    Commented May 13, 2023 at 3:16

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.