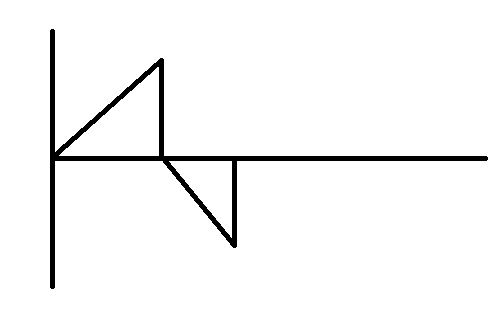
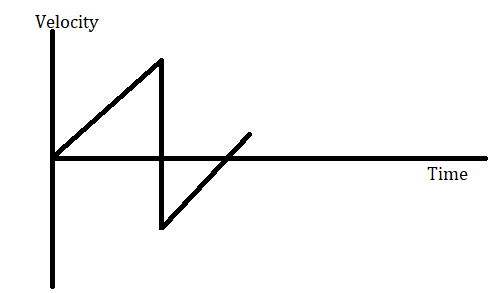1
$\begingroup$

The conceptual problem I am having difficulty with is something like this:

If a bouncy ball is dropped from some height $h$ and rebounds to a height of $0.75h$ in some time $t$ (for example), what is the balls acceleration at the instant when it hits the ground?

Here's what I think:

I assume that the initial speed of the ball is $0 m/s$ and that it reaches a maximum velocity just before hitting the ground. The instant the ball touches the ground, the velocity becomes $0 m/s$ again, after which it starts accelerating upward.

Drawing the velocity time graph, I have something like this:

enter image description here

Not exactly the best diagram, but I hope that it gets my idea across.

My question is then, does this mean that the acceleration at the instant that the ball hits the floor is $\infty$? (since slope of the v-t graph will approach $\infty$)

Improve this question
$\endgroup$
2

3 Answers 3

Reset to default
4
$\begingroup$

No, the acceleration of the ball isn't infinite. What happens is that when the ball touches the ground the face in contact with the ground comes to a stop but the rest of the ball above it slows down more gradually, compressing and distorting the ball like a spring. The ball resists being compressed, and when the its centre of mass comes to a halt the compression is released- the ball expands, sending the centre of mass back up again.

During the process the KE of the falling ball is converted to the PE of compression, which is then converted back to KE with some loss as heat etc.

The process takes a finite time, which is why the acceleration isn't infinite. The acceleration is high, however. It's value will depend on the coefficients of restitution of the ball and the surface it bounces from.

Improve this answer
$\endgroup$
2
$\begingroup$

Considering your velocity-time graph, you start with an apparently positive acceleration, a straight line as the ball hits the floor, then a negative acceleration.

I assume this is just a slight error, but the acceleration should remain constant, but the velocity becomes negative, meaning the graph should look a little more like this: V-T graph

Note that if you were to differentiate this graph you'd get a straight line graph as gravity is constant, and if you were to integrate it you'd get a displacement - time graph which would appear as a series of parabola (again - as gravity is a constant)

Improve this answer
$\endgroup$
0
$\begingroup$

If we have a hypothetical ball and a hypothetical ground that are not compressed and no energy is wasted, this object acts like light hitting a mirror when it hits the ground. The acceleration changes at the moment of impact with the ground are such that in a moment the constant amount of acceleration becomes negative.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.