Doesn't Conservation of angular momentum contradict the need for a centripetal force?

Question

If an object is rotating in space, then there has to be a centripetal force acting upon it to constantly change its direction. I thought if an object begins to rotate in space, it would slow down since there is no centripetal force that keeps it changing direction, but that is contradictory to the conservation of angular momentum law.

Answer 1

The centripetal force may change the direction, but since it's always orthogonal to the moving direction it will never change the absolute momentum / energy.

I thought if an object begins to rotate in space

An object never begins to rotate in space. Nor does a rotating object slow down. If there is no centripetal force, then it just won't rotate but fly apart, each particle tangetial to its "rotation" orbit. This will indeed look like a slowdown if you concentrate on angular speed – but after all that is not a conserved quantity. It's angular momentum that is conserved, with or without centripetal force, and obviously also for a linearly moving object (or multiple such objects):

$$ \mathbf{r}(t) = \mathbf{r}_0 + \mathbf{v}\cdot t $$ $$ \implies \dot{\mathbf{r}}(t) \equiv \mathbf{v} $$ So $$ \frac{\mathbf{L}(t)}{m} = \mathbf{r}(t) \times \dot{\mathbf{r}}(t) = (\mathbf{r}_0 + \mathbf{v}\cdot t) \times \mathbf{v} = \mathbf{r}_0\times \mathbf{v} + t\cdot \underbrace{\mathbf{v}\! \times\! \mathbf{v}}_{=0} = \mathbf{r}_0\times \mathbf{v}. $$ Which is constant.

Answer 2

Content

• Brief answer
• A first explanation
• Reply to Mark Mitchison
• The case of the disappearing angular momentum

The last part may be read directly and explains angular momentum conservation on a simple example.

The answer has been modified following Mark Mitchison's comments

Brief answer

Angular momentum exists independently of any centripetal force. The point to understand is that with any motion, a moving mass has an angular momentum with respect to any point in space you care to choose as center. If the torque of all forces on that mass with respect to that center point is zero, then the angular momentum does not change for that center. That is conservation of angular momentum.

When an appropriate (centripetal) force is applied orthogonally to the trajectory so that the mass goes into a rotation around a specific center, the angular momentum with respect to that center does not change because the torque of that force is zero for that center. But the angular momentum changes for all other points as they are not aligned (except in passing) with the center of rotation and the moving mass. So the role of the centripetal force is to enforce rotation around one specific center. It happens to be such that angular momentum is conserved with respect to that center.

There is no contradiction.

A first explanation

The author of the question states quite correctly that the role of the centripetal force is to keep changing the direction of motion of each fragment of the rotating/revolving body. This centripetal force is orthogonal to the motion, thus never contributes to its speed, only to its direction. So the centripetal force preserve the rotating aspect of motion, not motion itself. If it ceases, motion persist, but becomes linear. So the angular momentum seems gone: more on this below in "the case of the disappearing angular momentum".

The centripetal force can have many sources. For a solid body rotating on itself, it is the internal cohesion forces that keep the molecules together and prevent them from flying apart.

If it is a weight at the end of a rope (ever watched the hammer throw at the olympics) being rotated by a person, it is the rope pulling on the weight that transmit the centripetal force, has long as the person can keep holding it (he can feel the force in his arms). Note that when the person lets go, the weight will fly off a tangent, in a straight line (well, on Earth, it will curve to the ground). Note also that letting go may unbalance the thrower, if the weight is large enough.

If it is a planet in space, the centripetal force is provided by gravity from the sun. Remove the sun, and the planet will fly off a tangent (this is only a - not very good - thought experiment).

A satellite around Earth will also use Earth gravity as a centripetal force. But you could have a similar trajectory in deep space, without a planet providing the centripetal force: you just replace the gravitation by a push from a rocket engine, slowly rotating the orientation of the push so that it stays orthogonal to motion.

Centripetal force can be produced by a normal force, a reaction force from a circular support, as for motorbikes rotating inside a sphere (Motorcycle Sphere Cage Stunt)

I am sure there is more.

Now, what seems really preserved is momentum, so that When an object is rotating and kept in that rotation, preservation of momentum becomes preservation of angular momentum. We first looks at this, and learn better afterwards.

If for some reason the centripetal force ceases to act, the momentum seems preserved in a in linear momentum. A good example is a car with linear momentum and kinetic energy entering a loop.The linear momentum (apparently) induces angular momentum while the car stays bound to the circular motion by the loop. (Fifth Gear Loop the Loop). Actually this would be a better example in space, because on Earth there is a complication coming from the fact that going up the loop has to fight gravity, which does alter speed and momentum. (I do what I can)

Another example is the weight on a string. The angular momentum seems to disappears, leaving only the linear momentum when you let go of the string, thus removing the centripetal force. It can be deadly, as David taught Goliath.

Actually, what I said above is only an approximation, which seems to work when we consider that whatever provides the centripetal force is some kind of immovable reference, like earth for a ball. In reality, there is always a reaction on whatever provides the centripetal force, or ceases to provide it (as the hammer thrower), so that the angular momentum is always preserved, as remarked by Mark Mitchison and as discussed below in my reply to him, and then in a more detailed explanation of a very simple case.

Reply to Mark Mitchison.

I am replying here to Mark Mitchison's comment, as I do not like to leave an error in an answer, if I can help it. I do thank Mark Mitchison for making me think further about it (I am not a physicist) so that I could correct this answer.

I was very careless in my original answer which said, in the car loop example, that when "entering a loop [...] the momentum becomes angular momentum, to become linear momentum again when exiting". And again in the following sentence stating that the angular momentum of the weight "changes into linear momentum" when you let go of the rope.

Indeed, as Mark Mitchison remarks, angular momentum and linear momentum are different physical quantities. However they are not completely unrelated quantities. Angular momentum is to linear momentum what a torque is to a force. And, with appropriate devices, force can be apparently "changed" into torque, and conversely, following precise laws. We do that all the time. This is actually visible in the point mass momentum formula $L = (mv)r$ where $mv$ is the momentum of the mass $m$ with speed $v$ and $L$ its angular momentum with respect to a rotation axis at distance $r$. (More precisely, $r$ and $mv$ should be vectors, and $L$ their cross product.)

My intuition was that what is preserved is linear momentum, and that the preservation of angular momentum can be derived as a consequence, at least in classical mechanics. I also thought that the converse might be possible too. Looking a bit on the web, I do realize that it must be a bit more subtle, both being apparently faces of the same inertial coin. But my current understanding stops here.

Still, my interest on this site is, to a large extent, to be able to explain things with as little math as possible, and some back-of-the-envelope calculations. Math is of course essential, but I never took a formula for an understanding.

So I will try to explain here where the angular momentum goes when the centripetal force disappear.

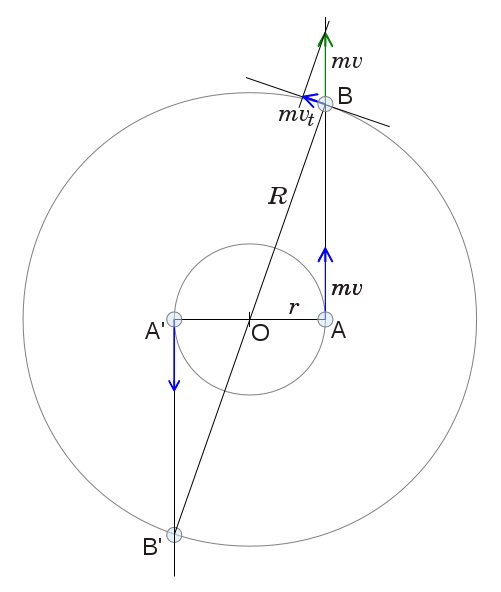
The case of the disappearing angular momentum

Angular momentum is like corpses in detective novels: you may not find them, but they have to be somewhere.

Lets take a simple murder case: it is the case of two equal point masses ($m$ each) tied by a massless rope of length $2r$, turning in freefall (no external forces) with a linear speed $v$. The rope is there to provide the centripetal force, which for each mass is the centrifugal force of the other transmitted by the rope. The two masses turn on a circle, the rope being a rotating diameter of the circle.

The linear momentum of each mass is $mv$, and its angular momentum is $(mv)r$. The total angular momentum is thus $2(mv)r$.

This is not really much different from David rotating his sling, except for the fact that David is heavier than his stones.

Now we cut the rope, letting the two point masses fly of the tangents, which are two parallel lines at a distance $2r$. There is only linear motion, so it seems that the angular momentum has disappeared. Let's find it.

You must first note that since there are no external forces on the system, its center of mass will not move. The masses fly away in opposite directions and at the same speed. Now, you may notice also that the trajectory of each is on a straight line, which is at distance $r$ from the center of mass (on two opposite sides).

Let O be the center of mass, A and A' the positions of the masses on the circle at time 0 when the rope is cut, and B and B' their position at some time $\theta$ later. Consider the linear momentum vector $mv$ on AB. It project as a vector $mv_t$ on the perpendicular to the line 0B in B, which forms with the vector $mv$ on AB a triangle similar to OAB. Let $R$ be the distance between O and B. It is easy to show from relations in similar triangles that $R(mv_t)=r(mv)$.

In other words, the mass in B (and same for B') still has the same angular momentum with respect to the center of mass.

Note that the line BB' between the masses is still a diameter that rotates ever more slowly so as to accomodate straight motion of both masses.

• More generally, For any chosen point in space, the line connecting this point to a moving mass will thus rotate according to the angular momentum of the mass with respect to the point considered and its moment of inertia for that point. This momentum is invariant in the absence of force applied to the mass. Such a force defines a corresponding torque with respect to the center of rotation considered, which can be applied taking into account to the moment of inertia of the mass for that same center.

Indeed, there was another invisible massless rope between the 2 masses, of length $2R$. So at time $\theta$, this new rope is taut, and the radial components of the two linear momentums, on line BB', cancel out providing for each mass a new centripetal force. What is left is the two projections $R(mv_t)$ for each mass, opposite and orthogonal to the line BOB'.

Then the two masses will start rotating again at distance $R$ around the common center of mass, with the same angular momentum as before, as we have computed. The speed will be slower, compensated in inverse proportion by a longer radius.

Our corpse was not dead, after all.

This shows that angular momentum is preserved on its own, but is related to linear momentum, at least in classical mechanics.

(Note that my use of vectors is a bit loose, since angular momentum is a vector cross product).

Sorry for being so long. I write also for myself. I found this work very fruitful as it lead me to another question: What is the difference between translation and rotation?