How current can flow in circuit if voltage is zero?

Question

In A.C. in capacitor circuit $I=i \sin(\omega t+\phi)$ and $V=v \sin(wt)$ Now putting $\omega t=0$ $V=0$ and $I = i \sin(\phi)$ How current can flow even though voltage is zero?

Answer 1

In the simplified model you're being presented, current can flow because there's no intervening resistance. Note that this is a good model to use in normal practice.

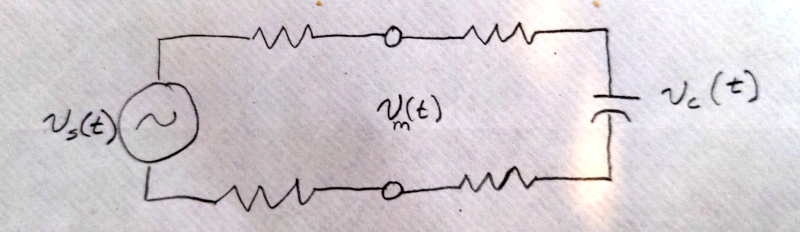
Here's a more detailed model. It's harder to use, and still doesn't fully model any real circuit -- because no model that we can write down is complicated enough to fully model any real circuit.

If you analyze this circuit, with the four resistors set to finite, non-zero resistances, then you'll see that $v_s(t)$, $v_m(t)$, and $v_c(t)$ are all different, because current is flowing. If you then find the limit as $R \to 0$, you'll see that the current is exactly 90 degrees out of phase with the voltage and there's no voltage differences to drive it -- but then, there's no resistance to current flow except for the capacitor's reactive impedance.

Answer 2

Short answer

You can imagine the capacitor is in series with a current source. The current source just charges and discharges it. Let's pretend the current source is currently negative but the voltage across the capacitor is positive. As the current rips electrons away from the capacitor the voltage across the capacitor will at some point hit zero as there are no more charges across it's plates. At this point the voltage is zero but the magnitude of the current is greater than zero. The current being applied will continue to push charges onto the other side of the capacitor and it will start charging negative. The AC cycle continues.

More intuition

Remember that voltage really represents the integral of the electric field between two points. If you assume the electric field is roughly constant (which is normally assumed within the regions calculations are performed in resistors and capacitors) you can say the electric field is proportional to the voltage. The electric field is of course proportional to the force on each particle. Meanwhile, current is the flow of particles and so it is proportional to the velocity of each particle.

In a classical billiard-ball model of electrons one normally thinks of the force accelerating the particles, which smash into parts of the material they're flowing in, generating heat and slowing themselves down. Eventually they reach a maximum velocity which is proportional to the force they are being pushed with. This is where we get $V = IR$.

As an example - it's rather intuitive that a capacitor can hold voltage even when you're not charging it. There's just charged particles sitting in there stuck to either side of the capacitor. In this case, the current is zero but the voltage is positive. If you have $V = IR$ stuck in your head this fact should scare you, but most people find this so intuitive they don't even think about it. But it's the same thing really - a capacitor is not a resistor. You do not need current flowing to produce a voltage and vice versa.

I am saying this just to make you realize that this simple relationship between V and I is not at all fundamental. In capacitors, $V = \frac{1}{C}\int_0^{\tau} I dt$. The simplest model for a capacitor is two parallel conducting plates separated by a dialectic. In a capacitor we measure the velocity of the electrons flowing into the plates as the current, but then they hit a wall and bunch up. We could say the current here is actually zero but we don't say that because the electrons repel an equal number of electrons on the opposite plate on the other side of the capacitor which gives the illusion the current is flowing straight through the capacitor. This is the current we care about since this is the current we see flowing in and out of our capacitor device. As more and more electrons are pushed into the capacitor the amount of electric field crossing between the two plates increases proportionally, leading to a voltage that's proportional to the total number of charges on the plates. This voltage does not represent the force pushing on any of the charges on the plates but rather represents the potential force a particle might feel if it were to be thrown into the space in between the two plates. This is the total voltage over the device since we model the capacitor plates as perfect conductors which means there is no voltage anywhere in the device except in the dialectric gap between the two plates. This gives us the capacitor model. If a negative current is applied we can remove some of the electrons and the field inside the capacitor goes down. Just counting the total electrons on the plates and measuring the voltage across them is what gives us that equation. V and I do not need to be proportional to each other anywhere but in resistors.

Answer 3

A capacitor changes voltage when charge is transferred to and from it, namely when current flows. Without a current at voltage 0V, the voltage could never become non-zero.

The zero-voltage transition marks the point in time where the capacitor stops delivering energy and starts accumulating energy again. The zero-current transition (at peak voltage) marks the point in time where the capacitor stops accumulating energy and starts delivering it again.

Answer 4

I think your problem is that $V=IR$ is a funny equation and AC currents make it funny.

Let's take a moment to remember what $V$ actually means, as in the original definition of $V$. $V$ is change in electric potential, which we created originally with the equation $(\Delta U_E)/q=V$, where $U_E$ is the electric potential energy.

Now, let's remember what $I$ means, actually. $I$ is the movement of charges, which for us, basically means that $I=qnv_dA$, where $q$ is the charge of a single charged particle moving, $n$ is the number of charged particles moving, $v_d$ is the drift velocity, and $A$ is the cross sectional area of the wire we are analyzing.*

So, now we remember what $V$ and $I$ are, we can use our knowledge of mechanics--not E&M but mechanics--to understand why $V=IR$ doesn't work here. The reason why charges were moving was because there was a potential gradient, and so they felt a force and gained a velocity. When you say "when $V=0$," you actually mean "$V_{source}=0$" (remember $V$ is a change in electric potential, which means we need to specify a beginning measuring point and an end measuring point; in this case, we are measuring from one end of the source's terminal to the other end of the source's terminal). What this means is that when $V_{source}=0$, the electrons in the wire feel no force from the source because $\boldsymbol F_E=\boldsymbol\nabla U$ and because $V=0$, which implies $\boldsymbol F_E=\boldsymbol\nabla U=0$. In other words, all $V_{source}=0$ means is that the charged particles are not changing their speed (and therefore $I$ is constant) because the particles feel no force, and therefore have no net acceleration.**

Now, you may be asking, "what's with $V=IR$, then?" Then reason why such an equation worked is because we were working with DC circuits, and not AC circuits. What happens in a wire is that there is a sort of friction, and this friction that the electrons feel is dependent on their speed. So, the faster that the electrons go, the more friction that they feel. When we have a DC current, we have a constant force from a battery, so the electrons get to a sort of 'terminal drift velocity,' kinda akin to 'terminal velocity' when you are, say, parachuting out of a plane.

$V=IR$ works for DC circuits and certain special situations for other circuits, but you have to be careful when you apply it to AC circuits. I will mention, you can say for a series AC circuit that $V_{source,max}=I_{max}R$. The closest similar equation would be $v_{source}(t)=i(t)z$, where $v_{source}$ and $i$ are now functions of time, and $z$ is the impedance, and not the resistance.

I hope that this helps (and that I didn't make any mistakes)!

*I am assuming uniform current for simplicity

**This is clearly a bit of a simplification, evidence of this is clear when just making a loop. The charged particles have to undergo some sort of acceleration to move in a loop. With that said, this point is insignificant for this discussion.