How can current have a value when an ac voltage at a certain moment is zero?

Question

Suppose we have an AC circuit where the current leads the voltage, as in a pure ac capacitive circuit(circuit with ac power supply, capacitor and the ohmic resistance is negligible) for example.

so sometimes the voltage is zero and, I think, that means there is no pushing force for the electrons to flow but current doesn't equal to zero, it will be max(in the mentioned circuit) or any other value(in any other circuit), so what is the pushing force for the electrons at that moment causing them to flow?

Answer 1

Cor a capacitor the current is proportional to the rate of change of voltage across it with the constant of proportionally the capacitance of the capacitor $I = C \frac {dV}{dt}$.

Graphically and diagrammatically the sequence of events is shown below.

You have asked why it is that the current is a maximum when the voltage of the supply is zero which occurs at time c.

One way to explain it is that if you did not query the large current just before or just after the voltage of the supply becomes zero how do you explain the current instantaneously becoming zero when the voltage of the supply is zero.
So one answer is to say that the electrons have inertia and although there is no voltage applied they continue to move as there is no force acting to stop them moving.
This is equivalent to having an oscillating spring-mass system and the mass moving through the static equilibrium position when the net force on the mass is zero.

In fact the circuit does have some inductance but the inductive reactance is very much smaller than the capacitive reactance.
So in terms of energy at time a all the energy is stored in the capacitor's electric field.
At time b the stored energy in the capacitor is decreasing and energy is being sent back to the voltage source and some is stored in the inductor's magnetic field.
At time c thew capacitor has lost all of its energy some of which has been returned to the voltage source and the rest is stored in the magnetic field of the inductor.
But here again the current cannot collapse to zero instantaneously at time c as the inductance in the circuit prevents it from doing so.

Answer 2

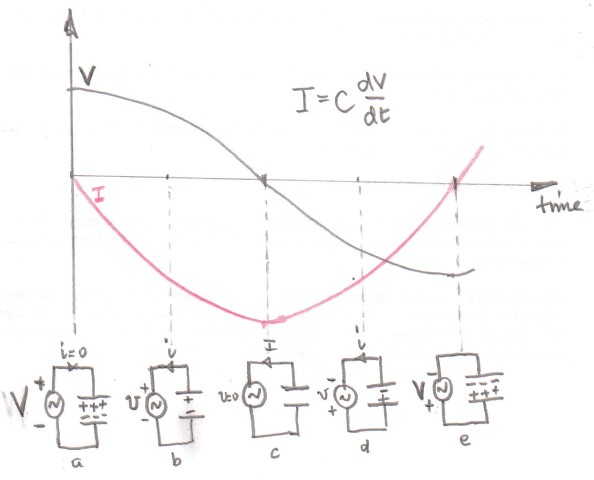
so sometimes the voltage is zero and, I think, that means there is no pushing force for the electrons to flow but current doesn't equal to zero, it will be max(in the mentioned circuit) or any other value(in any other circuit), so what is the pushing force for the electrons at that moment causing them to flow?

The voltage isn't the only "force" pushing the electrons throught the wire. There is also induced vortex-like electric field. Its effect on current is often quantified by a quantity called "electromotive force" or emf. This quantity is work that would be done on elementary positive charge if it was transported along the wire from one to the other terminal. The equation relating current $i$, voltage $U$ and emf is

$$ U + emf = Ri $$

So if at some instant voltage $U=0$, we have $Ri = emf$; the total driving force is that of induced electric field.

If the resistance of the circuit is low (no resistive load connected), the emf and U have opposite effect on the current most of the time (they are almost in antiphase), so they almost cancel each other and the current is low.