In the simplified model you're being presented, current can flow because there's no intervening resistance. Note that this is a good model to use in normal practice, because you can never.
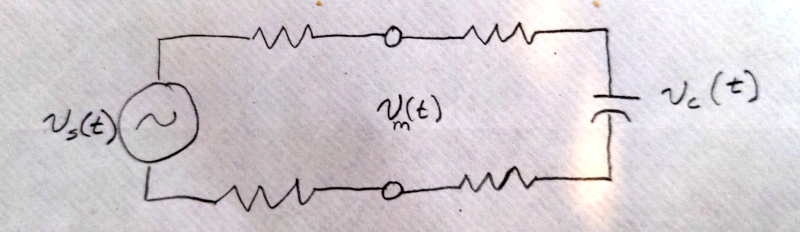
Here's a more detailed model. It's harder to use, and still doesn't fully model any real circuit -- because no model that we can write down is complicated enough to fully model any real circuit.
If you analyze this circuit, with the four resistors set to finite, non-zero resistances, then you'll see that $v_s(t)$, $v_m(t)$, and $v_c(t)$ are all different, because current is flowing. If you then find the limit as $R \to 0$, you'll see that the current is exactly 90 degrees out of phase with the voltage and there's no voltage differences to drive it -- but then, there's no resistance to current flow except for the capacitor's reactive impedance.