1
$\begingroup$

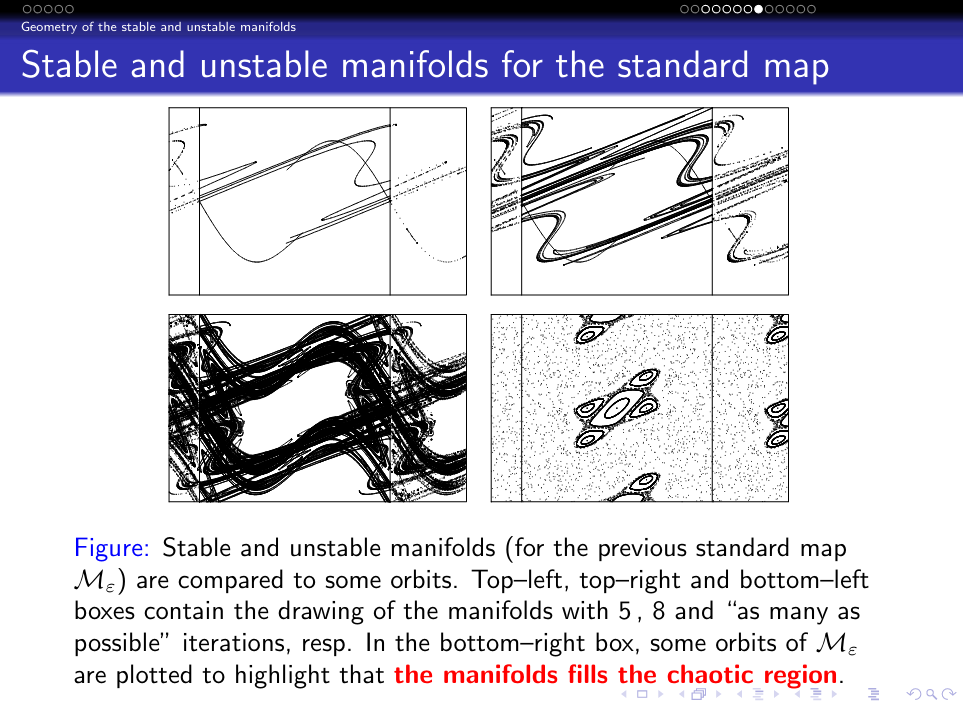
There is a quite classical description of chaotic systems based on the behaviour of stable and unstable manifolds around a stationary point of the Poincaré section. It is presented, for example, [here, in slide 9][1], where a sketch of the stable and unstable manifolds on the Poincaré section are drawn by hand.

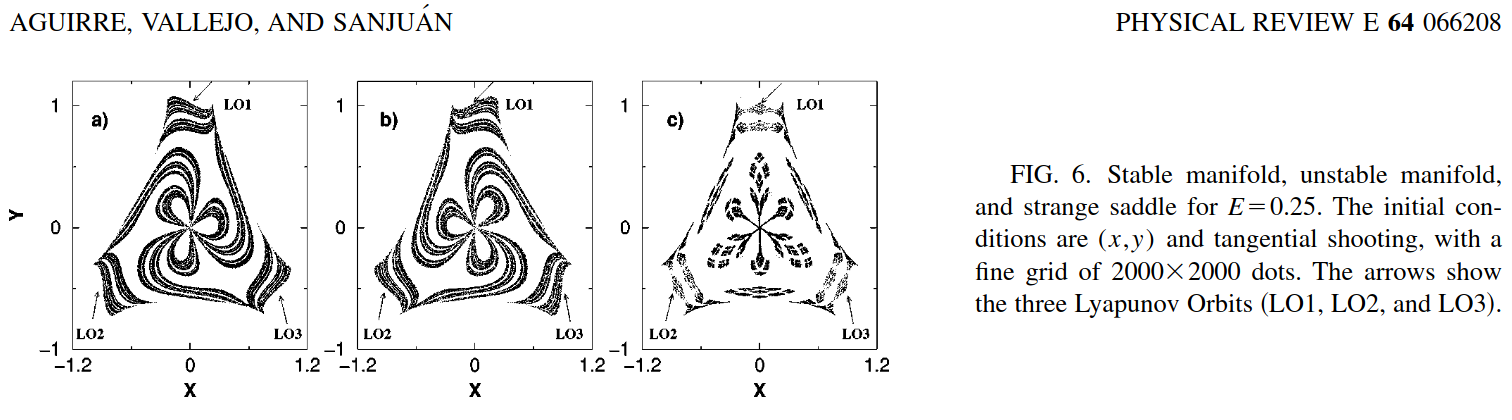
Now I am looking for a numerical study that reports the graphs of the stable and unstable manifolds, quantitatively evaluated (not just made by hand). Possibly, it should be done for Hénon-Heiles equations, or, alternatively, for another chaotic Hamiltonian system. It is important that it reports the conditions, i.e. the coordinates of the fixed point, so that I can exactly reproduce the calculation.

EDIT: I emphasize that I am looking for an example with the Henon-Heiles system. At least, with a Hamiltonian system. I see various examples with discrete maps, but there it is much easier to find a fixed point.

EDIT 2. I could calculate the fixed point and the stable and unstable manifold but finding such a picture in an already published paper would help. Here is the reason. I am calculating some parameters in various points of the phase space. I represent them on the Poincarè section. I would like to qualitatively show how they behave near fixed points and stable and unstable manifolds. The topic of my paper would not be this comparison, nor the calculation of stable and unstable manifolds, thus I would like to refer the reader to a paper, without having to document the procedure of the calculation.

[1]: http://www.mat.uniroma2.it/~locatell/school1-astronet2/material/lezione_Loca1.pdf "KAM Theory and Applications in CelestialMechanics – First Lecture: Chaotic Motions in Hamiltonian Systems", Ugo Locatelli, University of Roma “Tor Vergata”, lecture, 14-th of January, 2013.

Improve this question
$\endgroup$
1
  • $\begingroup$ Minor comment to the post (v2): Please consider to mention explicitly author, title, etc. of link, so it is possible to reconstruct link in case of link rot. $\endgroup$
    – Qmechanic ♦
    Commented Nov 18, 2022 at 18:50

2 Answers 2

Reset to default
1
$\begingroup$

In the chaotic case these manifolds cross infinitely many times and actually foliate the whole accessible space.

A numerical approximation of a finite segment of the manifolds is probably what you're looking for, and that is already given in the presentation you link (page 14):

stable and unstable manifolds for the standard map slide

If you use one of the usual methods for producing these pictures, even guessed initial coordinates should allow you to reproduce these plots.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Well, this is not for the Henon-Heiles system. It is not even a Hamiltonian system. It is a discrete mapping that preserves the area, so it is similar to a Poincarè section of a Hamiltonian system, but it is not really. For discrete maps, it is much easier to find fixed points, just by symbolic calculation. $\endgroup$
    – Doriano Brogioli
    Commented Nov 18, 2022 at 20:07
  • 1
    $\begingroup$ @DorianoBrogioli This indeed isn't the Hénon-Heiles system — which seemed fine before the question edits. Please also notice this mapping is a Hamiltonian system just as much as the Hénon-Heiles Poincaré map referred in the question — after all the Standard Map also is the Poincaré map of a continuous system, namely the kicked rotor. $\endgroup$
    – stafusa
    Commented Nov 18, 2022 at 21:13
1
$\begingroup$

What about this paper (e-print): Wada basins and chaotic invariant sets in the Hénon-Heiles system, J Aguirre, J C Vallejo, M A F Sanjuán, Phys. Rev. E 64, 066208 (2001)

enter image description here

Improve this answer
$\endgroup$
9
  • $\begingroup$ The typical image of the Henon-Heiles chaotic domains is a y dot vs. y Poincarè cut, while here the stable and unstable manifolds are shown in y vs. x plane. I am wondering if there is any reason preventing to use the y dot vs y section (I do not find any!). $\endgroup$
    – Doriano Brogioli
    Commented Nov 19, 2022 at 10:10
  • $\begingroup$ @DorianoBrogioli Good question. Without having studied the paper to say for sure, I think it's due to their being interested in scattering... $\endgroup$
    – stafusa
    Commented Nov 19, 2022 at 10:24
  • $\begingroup$ Yes, it seems that they study a condition in which the trajectory can escape. Do you have an example in which the trajectories are confined? $\endgroup$
    – Doriano Brogioli
    Commented Nov 19, 2022 at 21:14
  • $\begingroup$ @DorianoBrogioli As far I understand escape is a feature of the original system. As stated in page 77 of the original paper: "$E=\frac{1}{6}$ is the energy of escape in the potential [...] the star can eventually escape to infinity, if the orbit is ergodic." $\endgroup$
    – stafusa
    Commented Nov 19, 2022 at 21:31
  • $\begingroup$ I see that the paper focuses on that regime, but, in Henon-Heiles system, with small enough energy, the trajectories are confined. I find it surprising that, although the Henon-Heiles system is well known, although it is often used as an example of the Poincarè section, in confined conditions, no study of the stable and unstable manifolds is available! $\endgroup$
    – Doriano Brogioli
    Commented Nov 20, 2022 at 21:44

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.