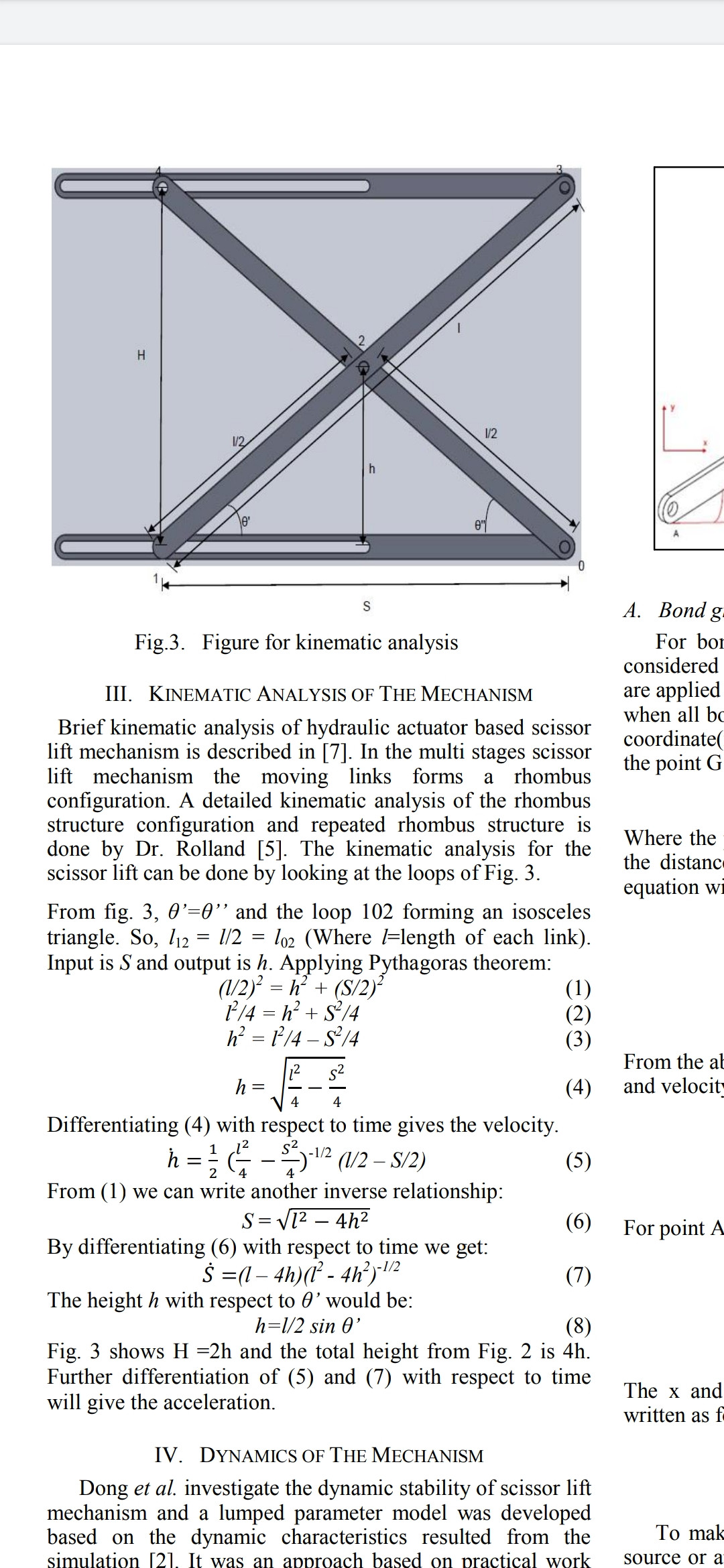
I found a solution for a problem I've been struggling with recently for a scissor lift table horizontal and vertical velocities. This research paper shows that both are dependent on $l$, $S$ and $h$. But the problem is l is a constant since it's the length of a steel bar that is supposed not to change, however the writer derived it first and applied derivative rule as if l was a variable. Does this mean this solution is wrong?
Here it is:
You are right. The equation for h'(t) is wrong the true equation would be $ 2h'(t)=(l^2-S^2(t))^{-1/2}*2S(t)S'(t)$ same in the equation vor S'(t) the h'(t) is missing and l'=0
you have one degree on freedom which is $~S~$
from the constraint equation
$$ h^2+\left(\frac S2\right)^2=\left(\frac l2\right)^2\tag 1$$
you obtain
$$h=h(S)=\frac 12\sqrt{l^2-S^2}$$
thus $$\dot h=\frac{\partial h}{\partial S}\,\dot S= -\frac 12\,{\frac {S}{\sqrt {{l}^{2}-{S}^{2}}}}\,\dot S$$
with $$\dot S=f(t)\quad ,S(t)=\int f(t)\,dt+S_0$$
and $~S_0=\frac l2\,\cos(\theta)~$
you can obtain the velocity $~\dot h(t)~$ and the position $~h(t)=\int \dot h\,dt$
Notice
$~S(t)\le l$
