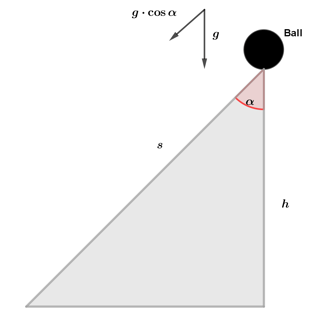
Let us consider two hills of the same height, with one drastically steeper than the other. Ignoring friction and air resistance, is it true that if we release a ball at the top of the hills, their speed will be the same at the bottom?
I've considered equating the loss of the Gravitational Potential Energy with the gain in Kinetic Energy of the balls (of same mass). However, I feel as though there is something I'm missing there.
The discomfort in my thinking is this. In the steeper hill, though the component of Gravity acting in the direction of motion of the ball is substantially larger than in the case of the less steep hill, would that not also mean that the ball would travel for a much shorter period of time (in turn, giving it less time to accelerate due to gravity)?
I can also be completely wrong and I acknowledge that but any clarifications (with the supporting math behind it :) ) would be greatly appreciated!