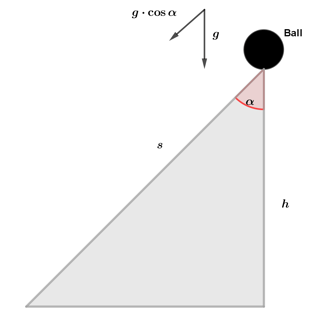
You may also want to calculate this more explicitely. Consider the equation of motion
$$s(t) \enspace = \enspace s_0 + v_0 \cdot t + a_0 \cdot \frac{t^2}{2} \quad ,$$
where $v_0$ is the initial velocity and $a_0$ is the initial acceleration (which will be constant along his path). If the hill is of height $h$, then the distance the ball will have to roll before reaching ground level is
$$s_0 = \frac{h}{\cos \alpha}$$
where $\alpha$ is the steepness of the hill (see Figure). Therefore, in this setting the ground level corresponds to $s = 0$.
The acceleration is dependend on the steepness as well and is of magnitude $g \cdot \cos \alpha$. We assume $v_0 = 0$. Inserting this into the equation of motion, one finds
$$s(t) \enspace = \enspace \frac{h}{\cos \alpha} - g \cdot \cos \alpha \cdot \frac{t^2}{2} \quad .$$
Note the negative sign in the second term. It is because we "start" at distance $s_0$ and want to go down to $s = 0$, so the acceleration is negatively directed. The time $t_1$ needed to reach ground level, i.e. at $s = 0$, is
$$s(t_1) = 0 \quad \Longrightarrow \quad t_1 = \sqrt{\frac{2h}{g \cdot \cos^2 \alpha}} \quad .$$
The velocity after said time is
$$s'(t_1) = g \cdot \cos \alpha \cdot t_1 = g \cdot \cos \alpha \cdot \sqrt{\frac{2h}{g \cdot \cos^2 \alpha}} = \sqrt{2hg}$$
which coincides exactly with the result "Miyase" obtained. It is independent of the steepness of the hill (represented by angle $\alpha$).
In other words, the acceleration on a steeper hill is larger than on a less steeper hill, but it acts on the body not as long as it does on the less steeper hill and so both balls end up with the same velocityspeed.