The area enclosed by a loop is the total work done. If you draw a square, isn't that the maximum amount of work done possible instead. Why is the isothermal/adiabatic path the most efficient instead of a simple square? It seems that an isobaric path gives the most work per area.
It seems that an isobaric path gives the most work per volume.
My first observation regarding your drawing of the cycle is that while you are showing heat being added during the isobaric expansion work, you do not show that heat is also being added during the isochoric pressure increase which produces no work. For the Carnot cycle all of the heat added produces work.
But more importantly, it also appears that you are comparing the work done in the isochoric-isobaric cycle with the work done in the Carnot cycle when both cycles operate over the same range of volumes, as shown in FIG 1 below. The problem with the comparison is that the isochoric-isobaric cycle is operating over a greater temperature range than the Carnot cycle. Note that in FIG 1 for the isochoric-isobaric cycle the maximum temperature is greater than that of the Carnot cycle and minimum temperature is less than the Carnot cycle. Thus in terms of thermal efficiency, it's mixing apples and oranges.
The Carnot cycle is the most efficient cycle over a given temperature range. So to compare the work and efficiency of your cycle with the Carnot cycle, you need to compare the two over the same temperature range, as shown in FIG 2 below.
Hope this helps.
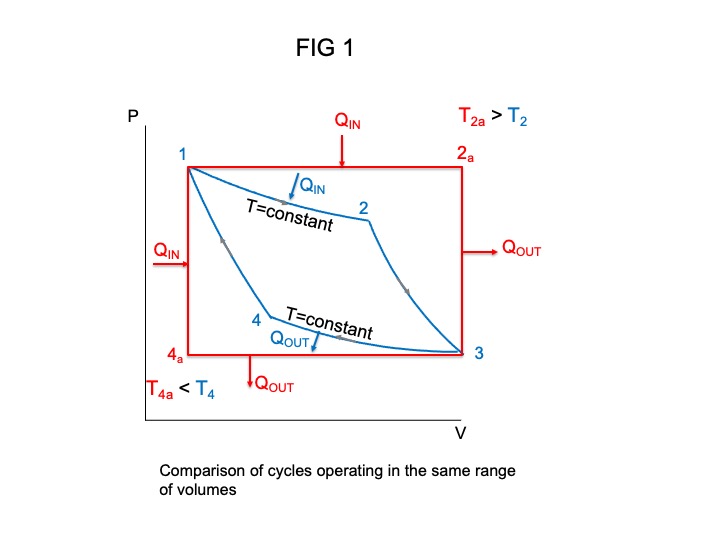
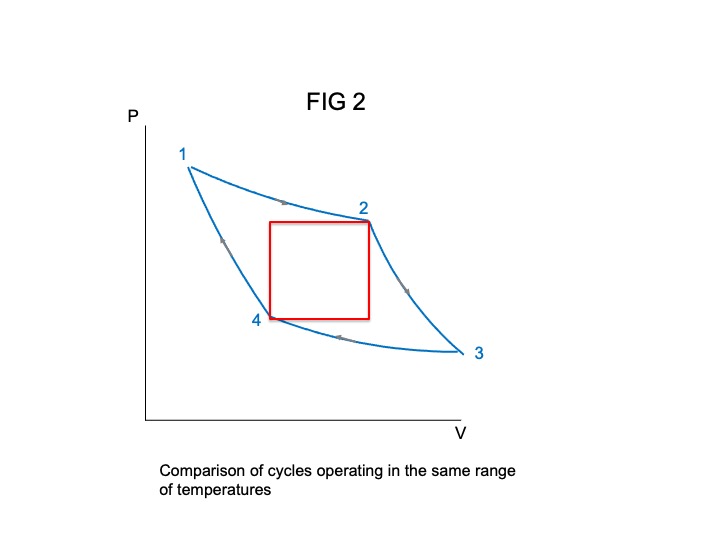
In carnot engine we care about maximising the efficiency, i.e how much useful work we get out the given heat. Now what is interesting to note is that any reversible engine working between two temperatures has the same efficiency as that of a carnot cycle. Why is that so?
So first things first, what is the efficiency of a carnot cycle.
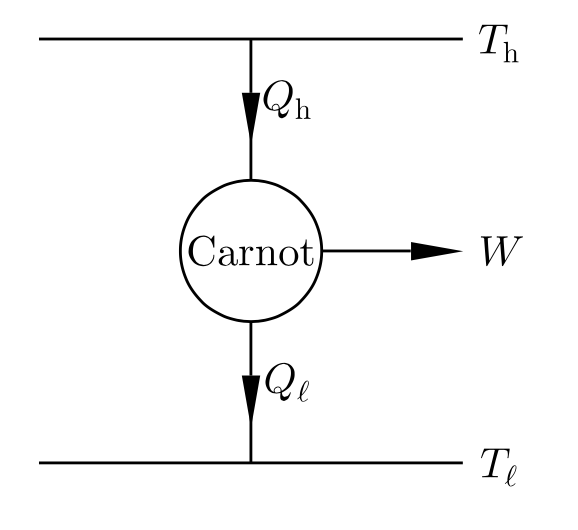
As you can see in the image the efficiency would be:
$$\eta_{carnot}=\frac{Q_h-Q_l}{Q_h}=1-\frac{T_l}{T_h}$$
Now, it is interesting that any real engine (irreversible) cannot be more efficient than a carnot engine.
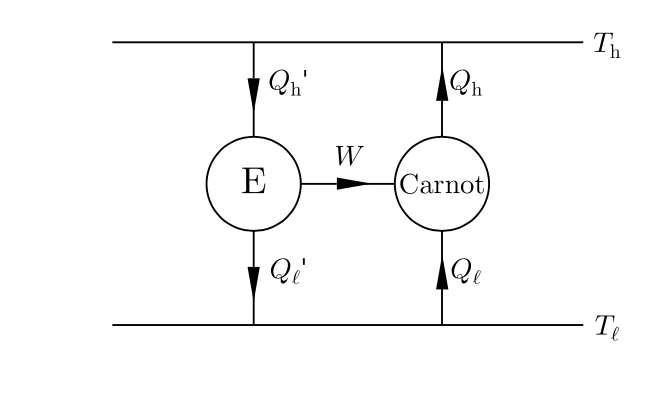
In the figure, say E is an engine that is more efficient than a Carnot engine, since $\eta_E> \eta_{\text{carnot}}$ we can write:
$$\frac{W}{Q_h'}>\frac{W}{Q_h}$$
Using the first law of thermodynamics we have:
$$W=Q'_h-Q'_l=Q_h-Q_l \implies Q'_h-Q_h=Q'_l-Q_l$$
$Q'_h-Q_h$ represents the amount of heat dumped into the reservoir at $T_h$ and $Q'_l-Q_l$ represents the amount of heat extracted from the reservoir at $T_l$. Because both are positive that means all the system is doing is taking heat from one reservoir and dumping all of it in another, violating the second Clausius statement.
This is the Carnot's theorem.
Now, how do we prove that any reversible engine has the same efficiency?
Say we connected an engine R, with efficiency $\eta_R \leq \eta_{carnot}$ from carnot theorem. If we run the whole system but in reverse it will simply transfer heat from hot to cold reservoir, unless the efficiencies of both the engines are the same.
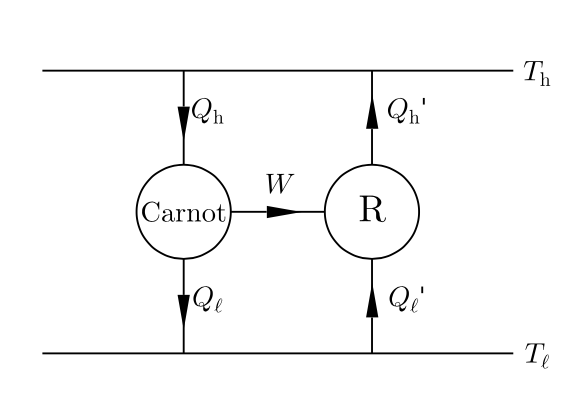
So, summing it up, we care about the efficiency not the amount of work per unit volume. Any reversible engine working between two temperatures have the same efficiency. And a real engine in general cannot be more efficient than a Carnot engine.
For further reading, read concepts in thermal physics by Blundell.
