The free-body-diagram of a falling ball where air resistance is not negligible, is the drag force ever greater than the weight of the ball? I mean mustn't it as it is slowing down? But then how is the terminal velocity obtained? Does it mean then that the drag force decreases, so increases first, then decreases?
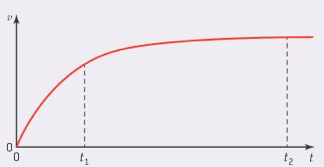
At least in my textbook they draw that the drag force on a falling ball is greater than that of its weight, how does this make sense? Perhaps let's look at the graph below, at t1, is the drag force greater than at t2? And I mean the gravitational force will always be on it the same from the instant it is released, right?