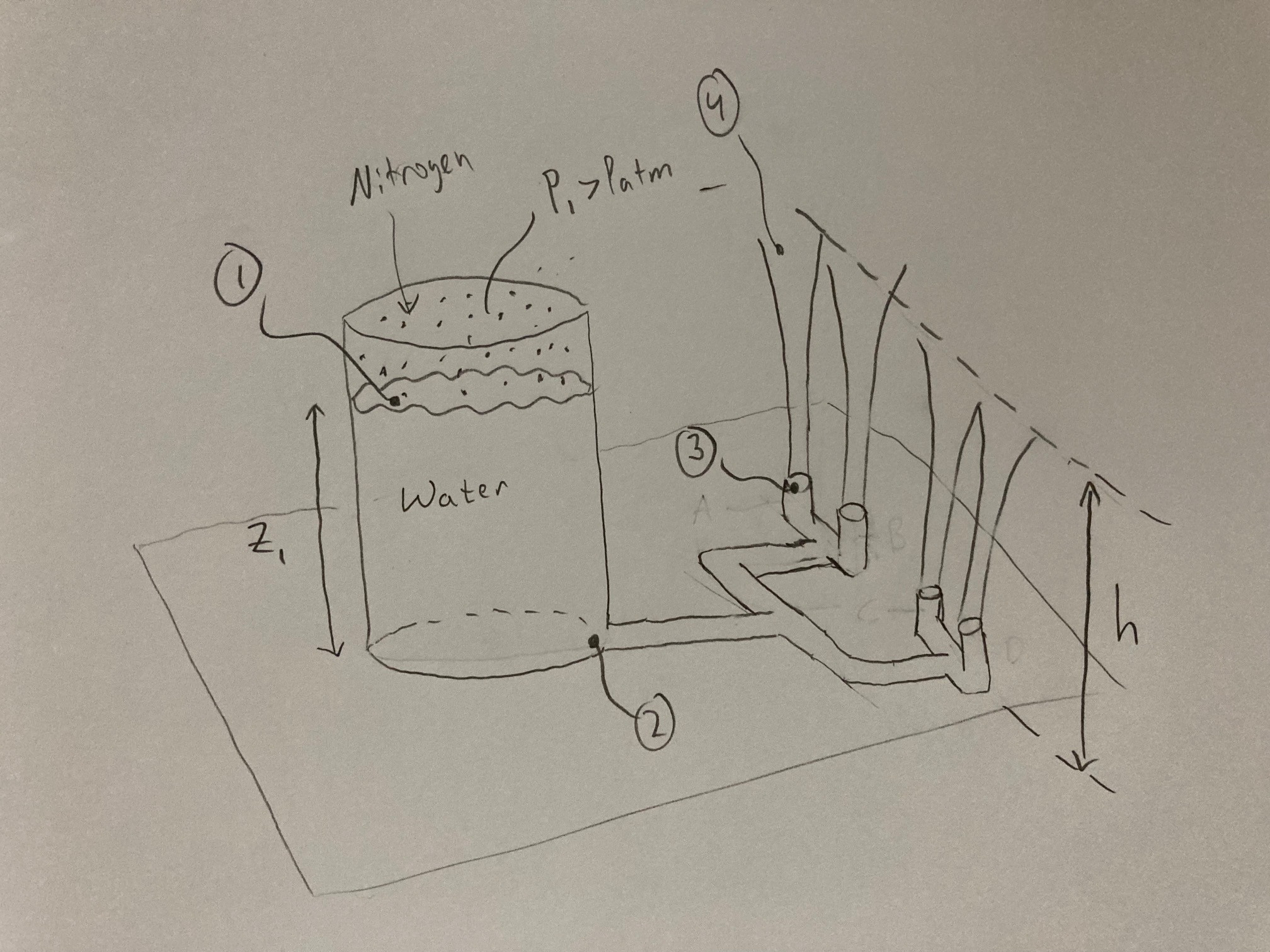
TL;DR With no head losses, when $A_2 > A_3$ then the flow rate through point 2 will be greater in case 2 (with four pipes on the output) than in case 1. Since we do not have any information about the head losses, I show below how to compare flow rates in idealized situation. Note that $A_3$ indicates total cross-section of all four pipes at the point 3 in case 2.
Or does continuity apply instead
The continuity equation applies when mass of a moving fluid does not change as it flows, which is the case in your example: volume (mass) that goes out of four pipes combined equals volume (mass) decrease in the tank
$$A_1 v_1 = A_2 v_2 = A_3 v_3$$
where $q$ is the volume flow rate, $A$ is the cross-section area, and $v$ is the fluid velocity. Note that here $A_3$ means combined cross-section of all four pipes. By combining continuity equation and idealized Bernoulli's equation
$$p + \rho g y + \frac{1}{2} \rho v^2 = \text{const.}$$
it is straightforward to show that ratio of velocities for the two cases is
$$\frac{v_2'}{v_2} = \sqrt{\frac{(A_1/A_2)^2 - 1}{(A_1/A_3)^2 - 1}} \qquad \text{and} \qquad \frac{v_3'}{v_2} = \sqrt{\frac{1-(A_2/A_1)^2}{1-(A_3/A_1)^2}}$$
where $v_2'$ and $v_3'$ are velocities at point 2 and 3 in case 2, and $v_2$ is velocity at point 2 in case 1.
Would the nozzle velocity equation stay the same (albeit with a different head loss)?
With no head losses and assuming $A_1 > A_2$ and $A_1 > A_3$, it is clear now that $v_2' > v_2$ and $v_3' > v_3$ when $A_3 > A_2$ and vice-versa.
This can also be solved intuitively:
When $A_3 = A_2$, where $A_3$ is cross-section of four nozzles combined, then point 1 sees the same cross-section to the output at atmospheric pressure. Hence, output velocity remains the same through points 2 and 3 in case 2 compared to point 2 in case 1.
As $A_3$ is getting smaller starting at $A_3 = A_2$, the point 1 sees smaller output cross-section and velocity through point 3 decreases. In the most extreme case, when $A_3 = 0$ the velocity is zero. As velocity through point 3 decreases, so does velocity through point 2.
As $A_3$ is getting larger starting at $A_3 = A_2$, the point 1 sees larger output cross-section and velocity through point 3 increases. As velocity through point 3 increases, so does velocity through point 2.
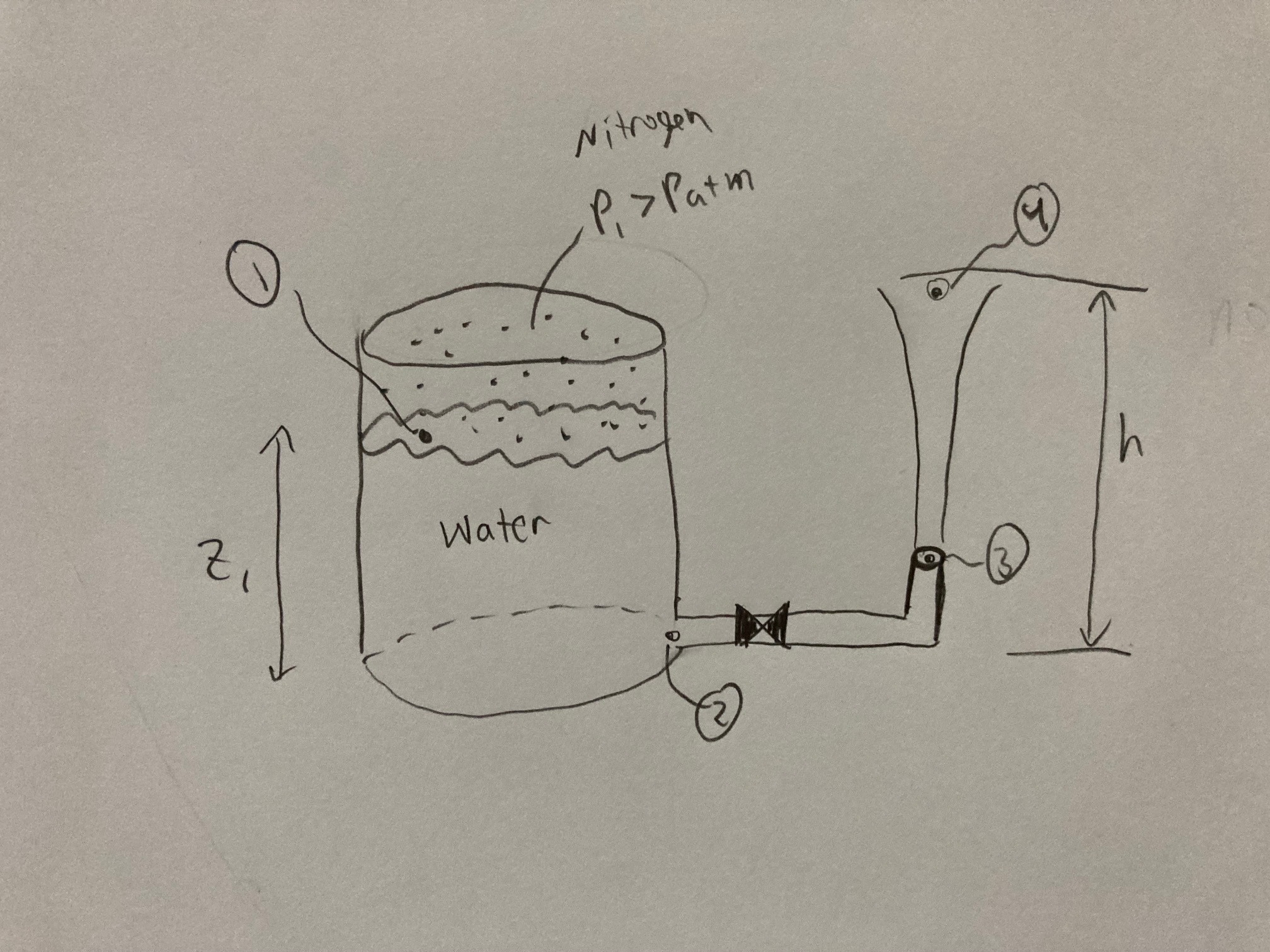This system pushes water through a nozzle. I can use Modified Bernoulli's equation to calculate the nozzle velocity as such:
$$V_3 = \sqrt{2g\left(\frac{P_1}{\rho g}+ z_1-\Delta h_L\right)}$$
