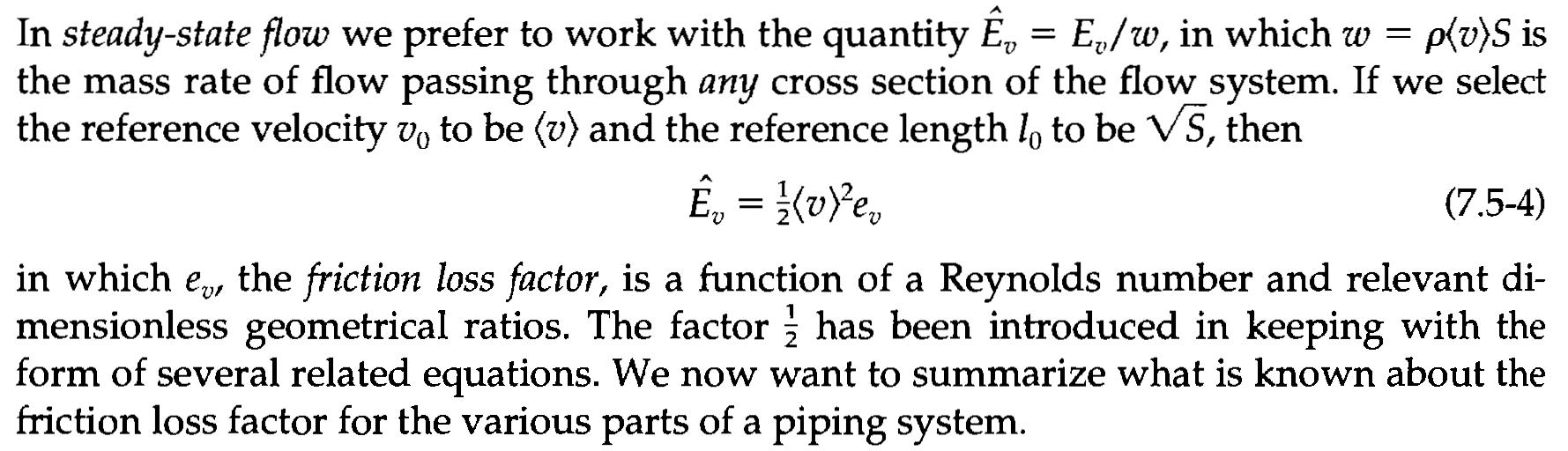
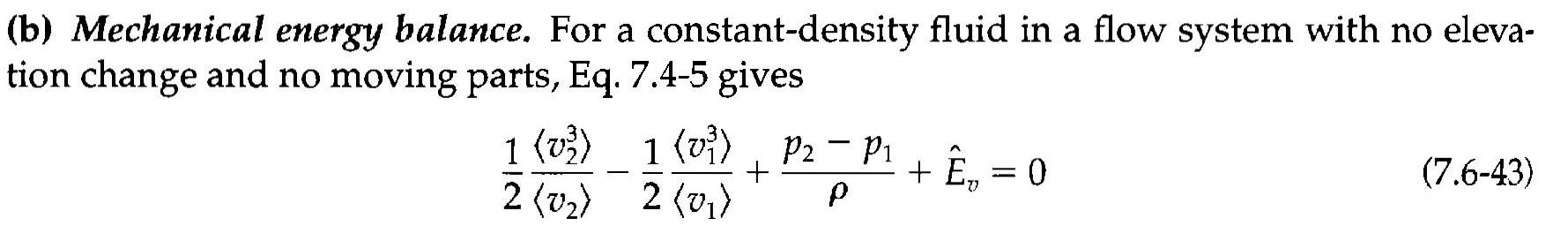1
$\begingroup$

I thought that Bernoulli equation could be used only in the case of non viscous fluid. But doing exercises on 2500 Solved Problems In Fluid Mechanics and Hydraulics (Schaum's Solved Problems Series) I found that this procedure is followed.

In the case of viscous laminar flow, Bernoulli equation is written as

$$z_1+\frac{v_1^2}{2 g}+\frac{p_1}{\rho g}=z_2+\frac{v_2^2}{2 g}+\frac{p_2}{\rho g}+h_L\tag{1}$$

Where $h_L$ is the head loss (due to viscosity) calculated using Hagen Poiseuille law.

$$h_L=\rho g \frac{8 \eta L \bar{v}}{R^2}\tag{2}$$

Is this a correct way to solve exercises involving viscosity?

Furthermore are there limitation to this use of Bernoulli equation (in case of viscosity)?

In particular

  • If the flow is not laminar, I cannot use $(2)$, but can I still write $(1)$ in that way?
  • Is $(1)$ valid only along the singular streamline or between different ones (assuming the fluid irrotational)?
Improve this question
$\endgroup$

2 Answers 2

Reset to default
2
$\begingroup$

This is a correct way to solve exercises involving viscosity (within certain constraints, e.g., constant viscosity). Eqn. 1 is a version of the Bernoulli equation, modified to include a frictional head loss, and is definitely valid, provided the velocities used are the average velocities. Eqn. 1 without the $h_L$ is valid along a streamline, even for a viscous flow. If Eqn. 1 is being used for a laminar viscous flow (say in a tube of slowly varying cross section), the kinetic energy terms should not have a 2 in the denominator. See Bird, Stewart, and Lightfoot, Transport Phenomena for details. Here they show that, if the 2 is to be included, then instead of using the average value of v squared, one should use the average value of $v^3$ divided by the average value of v. For laminar flow in a tube, this reduces to twice the square of the average velocity, so, if the average velocity squared is being used in the kinetic energy term, the 2 should not be included in the denominator if the flow is laminar.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thanks for the reply! Probably missing something but, in the case of laminar flow, since the velocity in the center of the tube is $v_{center}=2 \bar{v}$ - with $\bar{v}$ the average velocity - then $v_{center}^2=4 \bar{v}^2$ so $\frac{v^2}{2g}=\frac{2\bar{v}^2}{g}$. I get a factor $2$ in the numerator, could it be right? $\endgroup$
    – Sørën
    Commented Jun 23, 2016 at 11:17
  • 1
    $\begingroup$ No. The correct KE expression given in BSL is $\frac{1}{2}\frac{<v^3>}{<v>}$, where <> represents the average over the cross sectional area of the flow channel. For turbulent flow in a circular tube (which features close to a flat velocity profile), this reduces to $\frac{\bar{v}^2}{2}$. For laminar flow, this reduces to $\bar{v}^2$. $\endgroup$
    – Chet Miller
    Commented Jun 23, 2016 at 12:35
0
$\begingroup$

The reference given by Chet Miller states that: Mechanical energy balance equation

where the viscous term for your problem is defined as: Viscous term

I would propose that the equation given by the questions originator is correct (edit: nope), unless we are misunderstanding how to raise the velocity to the third power (edit: this is nt the source of the above confusion), as described by @Chet Miller.

Edit: there are several misunderstandings in the maths here and in the channel geometry. For a wide flat channel with fully developed laminar flow, the kinetic correction factor is 1.543.

See the full working and sources here:https://physics.stackexchange.com/a/823440/340947

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.